Waves and Vibrations

A wave is an oscillation that travels through space, accompanied by a transfer of energy.

Hooke's law of elasticity is an approximation that states that the extension of a spring is directly proportional to the load applied to it.

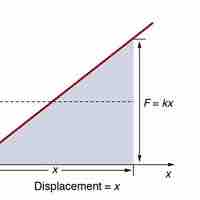
If a force results in only deformation, with no thermal, sound, or kinetic energy, the work done is stored as elastic potential energy.

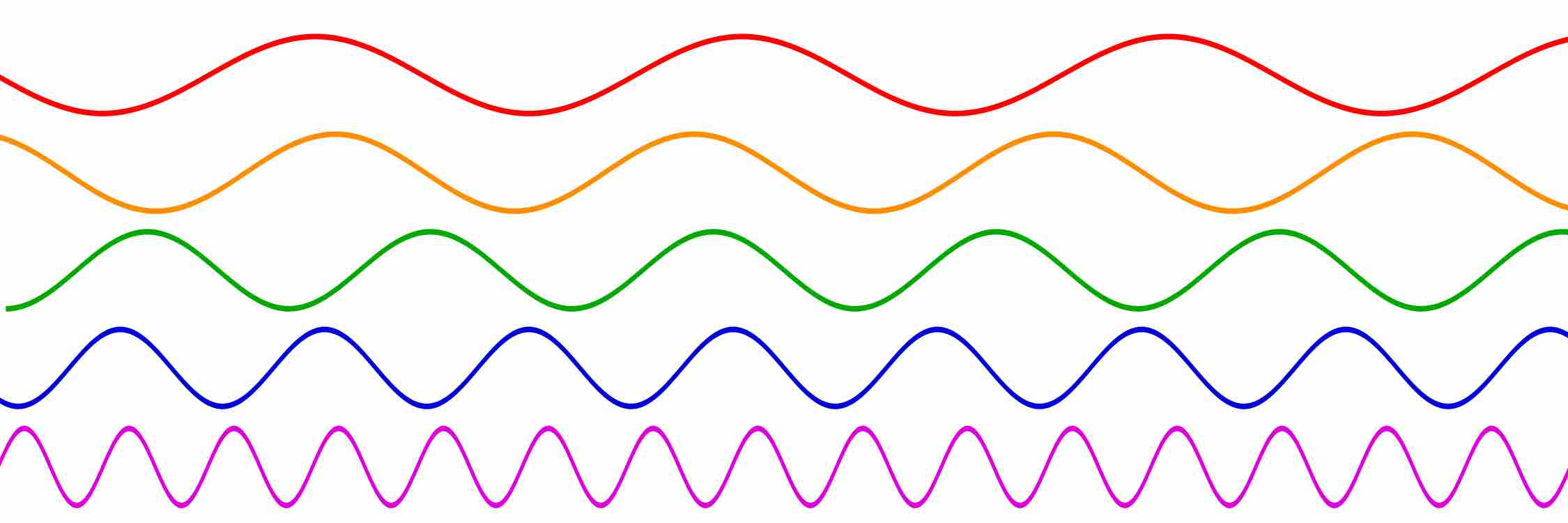
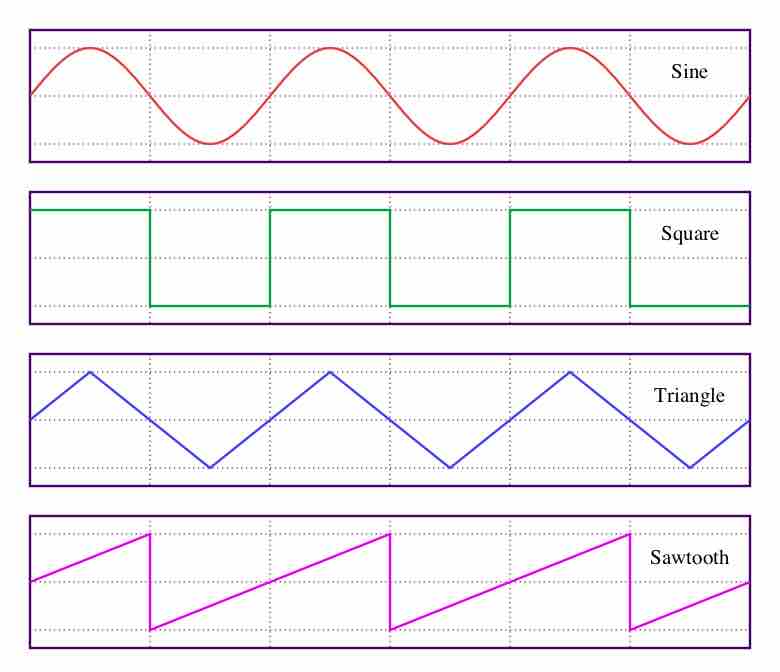
The period is the duration of one cycle in a repeating event, while the frequency is the number of cycles per unit time.

The period of a mass m on a spring of spring constant k can be calculated as $T=2\pi \sqrt{\frac{m}{k}}$.

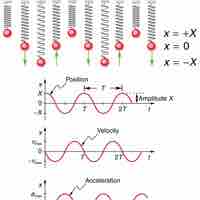
Simple harmonic motion is a type of periodic motion where the restoring force is directly proportional to the displacement.

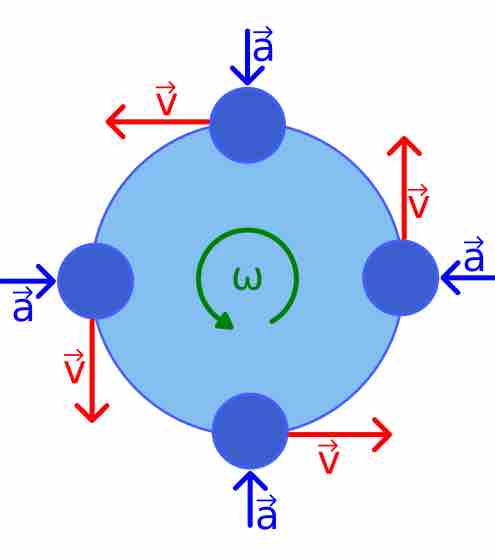
Simple harmonic motion is produced by the projection of uniform circular motion onto one of the axes in the x-y plane.

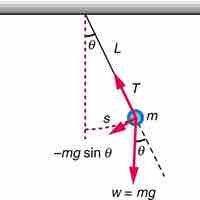
A simple pendulum acts like a harmonic oscillator with a period dependent only on L and g for sufficiently small amplitudes.

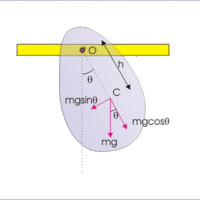
The period of a physical pendulum depends upon its moment of inertia about its pivot point and the distance from its center of mass.

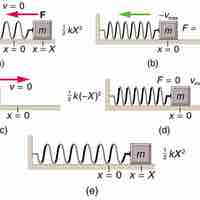
The total energy in a simple harmonic oscillator is the constant sum of the potential and kinetic energies.

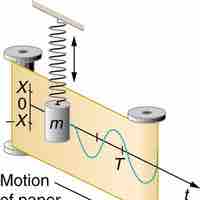
The solutions to the equations of motion of simple harmonic oscillators are always sinusoidal, i.e., sines and cosines.

Over time, the damped harmonic oscillator's motion will be reduced to a stop.

Driven harmonic oscillators are damped oscillators further affected by an externally applied force.

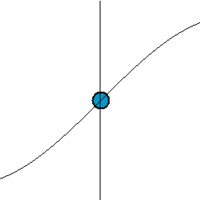
Wave motion transfers energy from one point to another, usually without permanent displacement of the particles of the medium.

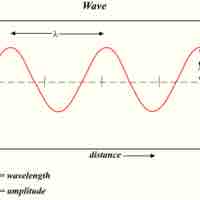
Transverse waves propagate through media with a speed $\vec{v}_w$ orthogonally to the direction of energy transfer.

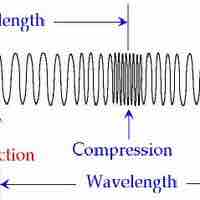
Longitudinal waves, sometimes called compression waves, oscillate in the direction of propagation.

Water waves can be commonly observed in daily life, and comprise both transverse and longitudinal wave motion.

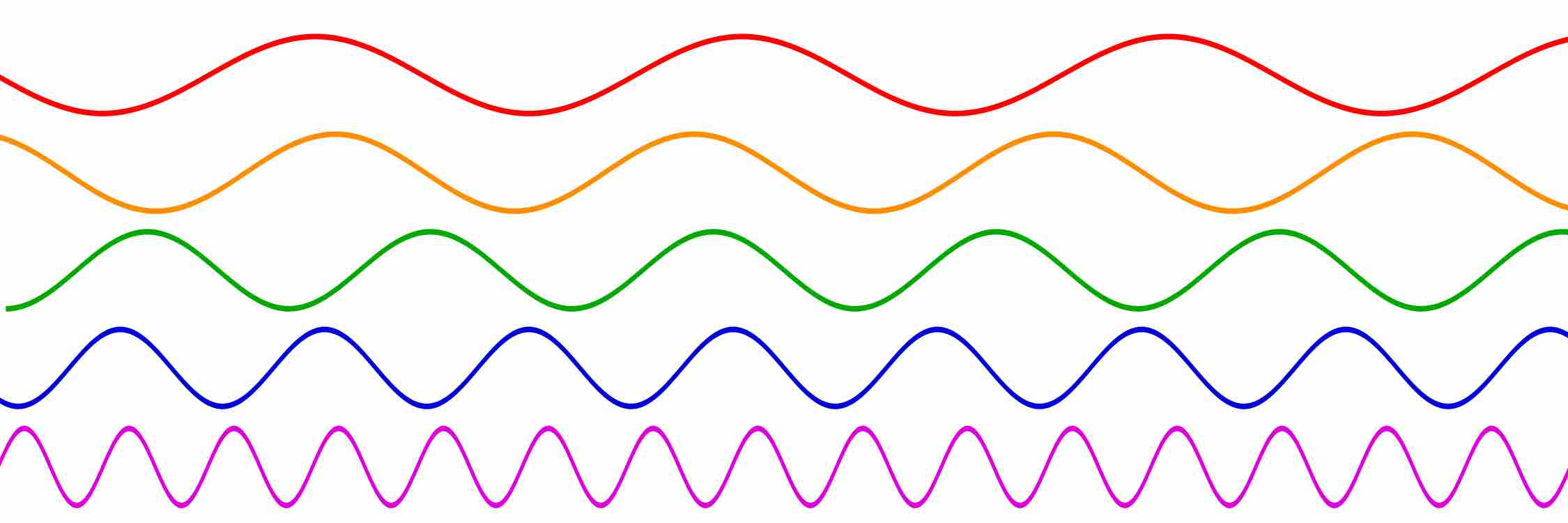
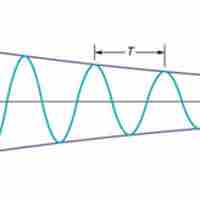
Waves are defined by its frequency, wavelength, and amplitude among others. They also have two kinds of velocity: phase and group velocity.

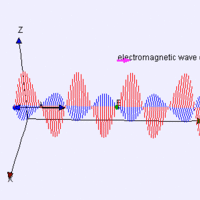
Waves transfer energy which can be used to do work.

When the medium changes, a wave often experiences partial transmission and partial refection at the interface.

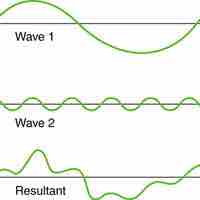
A wave may have a complicated shape that can result from superposition and interference of several waves.

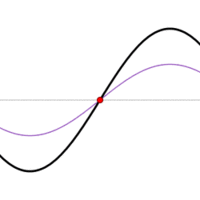
A standing wave is one in which two waves superimpose to produce a wave that varies in amplitude but does not propagate.

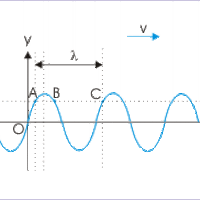
When vibrations in the string are simple harmonic motion, waves are described by harmonic wave functions.

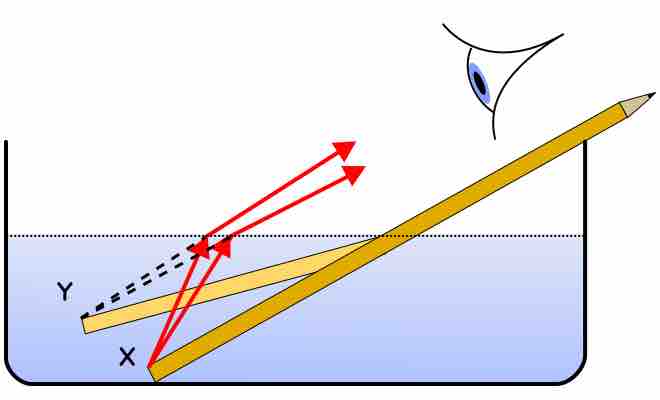
Refraction is a surface phenomenon that occurs as the change in direction of a wave due to a change in its medium.

Diffraction refers to various phenomena such as the bending of waves around obstacles and the spreading out of waves past small openings.

The most general solution of the wave equation $\frac{\partial^2 u}{\partial t^2} = c^2 \frac{\partial^2 u}{\partial x^2}$ is given as $u(x,t) = f(x+ct)+g(x-ct)$, where f and g are arbitrary functions.

The energy in a wave is proportional to its amplitude squared and the intensity of a wave is defined as power per unit area.

The speed of a wave on a string can be found by multiplying the wavelength by the frequency or by dividing the wavelength by the period.

When transverse waves in strings meet one end, they are reflected, and when the incident wave meets the reflected wave, interference occurs.