In general, one dimensional waves satisfy the 1D wave equation:
For example, a sinusoidal form
is a solution of the wave equation for
Solving the Wave Equation
First, we notice that any function u(x,t) satisfying
is a solution to the wave equation. To show this, note that
In the middle, we used the equation 1 along with the fact that partial derivatives are interchangeable.
To solve the equation 1, let's introduce new variables:
With the change of variables, the equation 1 becomes
where f and g are arbitrary functions. Converting back to the original variables of x and t, we conclude that the solution of the original wave equation is
f(x+ct) represents a left-going traveling wave, while g(x-ct) represents a right-going traveling wave. In other words, solutions of the 1D wave equation are sums of a left traveling function f and a right traveling function g. "Traveling" means that the shape of these individual arbitrary functions with respect to x stays constant, however the functions are translated left and right with time at the speed c. This solution was derived by Jean le Rond d'Alembert.
Boundary Condition
Any function that contains "x+ct" or "x-ct" can be a solution of the wave equation. The wave function is further determined by taking additional information, usually given as boundary conditions and some others. For example, in the case of a string in a guitar, we know that the wave has zero amplitude at both ends: u(x=0)=u(x=L)=0 . Also, the shape of the function at an instance can be provided to determine the function.
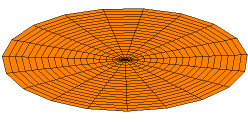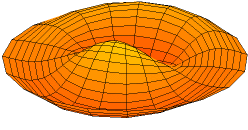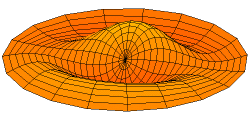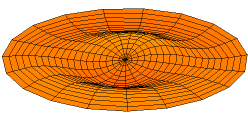
Wave Equation in Two Dimensions
A solution of the wave equation in two dimensions with a zero-displacement boundary condition along the entire outer edge.