Simple Harmonic Motion and Uniform Circular Motion

Uniform Circular Motion

Uniform circular motion describes the motion of a body traversing a circular path at constant speed. The distance of the body from the center of the circle remains constant at all times. Though the body's speed is constant, its velocity is not constant: velocity (a vector quantity) depends on both the body's speed and its direction of travel. Since the body is constantly changing direction as it travels around the circle, the velocity is changing also. This varying velocity indicates the presence of an acceleration called the centripetal acceleration. Centripetal acceleration is of constant magnitude and directed at all times towards the center of the circle. This acceleration is, in turn, produced by a centripetal force—a force in constant magnitude, and directed towards the center.

Velocity

The above figure illustrates velocity and acceleration vectors for uniform motion at four different points in the orbit. Since velocity v is tangent to the circular path, no two velocities point in the same direction. Although the object has a constant speed, its direction is always changing. This change in velocity is due to an acceleration, a, whose magnitude is (like that of the velocity) held constant, but whose direction also is always changing. The acceleration points radially inwards (centripetally) and is perpendicular to the velocity. This acceleration is known as centripetal acceleration.

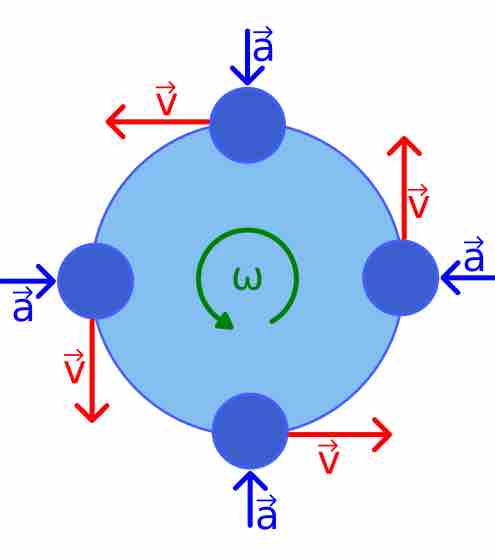
Uniform Circular Motion (at Four Different Point in the Orbit)

Velocity v and acceleration a in uniform circular motion at angular rate ω; the speed is constant, but the velocity is always tangent to the orbit; the acceleration has constant magnitude, but always points toward the center of rotation

Displacement around a circular path is often given in terms of an angle θ. This angle is the angle between a straight line drawn from the center of the circle to the objects starting position on the edge and a straight line drawn from the objects ending position on the edge to center of the circle. See for a visual representation of the angle where the point p started on the x-axis and moved to its present position. The angle θ describes how far it moved.

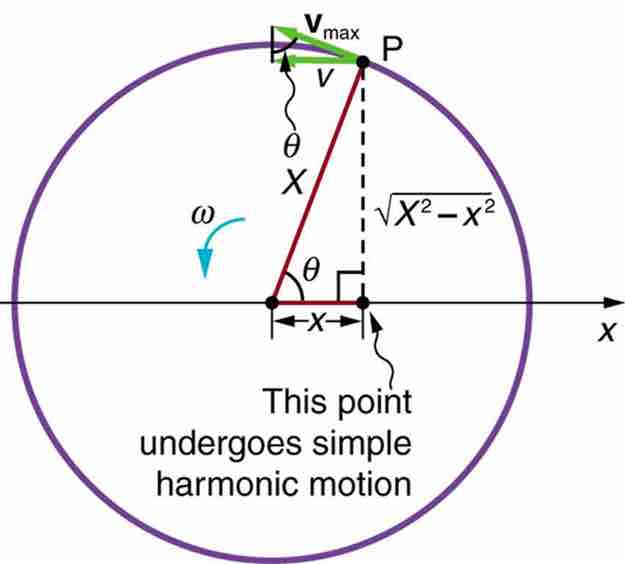
Projection of Uniform Circular Motion

A point P moving on a circular path with a constant angular velocity ω is undergoing uniform circular motion. Its projection on the x-axis undergoes simple harmonic motion. Also shown is the velocity of this point around the circle, v−max, and its projection, which is v. Note that these velocities form a similar triangle to the displacement triangle.

For a path around a circle of radius r, when an angle θ (measured in radians) is swept out, the distance traveled on the edge of the circle is s = rθ. You can prove this yourself by remembering that the circumference of a circle is 2*pi*r, so if the object traveled around the whole circle (one circumference) it will have gone through an angle of 2pi radians and traveled a distance of 2pi*r. Therefore, the speed of travel around the orbit is:

$v=r\frac{d\theta }{dt}=r\omega$,

where the angular rate of rotation is ω. (Note that ω = v/r. ) Thus, v is a constant, and the velocity vector v also rotates with constant magnitude v, at the same angular rate ω.

Acceleration

The acceleration in uniform circular motion is always directed inward and is given by:

$a=v\frac{d\theta }{dt}=v\omega =\frac{v^{2}}{r}$.

This acceleration acts to change the direction of v, but not the speed.

Simple Harmonic Motion from Uniform Circular Motion

There is an easy way to produce simple harmonic motion by using uniform circular motion. The figure below demonstrates one way of using this method. A ball is attached to a uniformly rotating vertical turntable, and its shadow is projected onto the floor as shown. The shadow undergoes simple harmonic motion .

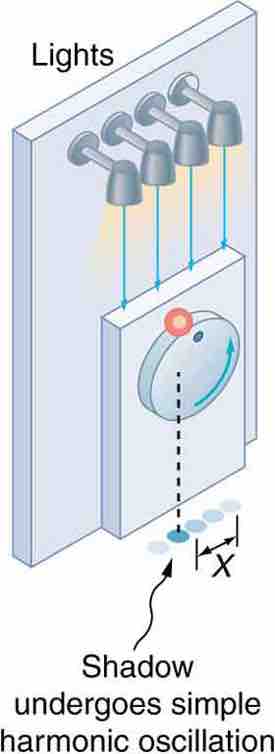
Shadow of a Ball Undergoing Simple Harmonic Motion

The shadow of a ball rotating at constant angular velocity ω on a turntable goes back and forth in precise simple harmonic motion.

The next figure shows the basic relationship between uniform circular motion and simple harmonic motion. The point P travels around the circle at constant angular velocity ω. The point P is analogous to the ball on a turntable in the figure above. The projection of the position of P onto a fixed axis undergoes simple harmonic motion and is analogous to the shadow of the object. At a point in time assumed in the figure, the projection has position x and moves to the left with velocity v. The velocity of the point P around the circle equals |v_max|. The projection of |v_max| on the x-axis is the velocity v of the simple harmonic motion along the x-axis .

To see that the projection undergoes simple harmonic motion, note that its position x is given by:

$x=Xcos\theta$,

where θ=ωt, ω is the constant angular velocity, and X is the radius of the circular path. Thus,

$x=Xcos\omega t$.

The angular velocity ω is in radians per unit time; in this case 2π radians is the time for one revolution T. That is, ω=2π/T. Substituting this expression for ω, we see that the position x is given by:

$x(t)=cos(\frac{2\pi t}{T})=cos(2\pi ft)$.

Note: This equation should look familiar from our earlier discussion of simple harmonic motion.