We know from the second law of thermodynamics that a heat engine cannot be 100 percent efficient, since there must always be some heat transfer Qc to the environment. (See our atom on "Heat Engines. ") How efficient can a heat engine be then? This question was answered at a theoretical level in 1824 by a young French engineer, Sadi Carnot (1796-1832), in his study of the then-emerging heat engine technology crucial to the Industrial Revolution. He devised a theoretical cycle, now called the Carnot cycle, which is the most efficient cyclical process possible. The second law of thermodynamics can be restated in terms of the Carnot cycle, and so what Carnot actually discovered was this fundamental law. Any heat engine employing the Carnot cycle is called a Carnot engine.
What is crucial to the Carnot cycle is that only reversible processes are used. Irreversible processes involve dissipative factors, such as friction and turbulence. This increases heat transfer Qc to the environment and reduces the efficiency of the engine. Obviously, then, reversible processes are superior.
The second law of thermodynamics (a third form): A Carnot engine operating between two given temperatures has the greatest possible efficiency of any heat engine operating between these two temperatures. Furthermore, all engines employing only reversible processes have this same maximum efficiency when operating between the same given temperatures.
Efficiency
The Carnot cycle comprises two isothermal and two adiabatic processes. Recall that both isothermal and adiabatic processes are, in principle, reversible .
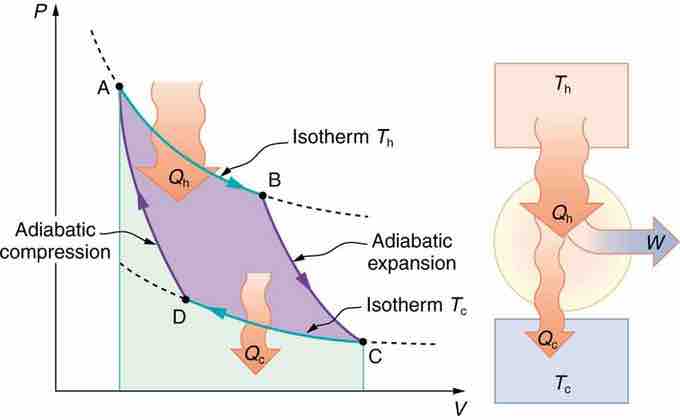
PV Diagram for a Carnot Cycle
PV diagram for a Carnot cycle, employing only reversible isothermal and adiabatic processes. Heat transfer Qh occurs into the working substance during the isothermal path AB, which takes place at constant temperature Th. Heat transfer Qc occurs out of the working substance during the isothermal path CD, which takes place at constant temperature Tc. The net work output W equals the area inside the path ABCDA. Also shown is a schematic of a Carnot engine operating between hot and cold reservoirs at temperatures Th and Tc.
Carnot also determined the efficiency of a perfect heat engine—that is, a Carnot engine. It is always true that the efficiency of a cyclical heat engine is given by:
What Carnot found was that for a perfect heat engine, the ratio Qc/Qh equals the ratio of the absolute temperatures of the heat reservoirs. That is, Qc/Qh=Tc/Th for a Carnot engine, so that the maximum or Carnot efficiency EffC is given by