What is Entropy?

In this and following Atoms, we will study entropy. By examining it, we shall see that the directions associated with the second law—heat transfer from hot to cold, for example—are related to the tendency in nature for systems to become disordered and for less energy to be available for use as work. The entropy of a system can in fact be shown to be a measure of its disorder and of the unavailability of energy to do work.

Definition of Entropy

We can see how entropy is defined by recalling our discussion of the Carnot engine. We noted that for a Carnot cycle, and hence for any reversible processes, Q_c/Q_h=T_c/T_h. Rearranging terms yields $\frac{Q_c}{T_c} = \frac{Q_h}{T_h}$ for any reversible process. Q_c and Q_h are absolute values of the heat transfer at temperatures T_c and T_h, respectively. This ratio of Q/T is defined to be the change in entropy ΔS for a reversible process,

$\Delta S = (\frac{Q}{T})_{\textbf{rev}}$,

where Q is the heat transfer, which is positive for heat transfer into and negative for heat transfer out of, and T is the absolute temperature at which the reversible process takes place. The SI unit for entropy is joules per kelvin (J/K). If temperature changes during the process, then it is usually a good approximation (for small changes in temperature) to take T to be the average temperature, avoiding the need to use integral calculus to find ΔS.

The definition of ΔS is strictly valid only for reversible processes, such as used in a Carnot engine. However, we can find ΔS precisely even for real, irreversible processes. The reason is that the entropy S of a system, like internal energy U, depends only on the state of the system and not how it reached that condition. Entropy is a property of state. Thus the change in entropy ΔS of a system between state one and state two is the same no matter how the change occurs. We just need to find or imagine a reversible process that takes us from state one to state two and calculate ΔS for that process. That will be the change in entropy for any process going from state one to state two .

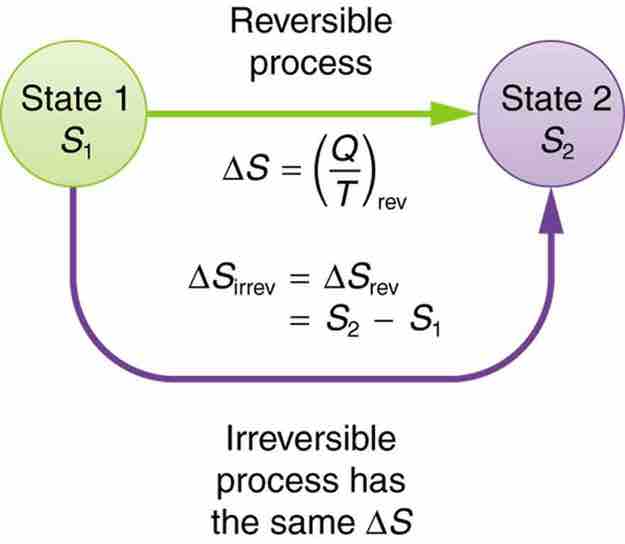
Change in Entropy

When a system goes from state one to state two, its entropy changes by the same amount ΔS, whether a hypothetical reversible path is followed or a real irreversible path is taken.

Example

Now let us take a look at the change in entropy of a Carnot engine and its heat reservoirs for one full cycle . The hot reservoir has a loss of entropy ΔSh=−Q_h/T_h, because heat transfer occurs out of it (remember that when heat transfers out, then Q has a negative sign). The cold reservoir has a gain of entropy ΔS_c=Q_c/T_c, because heat transfer occurs into it. (We assume the reservoirs are sufficiently large that their temperatures are constant. ) So the total change in entropy is

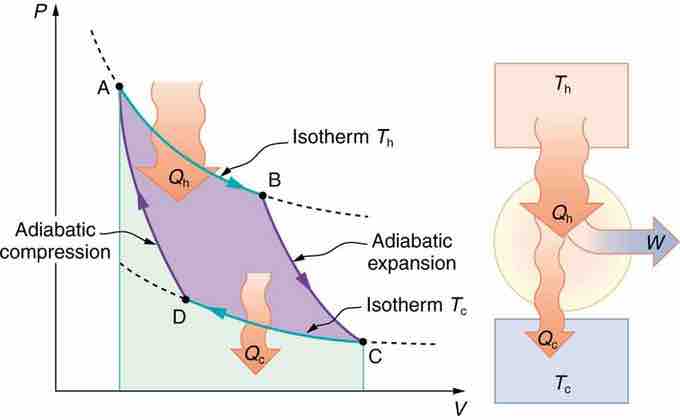
PV Diagram for a Carnot Cycle

PV diagram for a Carnot cycle, employing only reversible isothermal and adiabatic processes. Heat transfer Qh occurs into the working substance during the isothermal path AB, which takes place at constant temperature Th. Heat transfer Qc occurs out of the working substance during the isothermal path CD, which takes place at constant temperature Tc. The net work output W equals the area inside the path ABCDA. Also shown is a schematic of a Carnot engine operating between hot and cold reservoirs at temperatures Th and Tc.

$\Delta S_{tot} = \Delta S_h + \Delta S_c$.

Thus, since we know that Q_h/T_h=Q_c/T_c for a Carnot engine,

$\Delta S_{tot} = -\frac{Q_h}{T_h} + \frac{Q_c}{T_c} = 0$.

This result, which has general validity, means that the total change in entropy for a system in any reversible process is zero.