Chapter 15 The Money Supply and the Money Multiplier

15.1 A More Sophisticated Money Multiplier for M1

In Chapter 14 "The Money Supply Process", you learned that an increase (decrease) in the monetary base (MB, which = C + R) leads to an even greater increase (decrease) in the money supply (MS, such as M1M1 is a measure of the money supply that includes currency in circulation plus checkable deposits. or M2M2 is a measure of the money supply that includes M1 plus time deposits and noninstitutional (retail) money market funds.) due to the multiple deposit creation process. You also learned a simple but unrealistic upper-bound formula for estimating the change that assumed that banks hold no excess reserves and that the public holds no currency.

To get a more realistic estimate, we’ll have to do a little more work. We start with the observation that we can consider the money supply to be a function of the monetary base times some money multiplier (m):

This is basically a less specific version of the formula you learned in Chapter 14 "The Money Supply Process", except that instead of calculating the change in deposits (ΔD) brought about by the change in reserves (ΔR), we will now calculate the change in the money supply (ΔMS) brought about by the change in the monetary base (ΔMB). Furthermore, instead of using the reciprocal of the required reserve ratio (1/rr) as the multiplier, we will use a more sophisticated one (m₁, and later M₂) that doesn’t assume away cash and excess reserves.

We can add currency and excess reserves to the equation by algebraically describing their relationship to checkable depositsDeposits that can easily, cheaply, and quickly be drawn upon by check in order to make payments. Also known as transaction deposits. in the form of a ratio:

C/D = currency ratio

ER/D = excess reserves ratio

Recall that required reserves are equal to checkable deposits (D) times the required reserve ratio (rr). Total reserves equal required reserves plus excess reserves:

So we can render MB = C + R as MB = C + rrD + ER. Note that we have successfully removed C and ER from the multiple deposit expansion process by separating them from rrD. After further algebraic manipulations of the above equation and the reciprocal of the reserve ratio (1/rr) concept embedded in the simple deposit multiplier, we’re left with a more sophisticated, more realistic money multiplier:

So if

Required reserve ratio (rr) = .2

Currency in circulation = $100 billion

Deposits = $400 billion

Excess reserves = $10 billion

Practice calculating the money multiplier in Exercise 1.

Stop and Think Box

Explain Figure 15.1 "U.S. MB and M1, 1959–2007", Figure 15.2 "U.S. m", and Figure 15.3 "U.S. currency and checkable deposits, 1959–2007".

Figure 15.1 U.S. MB and M1, 1959–2007

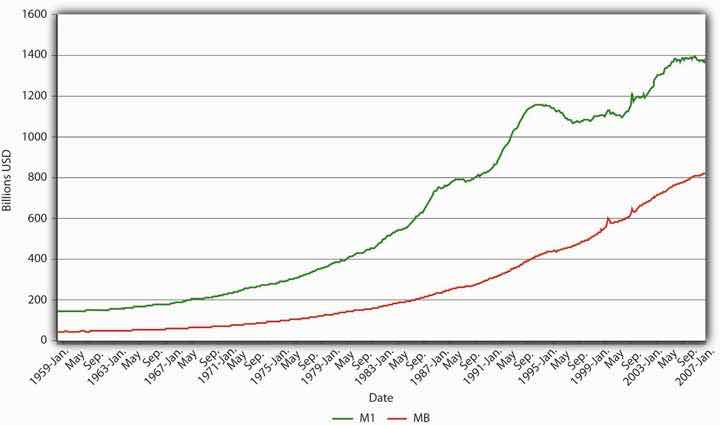
Figure 15.2 U.S. m₁, 1959–2007.

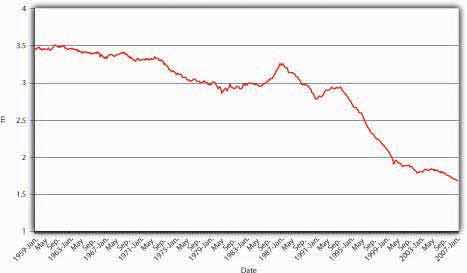
Figure 15.3 U.S. currency and checkable deposits, 1959–2007

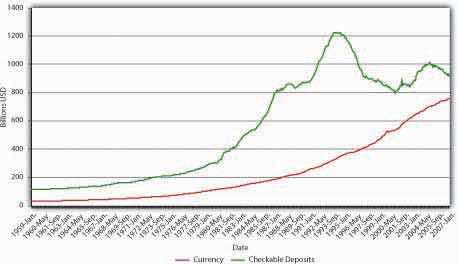
Figure 15.4 U.S. currency ratio, 1959–2007

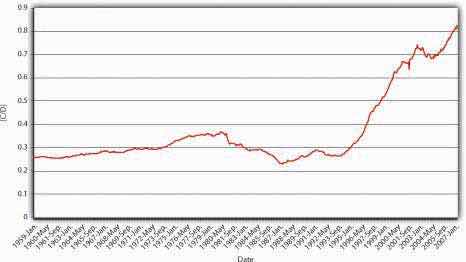
In Figure 15.1 "U.S. MB and M1, 1959–2007", M1 has increased because MB has increased, likely due to net open market purchases by the Fed. Apparently, m₁ has changed rather markedly since the early 1990s. In Figure 15.2 "U.S. m", the M1 money multiplier m has indeed dropped considerably since about 1995. That could be caused by an increase in rr, C/D, or ER/D. Figure 15.3 "U.S. currency and checkable deposits, 1959–2007" shows that m decreased primarily because C/D increased. It also shows that the increase in C/D was due largely to the stagnation in D coupled with the continued growth of C. The stagnation in D is likely due to the advent of sweep accounts. Figure 15.4 "U.S. currency ratio, 1959–2007" isolates C/D for closer study.

15.2 The M2 Money Multiplier

Note that m₁ is the M1 money multiplier. With a little bit more work, one can also calculate the M2 money multiplier (m₂). Recall from Chapter 3 "Money" that M2 = C + D + T + MMF, where T = time and savings deposits and MMF = money market funds, money market deposit accounts, and overnight loans. We account for the extra types of deposits in the same way as we accounted for currency and excess reserves, by expressing them as ratios against checkable deposits:

(T/D) = time deposit ratio

(MMF/D) = money market ratio

which leads to the following equation:

Once you calculate M2, multiply it by the change in MB to calculate the change in the MS, specifically in M2, just as you did in Exercise 2. Notice that the denominator of the m₂ equation is the same as the m₁ equation but that we have added the time and money market ratios to the numerator. So M2 is alwaysM2 would equal m₁ iff T = 0 and MMF = 0, which is highly unlikely. Note: iff means if and only if. > m₁, ceteris paribus, which makes sense when you recall that M2 is composed of M1 plus other forms of money. To verify this, recall that we calculated m₁ as 2.6316 when

Required reserves (rr) = .2

Currency in circulation = $100 million

Deposits = $400 million

Excess reserves = $10 million

We’ll now add time deposits of $900 million and money market funds of $800 million and calculate M2:

This is quite a bit higher than m₁ because time deposits and money market funds are not subject to reserve requirements, so they can expand more than checkable deposits because there is less drag on them during the multiple expansion process.

Practice calculating the M2 money multiplier on your own in the exercise.

15.3 Summary and Explanation

By way of summary, Figure 15.5 "Major influences on m" explains why each of the major variables influences m₁ and m₂ in the ways implied by the equations presented above.

Figure 15.5 Major influences on m₁ and m₂

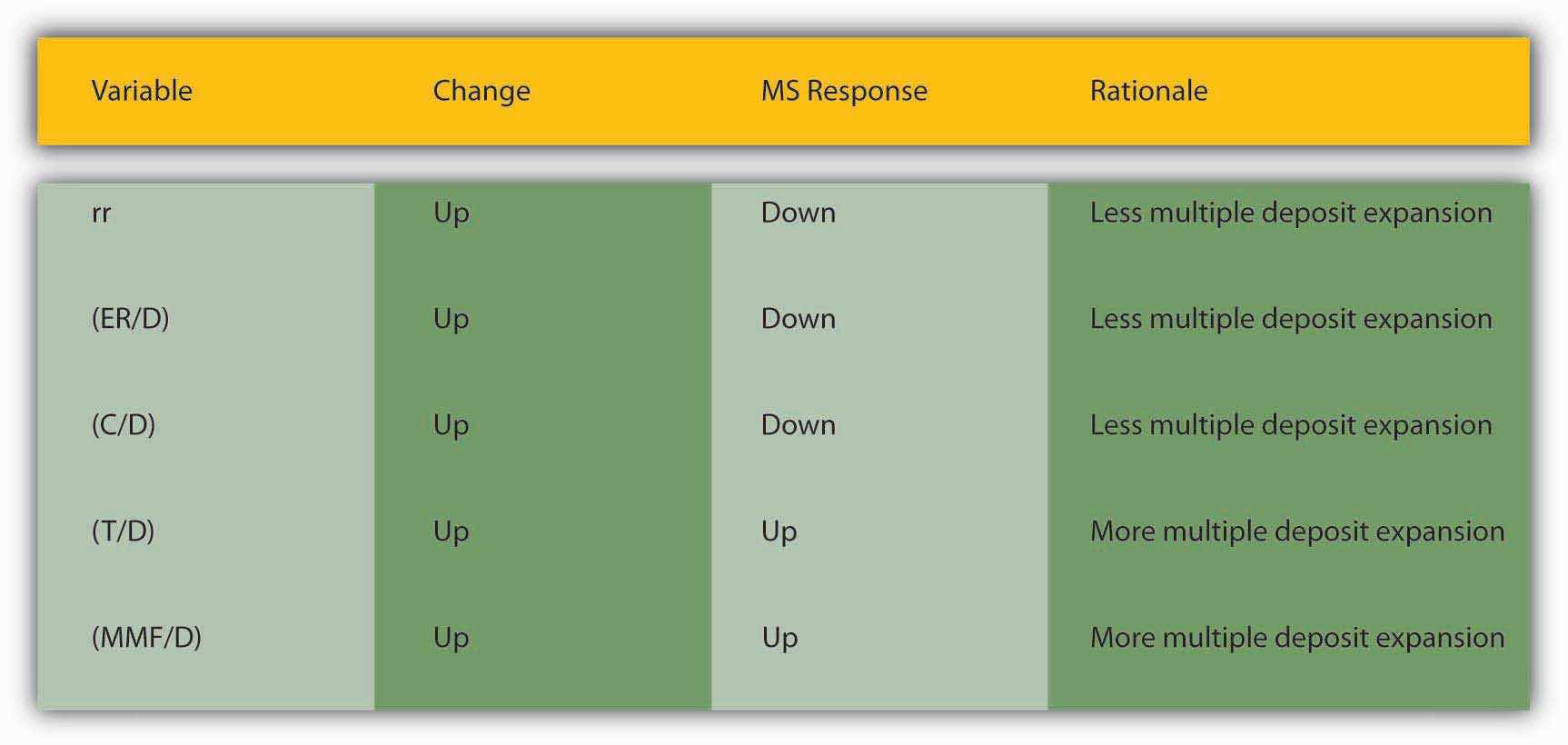
As we saw in Chapter 14 "The Money Supply Process", currency holdings, excess reserves, and required reserves slow down the multiple deposit creation process by removing funds from it. The bigger rr and ER/D are, the less each bank lends of the new deposits it receives. The bigger C/D is, the less money is deposited in the first place. For those reasons, we place those variables in the denominator. The larger the denominator, holding the numerator constant, the smaller m₁ or M2 will be, of course. The appropriate money supply components compose the numerator-currency, and checkable deposits for m₁, and currency, checkable deposits, time deposits, and money market mutual funds compose the numerator for M2.

This leaves us to consider why C/D, rr, and ER/D change over time. Short term, the currency ratio varies directly with the interest rate and the stability of the banking system. As the interest rate increases, the opportunity cost of keeping cash increases, so people are less anxious to hold it. People are also less anxious to hold currency if the banking system is stable because their money is safer in a checking deposit. If interest rates are extremely low or people believe the banks might be shaky, they naturally want to hold more physical cash. Longer term, C/D may be influenced by technology and loophole mining, encouraging bankers and depositors to eschew traditional checkable deposits in favor of sweep accounts.

The required reserve ratio is mandated by the central bank but, as noted in Chapter 10 "Innovation and Structure in Banking and Finance", loophole mining and technology have rendered it less important in recent years because sweep accounts allow banks to minimize the de jure level of their checkable deposits. In many places, rr is no longer a binding constraint on banks so, as we’ll see, most central banks no longer consider changing it as an effective monetary policy tool. (This in no way affects the money multiplier, which would provide the same figure for m₁ or M2 whether we calculate them as above or replace rr and ER/D with R/D, where R = total reserves.)

Excess reserves (or just reserves in a system without required reserves) are inversely related to the interest rate. In the early 1960s and early 2000s, when the interest rate was well less than 5 percent, ER/D was high, at .003 to .004. In the 1970s and 1980s, when interest rates were 10 percent and higher, ER/D dropped to .001 to .002. As we learned in Chapter 9 "Bank Management", expected deposit outflows directly affect excess reserve levels as banks stock up on reserves to meet the outflows. When uncertainty is high or a banking crisis is in progress or appears imminent, bankers will increase ER to protect their banks.

In summary, the central bank influences the money supply by controlling the monetary base and, to a far lesser extent, the required reserve ratio. Depositors, banks, and borrowers influence the money supply by influencing m₁ and M2, specifically by determining the money multiplier, with depositors largely in control of C/D; depositors and banks interacting via deposit outflow expectations to determine E/R; and borrowers, depositors, banks, and the central bank interacting to determine interest rates and hence to some extent both C/D and E/R.

15.4 Suggested Reading

Macesich, George. The Money Supply Process: A Comparative Analysis. New York: Praeger, 1999.