Estimating the Target Parameter: Interval Estimation

Interval estimation is the use of sample data to calculate an interval of possible (or probable) values of an unknown population parameter.

Learning Objective

Use sample data to calculate interval estimation

Key Points

The most prevalent forms of interval estimation are confidence intervals (a frequentist method) and credible intervals (a Bayesian method).
When estimating parameters of a population, we must verify that the sample is random, that data from the population have a Normal distribution with mean $\mu$ and standard deviation $\sigma$, and that individual observations are independent.
In order to specify a specific $t$-distribution, which is different for each sample size $n$, we use its degrees of freedom, which is denoted by $df$, and $df = n-1$.
If we wanted to calculate a confidence interval for the population mean, we would use: $\bar{x}\pm t^{*}\frac{s}{\sqrt{n}}$, where $t^*$ is the critical value for the $t(n-1)$ distribution.

Terms

t-distribution
a family of continuous probability disrtibutions that arises when estimating the mean of a normally distributed population in situations where the sample size is small and population standard devition is unknown
critical value
the value corresponding to a given significance level

Full Text

Interval estimation is the use of sample data to calculate an interval of possible (or probable) values of an unknown population parameter. The most prevalent forms of interval estimation are:

confidence intervals (a frequentist method); and
credible intervals (a Bayesian method).

Other common approaches to interval estimation are:

Tolerance intervals
Prediction intervals - used mainly in Regression Analysis
Likelihood intervals

Example: Estimating the Population Mean

How can we construct a confidence interval for an unknown population mean $\mu$ when we don't know the population standard deviation $\sigma$? We need to estimate from the data in order to do this. We also need to verify three conditions about the data:

The data is from a simple random sample of size $n$ from the population of interest.
Data from the population have a Normal distribution with mean and standard deviation. These are both unknown parameters.
The method for calculating a confidence interval assumes that individual observations are independent.

The sample mean $\bar{x}$ has a Normal distribution with mean and standard deviation $\frac{\sigma }{\sqrt{n}}$. Since we don't know $\sigma$, we estimate it using the sample standard deviation $s$. So, we estimate the standard deviation of $\bar{x}$ using $\frac{s }{\sqrt{n}}$, which is called the standard error of the sample mean.

The $t$-Distribution

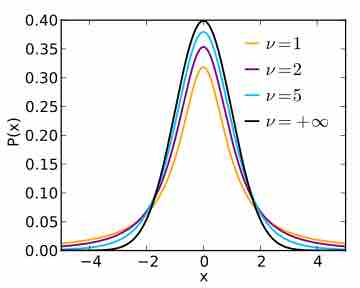
When we do not know $\frac{\sigma}{\sqrt{n}}$, we use $\frac{s }{\sqrt{n}}$. The distribution of the resulting statistic, $t$, is not Normal and fits the $t$-distribution. There is a different $t$-distribution for each sample size $n$. In order to specify a specific $t$-distribution, we use its degrees of freedom, which is denoted by $df$, and $df= n-1$.

$t$-Distribution

A plot of the $t$-distribution for several different degrees of freedom.

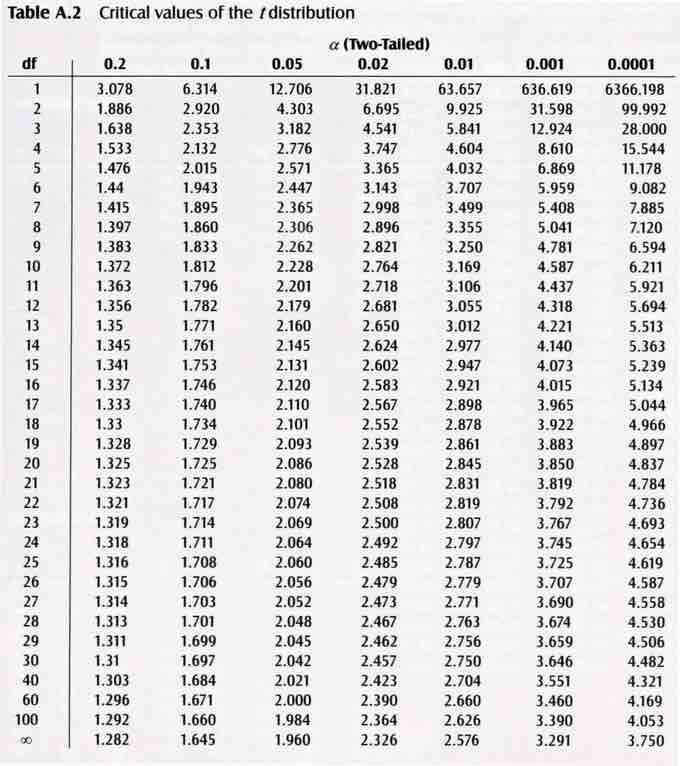
If we wanted to estimate the population mean, we can now put together everything we've learned. First, draw a simple random sample from a population with an unknown mean. A confidence interval for is calculated by: $\bar{x}\pm t^{*}\frac{s}{\sqrt{n}}$, where $t^*$ is the critical value for the $t(n-1)$ distribution.

$t$-Table

Critical values of the $t$-distribution.

[ edit ]

Prev Concept

Estimating the Target Parameter: Point Estimation

Estimating a Population Proportion

Next Concept