Triple Integrals

For $T \subseteq R^3$, the triple integral over $T$ is written as 

$\displaystyle{\iiint_T f(x,y,z)\, dx\, dy\, dz}$

Notice that, by convention, the triple integral has three integral signs (and a double integral has two integral signs); this is a notational convention which is convenient when computing a multiple integral as an iterated integral.

We have seen that double integrals can be evaluated over regions with a general shape. The extension of those formulae to triple integrals should be apparent. If $T$ is a domain that is normal with respect to the xy-plane and determined by the functions $\alpha (x,y)$ and $\beta(x,y)$, then: 

$\displaystyle{\iiint_T f(x,y,z) \ dx\, dy\, dz = \iint_D \int_{\alpha (x,y)}^{\beta (x,y)} f(x,y,z) \, dz dx dy}$

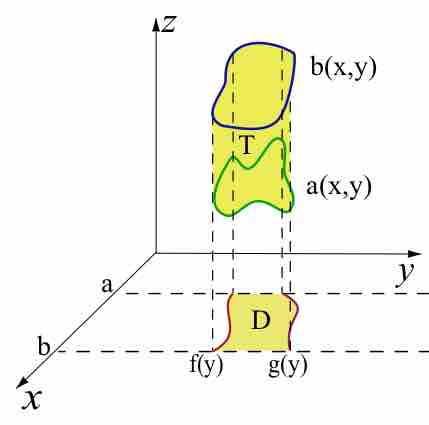
Graphical Representation of a Triple Integral

Example of domain in $R^3$ that is normal with respect to the $xy$-plane.

Example 1

The volume of the parallelepiped of sides 4 by 6 by 5 may be obtained in two ways:

• By calculating the double integral of the function $f(x, y) = 5$ over the region $D$ in the $xy$-plane which is the base of the parallelepiped: $\iint_D 5 \ dx\, dy$
• By calculating the triple integral of the constant function 1 over the parallelepiped itself: $\iiint_\mathrm{parallelepiped} 1 \, dx\, dy\, dz$

Example 2

Integrate $f(x,y,z) = x^2 + y^2 + z^2$ over the domain $D = \left \{ x^2 + y^2 + z^2 \le 16 \right \}$.

Looking at the domain, it seems convenient to adopt the passage in spherical coordinates; in fact, the intervals of the variables that delimit the new $T$ region are obviously: 

$(0 \le \rho \le 4, \ 0 \le \phi \le \pi, \ 0 \le \theta \le 2 \pi)$

For the function, we get:

$f(\rho \sin \phi \cos \theta, \rho \sin \phi \sin \theta, \rho \cos \phi) = \rho^2$

Therefore:

$\begin{aligned} \iiint_D (x^2 + y^2 +z^2) \, dx\, dy\, dz &= \iiint_T \rho^2 \ \rho^2 \sin \theta \, d\rho\, d\theta\, d\phi \\ &= \int_0^{\pi} \sin \phi \,d\phi \int_0^4 \rho^4 d \rho \int_0^{2 \pi} d\theta \\ &= 2 \pi \int_0^{\pi} \sin \phi \left[ \frac{\rho^5}{5} \right]_0^4 \, d \phi \\ &= 2 \pi \left[ \frac{\rho^5}{5} \right]_0^4 \left[- \cos \phi \right]_0^{\pi} = \frac{4096 \pi}{5} \end{aligned}$