Electric Field from a Point Charge

The electric field of a point charge is, like any electric field, a vector field that represents the effect that the point charge has on other charges around it. The effect is felt as a force, and when charged particles are not in motion, this force is known as the electrostatic force. The electrostatic force is, much like gravity, a force that acts at a distance. Therefore, we rationalize this action at a distance by saying that charges create fields around them that have effects on other charges.

Given a point charge, or a particle of infinitesimal size that contains a certain charge, electric field lines emanate radially in all directions. If the charge is positive, field lines point radially away from it; if the charge is negative, field lines point radially towards it . 

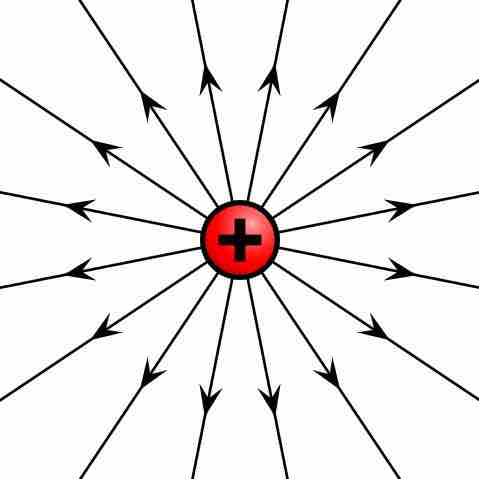
Electric field of positive point charge

The electric field of a positively charged particle points radially away from the charge.

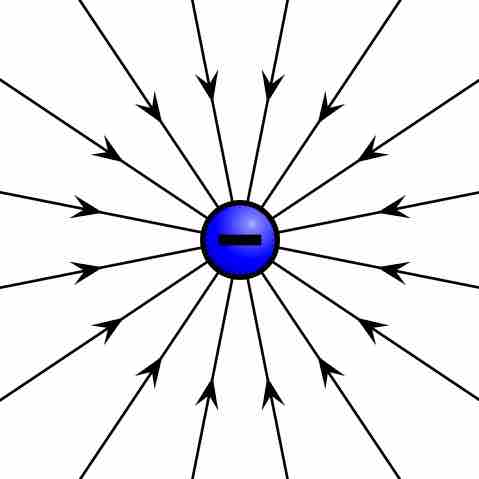
Electric field of negative point charge

The electric field of a negatively charged particle points radially toward the particle.

The reason for these directions can be seen in the derivation of the electric field of a point charge. Let's first take a look at the definition of the electric field of a point particle:

$\displaystyle{\vec{E} = \frac{1}{4\pi\epsilon_o}\frac{q}{r^2}\hat{r} = k\frac{q}{r^2}\hat{r}}$

The above equation is defined in radial coordinates, which can be seen in . The constant k is a result of simply combining the constants together, and q is the charge of the particle creating the electric field. This charge is either positive or negative. If the charge is positive, as shown above, the electric field will be pointing in a positive radial direction from the charge q (away from the charge).

As a demonstration of this phenomenon, if we now place another positive charge, Q (called the test charge), at some radial distance, R, away from the original particle, the test charge will feel a force given by

$\displaystyle{\vec{F} = Q\vec{E} = Q\frac{1}{4\pi\epsilon_o}\frac{q}{R^2}\hat{r}}$

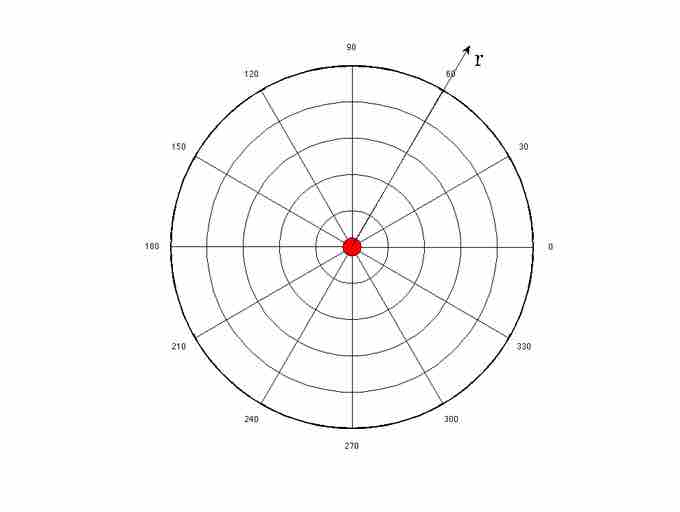
Radial Coordinate System

The electric field of a point charge is defined in radial coordinates. The positive r direction points away from the origin, and the negative r direction points toward the origin. The electric field of a point charge is symmetric with respect to the θdirection.

The thing to keep in mind is that the force above is acting on the test charge Q, in the positive radial direction as defined by the original charge q. This means that because the charges are both positive and will repel one another, the force on the test charge points away from the original charge.

If the test charge were negative, the force felt on that charge would be:

$\vec{F} = Q\vec{E} = -Q\frac{1}{4\pi\epsilon_o}\frac{q}{R^2}\hat{r}$

Notice that this points in the negative $\hat{r}$ direction, which is toward the original charge. This makes sense because opposite charges attract, and the force on the test charge will tend to push it toward the original positive charge creating the field. The above mathematical description of the electric field of a point charge is known as Coulomb's law.