Economic order quantity is the order quantity that minimizes total inventory holding costs and ordering costs. It is one of the oldest classical production scheduling models. The framework used to determine this order quantity is also known as "Barabas EOQ Model" or "Barabas Formula. " The model was developed by Ford W. Harris in 1913, but R. H. Wilson, a consultant who applied it extensively, is given credit for his in-depth analysis.
We want to determine the optimal number of units to order so that we minimize the total cost associated with the purchase, delivery, and storage of the product. The required parameters to the solution are the total demand for the year, the purchase cost for each item, the fixed cost to place the order, and the storage cost for each item per year. Note that the number of times an order is placed will also affect the total cost, though this number can be determined from the other parameters.
Underlying assumptions are:
- The ordering cost is constant.
- The rate of demand is known, and spread evenly throughout the year.
- The lead time is fixed.
- The purchase price of the item is constant (i.e., no discount is available).
- The replenishment is made instantaneously; the whole batch is delivered at once.
- Only one product is involved.
Variables for the function are: Q = order quantity, Q*= optimal order quantity, D = annual demand quantity, S = fixed cost per order (not per unit, typically cost of ordering and shipping and handling. This is not the cost of goods), H = annual holding cost per unit (also known as carrying cost or storage cost) (warehouse space, refrigeration, insurance, etc., usually not related to the unit cost).
The single-item EOQ formula finds the minimum point of the following cost function:
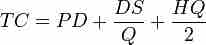
Economic order quantity
Economic order quantity function solving for Q.
Total Cost = purchase cost + ordering cost + holding cost
- Purchase cost: This is the variable cost of goods: purchase unit price × annual demand quantity. This is P×D.
- Ordering cost: This is the cost of placing orders: each order has a fixed cost S, and we need to order D/Q times per year. This is S × D/Q.
- Holding cost: the average quantity in stock (between fully replenished and empty) is Q/2, so this cost is H × Q/2.
To determine the minimum point of the total cost curve, partially differentiate the total cost with respect to Q (assume all other variables are constant) and set to 0:
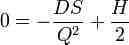
Equation
Equation to determine the minimum point of the total cost curve.
Solving for Q gives Q* (the optimal order quantity):
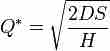
Equation 3
Equation solving for Q*