Dating Using Radioactive Decay

Radiometric Dating

Radiometric dating, often called radioactive dating, is a technique used to determine the age of materials such as rocks. It is based on a comparison between the observed abundance of a naturally occurring radioactive isotope and its decay products, using known decay rates. It is the principal source of information about the absolute age of rocks and other geological features, including the age of the Earth itself, and it can be used to date a wide range of natural and man-made materials.

The best-known radiometric dating techniques include radiocarbon dating, potassium-argon dating, and uranium-lead dating. By establishing geological timescales, radiometric dating provides a significant source of information about the ages of fossils and rates of evolutionary change, and it is also used to date archaeological materials, including ancient artifacts. The different methods of radiometric dating are accurate over different timescales, and they are useful for different materials.

Decay

After one half-life has elapsed, one half of the atoms of the nuclide in question will have decayed into a "daughter" nuclide, or decay product. In many cases, the daughter nuclide is radioactive, resulting in a decay chain. This chain eventually ends with the formation of a stable, nonradioactive daughter nuclide. Each step in such a chain is characterized by a distinct half-life. In these cases, the half-life of interest in radiometric dating is usually the longest one in the chain. This half-life will be the rate-limiting factor in the ultimate transformation of the radioactive nuclide into its stable daughter(s).

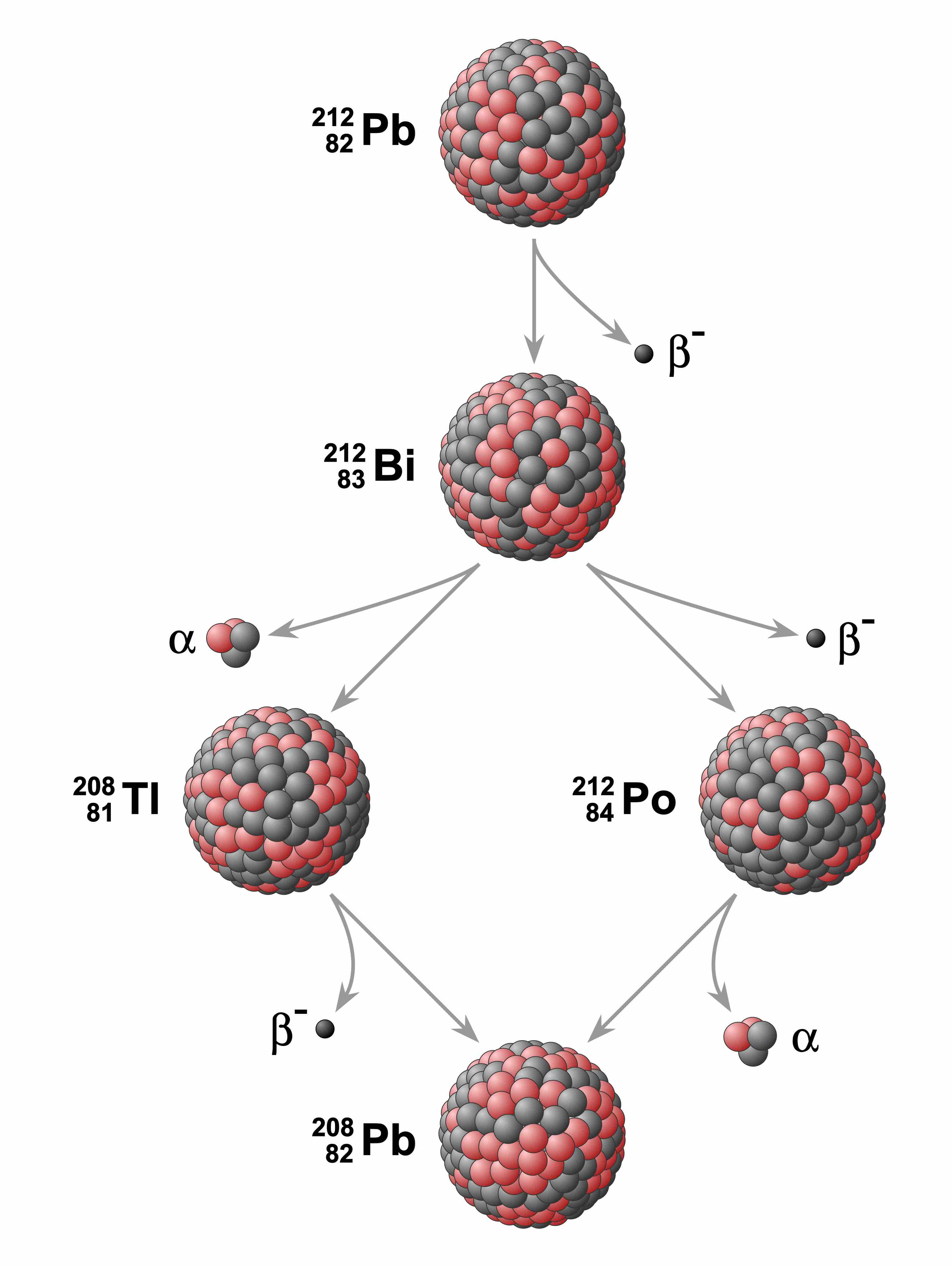
Lead decay chain

Example of a radioactive decay chain from lead-212 (212Pb) to lead-208 (208Pb) . Each parent nuclide spontaneously decays into a daughter nuclide (the decay product) via an α decay or a β decay. The final decay product, lead-208 (208Pb), is stable and can no longer undergo spontaneous radioactive decay.

Systems that have been exploited for radiometric dating have half-lives ranging from only about 10 years (e.g., tritium) to over 100 billion years (e.g., Samarium-147). However, in general, the half-life of a nuclide depends solely on its nuclear properties and is essentially a constant. Therefore, in any material containing a radioactive nuclide, the proportion of the original nuclide to its decay products changes in a predictable way as the original nuclide decays over time. This predictability allows the relative abundances of related nuclides to be used as a clock to measure the time it takes for the parent atom to decay into the daughter atom(s).

Accuracy and Contamination

Accurate radiometric dating generally requires that:

• The parent has a long enough half-life to ensure the parent will be present in significant amounts at the time of measurement.
• The half-life of the parent is accurately known.
• Enough of the daughter product is produced to be accurately measured and distinguished from the initial amount of the daughter present in the material.

The mathematical expression that relates radioactive decay to geologic time is:

$D={D}_{0}+N(t)({e}^{\lambda t}-1)$

Here, t is age of the sample; D is number of atoms of the daughter isotope in the sample; D₀ is number of atoms of the daughter isotope in the original composition; N is number of atoms of the parent isotope in the sample at time t (the present), given by N(t) = N_oe^-λt; and λ is the decay constant of the parent isotope, equal to the inverse of the radioactive half-life of the parent isotope times the natural logarithm of 2.

Example

A 100 g sample of Cs-137 is allowed to decay. Calculate the mass of Cs-137 that will be left after 90 years. The half-life of Cs-137 is 30 years.

First half-life (30 years): 100 grams of Cs-137 decays and 50 grams are left.

Second half-life (60 years total): The remaining 50 grams of Cs-137 decay and 25 grams are left.

Third half-life (90 years total): The remaining 25 grams of Cs-137 decay and 12.5 grams are left.