Balancing Redox Equations

Every balanced redox reaction is composed of two half-reactions: the oxidation half-reaction, and the reduction half-reaction. For example, look at the following redox reaction between iron and copper:

$Fe + Cu^{+2} \rightarrow Fe^{+2} + Cu$

In this reaction, iron is oxidized, and copper is reduced (or, iron is the reducing agent, and copper is the oxidizing agent.) We can split this reaction into two half-reactions. The oxidation half-reaction looks as follows:

$Fe \rightarrow Fe^{+2} + 2e^-$

This shows the oxidation of iron and the loss of two electrons. Notice that this equation is balanced in both mass and charge: we have one atom of iron on each side of the equation (mass is balanced), and the net charge on each side of the equation is equal to zero (charge is balanced).

Next, look at the reduction half-reaction:

$Cu^{+2} + 2e^- \rightarrow Cu$

This half-reaction explicitly shows the copper (II) ion gaining two electrons. Note again that the equation is balanced in mass and charge. Now that we have our two balanced half-reactions, we can combine them to get the full redox reaction:

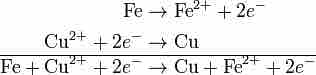
Adding the two halves of a redox reaction

These two halves of the reaction can be added like any other chemical equation. Once the equations are added, the electrons on each side cancel out.

Note that the two electrons on each side of the equation cancel out. This is very important, because the final balanced equation for any redox reaction should never contain any electrons.

Electrons moving from one pole of a battery through a circuit and back through the battery's other pole is an example of applied redox reaction.

Balancing Redox Equations in Acidic Solution: Basic Rules

If a reaction occurs in an acidic environment, you can balance the redox equation as follows:

1. Write the oxidation and reduction half-reactions, including the whole compound involved in the reaction—not just the element that is being reduced or oxidized.
2. Balance both reactions for all elements except oxygen and hydrogen.
3. If the oxygen atoms are not balanced in either reaction, add water molecules to the side missing the oxygen.
4. If the hydrogen atoms are not balanced, add hydrogen ions (H⁺) until the hydrogen atoms are balanced.
5. Multiply the half-reactions by the appropriate numbers so that they both have equal numbers of electrons.
6. Add the two equations to cancel out the electrons to balance the equation.

Balancing Redox Equations in Acidic Solution: Example

The following is an unbalanced redox equation that takes place in acidic solution:

$Cr_2O_7^{2-}(aq)+NO_2^-(aq) \rightarrow Cr^{3+}(aq) + NO_3^-(aq)$

First, we need to split this reaction into its two half-reactions. Let's start with the oxidation half-reaction:

$NO_2^-(aq) \rightarrow NO_3^-(aq)$

We need to balance this equation by mass. The equation is already balanced in nitrogens, but not oxygens. You can balance oxygen by adding the appropriate number of water molecules:

$H_2O(l) + NO_2^-(aq) \rightarrow NO_3^-(aq)$

Now the equation is balanced in oxygens, but not hydrogens. To balance hydrogens in acidic solution, we add H⁺(aq):

$H_2O(l) + NO_2^-(aq) \rightarrow NO_3^-(aq) + 2H^+(aq)$

The equation is now balanced in mass, but not charge. To balance the charge, we will add two electrons to the right side of the equation:

$H_2O(l) + NO_2^-(aq) \rightarrow NO_3^-(aq) + 2H^+(aq) + 2e^-$

The charge is now balanced; each side of the equation has an equal net charge of -1.

Let's now move on to the reduction half-reaction:

$Cr_2O_7^{2-} \rightarrow Cr^{3+}$

Once again, we will balance mass first, balancing oxygens by adding in water molecules:

$Cr_2O_7^{2-} \rightarrow 2 Cr^{3+} + 7 H_2O(l)$

Again, since we are in acidic solution, we balance hydrogens by adding H⁺(aq):

$14 H^+(aq) + Cr_2O_7^{2-} \rightarrow 2 Cr^{3+} + 7 H_2O(l)$

The equation is now balanced for mass, and we need only balance for charge. As it stands now, the left side of the equation has a net charge of +14 - 2 = +12. The right side of the equation has a net charge of +6 (2 x +3 = +6). We therefore need to add 6 electrons to the right side of the equation to balance the charges:

$6e^- + 14 H^+(aq) + Cr_2O_7^{2-} \rightarrow 2 Cr^{3+} + 7 H_2O(l)$

Now we have balanced both of our half-reactions. To sum up so far:

Balanced oxidation half-reaction: $H_2O(l) + NO_2^-(aq) \rightarrow NO_3^-(aq) + 2H^+(aq) + 2e^-$

Balanced reduction half-reaction: $6e^- + 14 H^+(aq) + Cr_2O_7^{2-} \rightarrow 2 Cr^{3+} + 7 H_2O(l)$

Lastly, in order to get our full balanced redox equation, we need to add our half-reactions so that all the electrons cancel out. For this reaction, we can multiply the first half-reaction by 3:

$3 \times (H_2O(l) + NO_2^-(aq) \rightarrow NO_3^-(aq) + 2H^+(aq) + 2e^-) =$

$3 H_2O(l) + 3NO_2^-(aq) \rightarrow 3NO_3^-(aq) + 6H^+(aq) + 6e^-$

Now we can add our half-reactions together:

$3 H_2O(l) + 3NO_2^-(aq) \rightarrow 3NO_3^-(aq) + 6H^+(aq) + 6e^-$

$6e^- + 14 H^+(aq) + Cr_2O_7^{2-} \rightarrow 2 Cr^{3+} + 7 H_2O(l)$

------------------------------------------------------------------------------------------------------

$6e^-+3H_2O(l) + 3NO_2^-(aq) + 14 H^+(aq) + Cr_2O_7^{2-} \rightarrow 2 Cr^{3+} + 7 H_2O(l) + 3NO_3^-(aq)+6H^+(aq)+6e^-$

Canceling out all common terms, including the electrons, we get our final balanced equation:

$3NO_2^-(aq) + 8 H^+(aq) + Cr_2O_7^{2-} \rightarrow 2 Cr^{3+} + 4 H_2O(l) + 3NO_3^-(aq)$

Notice that the final equation is balanced in mass as well as charge (each side of the equation has a net charge of +3).

Although this example seems intimidating, balancing redox reactions in acidic solution becomes much easier with careful practice.

Balancing Redox Reactions in Basic Solution - Example

If a redox reaction occurs in basic solution, we proceed as we did above, with one minor difference: once we have added H⁺(aq) to balance hydrogens, we simply add the same number of hydroxides to both sides of the equation. For instance:

$H_2O(l) + NO_2^-(aq) \rightarrow NO_3^-(aq) + 2H^+(aq)$

The half-reaction above is balanced for mass in acidic solution. If we are in basic solution, however, we would need to add 2 hydroxides to both sides of the equation:

$2OH^-(aq)+H_2O(l) + NO_2^-(aq) \rightarrow NO_3^-(aq) + 2H^+(aq) + 2OH^-(aq)$

Notice that we have both H+(aq) and OH-(aq) on the right side of the equation. These species will neutralize each other to form water, so we can rewrite this as follows:

$2OH^-(aq)+H_2O(l) + NO_2^-(aq) \rightarrow NO_3^-(aq) + 2H_2O(l)$

Lastly, because we have water molecules on both sides of the equation, we cancel out like terms to give us:

$2OH^-(aq)+NO_2^-(aq) \rightarrow NO_3^-(aq) + H_2O(l)$

This half-reaction is now balanced for mass in basic solution. From here, we proceed just as we did above in acidic solution: balance the charge by adding the appropriate number of electrons.

Balancing redox equations can certainly be complicated and time-consuming, so it is wise to practice them extensively.