Applications of Second-Order Differential Equations

Examples of homogeneous or nonhomogeneous second-order linear differential equation can be found in many different disciplines, such as physics, economics, and engineering. In this atom, we will learn about the harmonic oscillator, which is one of the simplest yet most important mechanical system in physics.

Harmonic oscillator

In classical mechanics, a harmonic oscillator is a system that, when displaced from its equilibrium position, experiences a restoring force, $F$, proportional to the displacement, $x$: $\vec F = -k \vec x \,$, where $k$ is a positive constant. The system under consideration could be an object attached to a spring, a pendulum, etc. Electronic circuits such as RLC circuits are also described by similar equations.

Simple harmonic oscillation

If $F$ is the only force acting on the system, the system is called a simple harmonic oscillator, and it undergoes simple harmonic motion: sinusoidal oscillations about the equilibrium point, with a constant amplitude and a constant frequency. The equation of motion is given as:

 $\displaystyle{F = m a = m \frac{\mathrm{d}^2x}{\mathrm{d}t^2} = -k x}$

Therefore, we end up with a homogeneous second-order linear differential equation:

 $\displaystyle{m \frac{\mathrm{d}^2x}{\mathrm{d}t^2} + k x = 0}$

Note that the function $x(t) = A\cos\left( \omega_0 t+\phi\right)$ satisfies the equation where $\omega_0 = \sqrt{\frac{k}{m}} = \frac{2\pi}{T}$. $\omega_0$ is called angular velocity, and the constants $A$ and $\phi$ are determined from initial conditions of the motion.

Damped harmonic oscillator

In real oscillators, friction (or damping) slows the motion of the system. In many vibrating systems the frictional force $Ff$ can be modeled as being proportional to the velocity v of the object: $Ff = −cv$, where $c$ is called the viscous damping coefficient. Including this additional term, the equation of motion is given as:

$\displaystyle{\frac{\mathrm{d}^2x}{\mathrm{d}t^2} + 2\zeta\omega_0\frac{\mathrm{d}x}{\mathrm{d}t} + \omega_0^{\,2} x = 0}$ 

where $\zeta = \frac{c}{2 \sqrt{mk}}$ is called the "damping ratio."

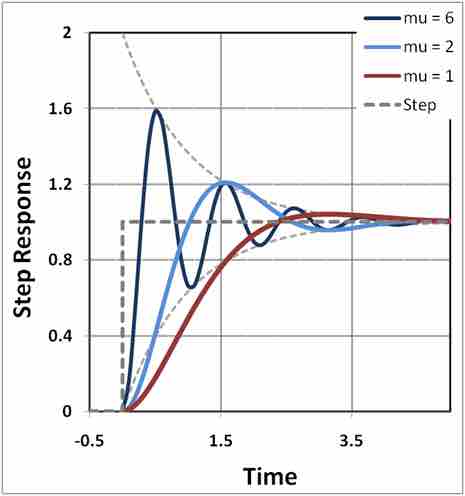
Damped Harmonic Oscillators

A solution of damped harmonic oscillator. Curves in different colors show various responses depending on the damping ratio.

Driven harmonic oscillator: Driven harmonic oscillators are damped oscillators further affected by an externally applied force $F(t)$. Newton's 2nd law ($F=ma$) takes the form:

$\displaystyle{F(t)-kx-c\frac{\mathrm{d}x}{\mathrm{d}t}=m\frac{\mathrm{d}^2x}{\mathrm{d}t^2}}$

It is usually rewritten into the form:

$\displaystyle{\frac{\mathrm{d}^2x}{\mathrm{d}t^2} + 2\zeta\omega_0\frac{\mathrm{d}x}{\mathrm{d}t} + \omega_0^2 x = \frac{F(t)}{m}}$

which is a nonhomogeneous second-order linear differential equation.