Exponential Decay

Exponential decay is the result of a function that decreases in proportion to its current value.

Learning Objective

Use the exponential decay formula to calculate how much of something is left after a period of time

Key Points

Exponential decrease can be modeled as: $N(t)=N_0e^{-\lambda t}$ where $N$ is the quantity, $N_0$ is the initial quantity, $\lambda$ is the decay constant, and $t$ is time.
Oftentimes, half-life is used to describe the amount of time required for half of a sample to decay. It can be defined mathematically as: $t_{1/2}=\frac{ln(2)}{\lambda }$where $t_{1/2}$ is half-life.
Half-life can be inserted into the exponential decay model as such: $N(t)=N_0(\frac{1}{2})^{t/t_{1/2}}$. Notice how the exponential changes, but the form of the function remains.

Terms

isotope
Any of two or more forms of an element where the atoms have the same number of protons, but a different number of neutrons. As a consequence, atoms for the same isotope will have the same atomic number but a different mass number (atomic weight).
half-life
The time it takes for a substance (drug, radioactive nuclide, or other) to lose half of its pharmacological, physiological, biological, or radiological activity.

Full Text

Introduction

Just as it is possible for a variable to grow exponentially as a function of another, so can the a variable decrease exponentially. Consider the decrease of a population that occurs at a rate proportional to its value. This rate at which the population is decreasing remains constant but as the population is continually decreasing the overall decline becomes less and less steep.

Exponential rate of change can be modeled algebraically by the following formula:

$N(t)=N_0e^{-\lambda t}$

where $N$ is the quantity, $N{_0}$ is the initial quantity, $\lambda$ is the decay constant, and $t$ is time. The decay constant is indeed a constant, but the form of the equation (the negative exponent of e) results in an ever-changing rate of decline.

Half Life

The time it takes for a substance (drug, radioactive nuclide, or other) to lose half of its pharmacological, physiological, biological, or radiological activity is called its half-life. The exponential decay of the substance is a time-dependent decline and a prime example of exponential decay.

As an example let us assume we have a $100$ pounds of a substance with a half-life of $5$ years. Then in $5$ years half the amount ($50$ pounds) remains. In another $5$ years there will be $25$ pounds remaining. In another $5$ years, or $15$ years from the beginning, there will be $12.5$. The amount by which the substance decreases, is itself slowly decreasing.

Use of Half Life in Carbon Dating

Half-life is very useful in determining the age of historical artifacts through a process known as carbon dating. Given a sample of carbon in an ancient, preserved piece of flesh, the age of the sample can be determined based on the percentage of radioactive carbon-13 remaining. 1.1% of carbon is C-13 and it decays to carbon-12. C-13 has a half-life of 5700 years—that is, in 5700 years, half of a sample of C-13 will have converted to C-12, which represents approximately all the remaining carbon. Using this information it is possible to determine the age of the artifact given the amount of C-13 it presently contains, and comparing it to the amount of C-13 it should contain.

Half-life can be mathematically defined as:

$\displaystyle t_{1/2}=\frac{ln(2)}{\lambda }$

It can also be conveniently inserted into the exponential decay formula as follows:

$\displaystyle N(t)=N_0(\frac{1}{2})^{t/t_{1/2}}$

Thus, if a sample is found to contain 0.55% of its carbon as C-13 (exactly half of the usual 1.1%), it can be calculated that the sample has undergone exactly one half-life, and is thus 5,700 years old.

Below is a graph highlighting exponential decay of a radioactive substance. Using the graph, find that half-life.

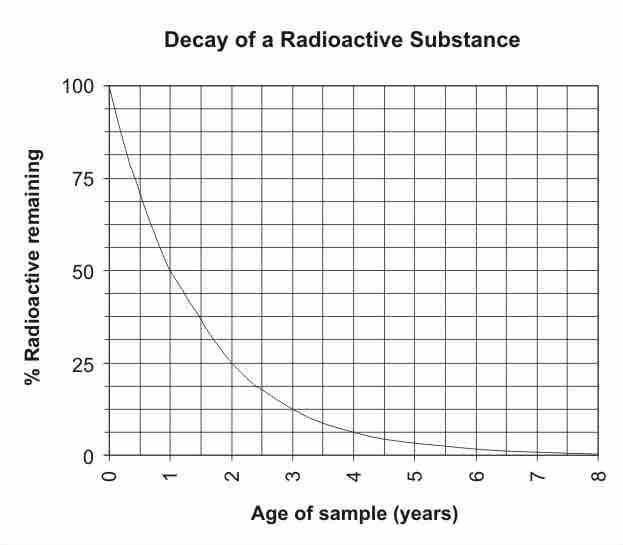
Graph depicting radioactive decay

The amount of a substance undergoing radioactive decay decreases exponentially, eventually reaching zero. Since there is 50% of the substance left after 1 year, the half-life is 1 year.

[ edit ]

Prev Concept

Interest Compounded Continuously

What is a Matrix?

Next Concept