9.2 Concentration

Learning Objectives

  1. Express the amount of solute in a solution in various concentration units.
  2. Use molarity to determine quantities in chemical reactions.
  3. Determine the resulting concentration of a diluted solution.

To define a solution precisely, we need to state its concentrationHow much solute is dissolved in a certain amount of solvent.: how much solute is dissolved in a certain amount of solvent. Words such as dilute or concentrated are used to describe solutions that have a little or a lot of dissolved solute, respectively, but these are relative terms whose meanings depend on various factors.

Solubility

There is usually a limit to how much solute will dissolve in a given amount of solvent. This limit is called the solubilityThe limit of how much solute can be dissolved in a given amount of solvent. of the solute. Some solutes have a very small solubility, while other solutes are soluble in all proportions. Table 9.2 "Solubilities of Various Solutes in Water at 25°C (Except as Noted)" lists the solubilities of various solutes in water. Solubilities vary with temperature, so Table 9.2 "Solubilities of Various Solutes in Water at 25°C (Except as Noted)" includes the temperature at which the solubility was determined.

Table 9.2 Solubilities of Various Solutes in Water at 25°C (Except as Noted)

Substance Solubility (g in 100 mL of H2O)
AgCl(s) 0.019
C6H6(ℓ) (benzene) 0.178
CH4(g) 0.0023
CO2(g) 0.150
CaCO3(s) 0.058
CaF2(s) 0.0016
Ca(NO3)2(s) 143.9
C6H12O6 (glucose) 120.3 (at 30°C)
KBr(s) 67.8
MgCO3(s) 2.20
NaCl(s) 36.0
NaHCO3(s) 8.41
C12H22O11 (sucrose) 204.0 (at 20°C)

If a solution contains so much solute that its solubility limit is reached, the solution is said to be saturatedA solution whose solute is at its solubility limit., and its concentration is known from information contained in Table 9.2 "Solubilities of Various Solutes in Water at 25°C (Except as Noted)". If a solution contains less solute than the solubility limit, it is unsaturatedA solution whose solute is less than its solubility limit.. Under special circumstances, more solute can be dissolved even after the normal solubility limit is reached; such solutions are called supersaturated and are not stable. If the solute is solid, excess solute can easily recrystallize. If the solute is a gas, it can bubble out of solution uncontrollably, like what happens when you shake a soda can and then immediately open it.

Note

Recrystallization of excess solute from a supersaturated solution usually gives off energy as heat. Commercial heat packs containing supersaturated sodium acetate (NaC2H3O2) take advantage of this phenomenon. You can probably find them at your local drugstore.

Most solutions we encounter are unsaturated, so knowing the solubility of the solute does not accurately express the amount of solute in these solutions. There are several common ways of specifying the concentration of a solution.

Percent Composition

There are several ways of expressing the concentration of a solution by using a percentage. The mass/mass percentA concentration unit that relates the mass of the solute to the mass of the solution. (% m/m) is defined as the mass of a solute divided by the mass of a solution times 100:

% m/m = mass of solutemass of solution×100%

If you can measure the masses of the solute and the solution, determining the mass/mass percent is easy. Each mass must be expressed in the same units to determine the proper concentration.

Example 2

A saline solution with a mass of 355 g has 36.5 g of NaCl dissolved in it. What is the mass/mass percent concentration of the solution?

Solution

We can substitute the quantities given in the equation for mass/mass percent:

% m/m=36.5 g355 g×100%=10.3%

Skill-Building Exercise

  1. A dextrose (also called D-glucose, C6H12O6) solution with a mass of 2.00 × 102 g has 15.8 g of dextrose dissolved in it. What is the mass/mass percent concentration of the solution?

For gases and liquids, volumes are relatively easy to measure, so the concentration of a liquid or a gas solution can be expressed as a volume/volume percentA concentration unit that relates the volume of the solute to the volume of the solution. (% v/v): the volume of a solute divided by the volume of a solution times 100:

% v/v = volume of solutevolume of solution×100%

Again, the units of the solute and the solution must be the same. A hybrid concentration unit, mass/volume percentA concentration unit that relates the mass of the solute to the volume of the solution. (% m/v), is commonly used for intravenous (IV) fluids (Figure 9.2 "Mass/Volume Percent"). It is defined as the mass in grams of a solute, divided by volume in milliliters of solution times 100:

% m/v = mass of solute (g)volume of solution (mL)×100%

Each percent concentration can be used to produce a conversion factor between the amount of solute, the amount of solution, and the percent. Furthermore, given any two quantities in any percent composition, the third quantity can be calculated, as the following example illustrates.

Example 3

A sample of 45.0% v/v solution of ethanol (C2H5OH) in water has a volume of 115 mL. What volume of ethanol solute does the sample contain?

Solution

A percentage concentration is simply the number of parts of solute per 100 parts of solution. Thus, the percent concentration of 45.0% v/v implies the following:

45.0% v/v → 45 mL C2H5OH100 mL solution

That is, there are 45 mL of C2H5OH for every 100 mL of solution. We can use this fraction as a conversion factor to determine the amount of C2H5OH in 115 mL of solution:

115 mL solution×45 mL C2H5OH100 mL solution=51.8 mL C2H5OH

Note

The highest concentration of ethanol that can be obtained normally is 95% ethanol, which is actually 95% v/v.

Skill-Building Exercise

  1. What volume of a 12.75% m/v solution of glucose (C6H12O6) in water is needed to obtain 50.0 g of C6H12O6?

Example 4

A normal saline IV solution contains 9.0 g of NaCl in every liter of solution. What is the mass/volume percent of normal saline?

Solution

We can use the definition of mass/volume percent, but first we have to express the volume in milliliter units:

1 L = 1,000 mL

Because this is an exact relationship, it does not affect the significant figures of our result.

% m/v = 9.0 g NaCl1,000 mL solution×100%=0.90% m/v

Skill-Building Exercise

  1. The chlorine bleach that you might find in your laundry room is typically composed of 27.0 g of sodium hypochlorite (NaOCl), dissolved to make 500.0 mL of solution. What is the mass/volume percent of the bleach?

In addition to percentage units, the units for expressing the concentration of extremely dilute solutions are parts per million (ppm)The mass of a solute compared to the mass of a solution times 1,000,000. and parts per billion (ppb)The mass of a solute compared to the mass of a solution times 1,000,000,000.. Both of these units are mass based and are defined as follows:

ppm=mass of solutemass of solution×1,000,000 ppb=mass of solutemass of solution×1,000,000,000

Note

Similar to parts per million and parts per billion, related units include parts per thousand (ppth) and parts per trillion (ppt).

Concentrations of trace elements in the body—elements that are present in extremely low concentrations but are nonetheless necessary for life—are commonly expressed in parts per million or parts per billion. Concentrations of poisons and pollutants are also described in these units. For example, cobalt is present in the body at a concentration of 21 ppb, while the State of Oregon’s Department of Agriculture limits the concentration of arsenic in fertilizers to 9 ppm.

Note

In aqueous solutions, 1 ppm is essentially equal to 1 mg/L, and 1 ppb is equivalent to 1 µg/L.

Example 5

If the concentration of cobalt in a human body is 21 ppb, what mass in grams of Co is present in a body having a mass of 70.0 kg?

Solution

A concentration of 21 ppb means “21 g of solute per 1,000,000,000 g of solution.” Written as a conversion factor, this concentration of Co is as follows:

21 ppb Co → 21 g Co1,000,000,000 g solution

We can use this as a conversion factor, but first we must convert 70.0 kg to gram units:

70.0 kg×1,000 g1 kg=7.00×104 g

Now we determine the amount of Co:

7.00×104 g solution×21 g Co1,000,000,000 g solution=0.0015 g Co

This is only 1.5 mg.

Skill-Building Exercise

  1. An 85 kg body contains 0.012 g of Ni. What is the concentration of Ni in parts per million?

Molarity

Another way of expressing concentration is to give the number of moles of solute per unit volume of solution. Such concentration units are useful for discussing chemical reactions in which a solute is a product or a reactant. Molar mass can then be used as a conversion factor to convert amounts in moles to amounts in grams.

MolarityNumber of moles of solute per liter of solution. is defined as the number of moles of a solute dissolved per liter of solution:

molarity=number of moles of solutenumber of liters of solution

Molarity is abbreviated M (often referred to as “molar”), and the units are often abbreviated as mol/L. It is important to remember that “mol” in this expression refers to moles of solute and that “L” refers to liters of solution. For example, if you have 1.5 mol of NaCl dissolved in 0.500 L of solution, its molarity is therefore

1.5 mol NaCl0.500 L solution=3.0 M NaCl

which is read as “three point oh molar sodium chloride.” Sometimes (aq) is added when the solvent is water, as in “3.0 M NaCl(aq).”

Before a molarity concentration can be calculated, the amount of the solute must be expressed in moles, and the volume of the solution must be expressed in liters, as demonstrated in the following example.

Example 6

What is the molarity of an aqueous solution of 25.0 g of NaOH in 750 mL?

Solution

Before we substitute these quantities into the definition of molarity, we must convert them to the proper units. The mass of NaOH must be converted to moles of NaOH. The molar mass of NaOH is 40.00 g/mol:

25.0 g NaOH×1 mol NaOH40.00 g NaOH=0.625 mol NaOH

Next, we convert the volume units from milliliters to liters:

750 mL×1 L1,000 mL=0.750 L

Now that the quantities are expressed in the proper units, we can substitute them into the definition of molarity:

M=0.625 mol NaOH0.750 L=0.833 M NaOH

Skill-Building Exercise

  1. If a 350 mL cup of coffee contains 0.150 g of caffeine (C8H10N4O2), what is the molarity of this caffeine solution?

The definition of molarity can also be used to calculate a needed volume of solution, given its concentration and the number of moles desired, or the number of moles of solute (and subsequently, the mass of the solute), given its concentration and volume. The following example illustrates this.

Example 7

  1. What volume of a 0.0753 M solution of dimethylamine [(CH3)2NH] is needed to obtain 0.450 mol of the compound?
  2. Ethylene glycol (C2H6O2) is mixed with water to make auto engine coolants. How many grams of C2H6O2 are in 5.00 L of a 6.00 M aqueous solution?

Solution

In both parts, we will use the definition of molarity to solve for the desired quantity.

  1. 0.0753 M=0.450 mol (CH3)2NHvolume of solution

    To solve for the volume of solution, we multiply both sides by volume of solution and divide both sides by the molarity value to isolate the volume of solution on one side of the equation:

    volume of solution=0.450 mol (CH3)2NH0.0753 M=5.98 L

    Note that because the definition of molarity is mol/L, the division of mol by M yields L, a unit of volume.

  2. The molar mass of C2H6O2 is 62.08 g/mol., so

    6.00 M=moles of solute5.00 L

    To solve for the number of moles of solute, we multiply both sides by the volume:

    moles of solute = (6.00 M)(5.00 L) = 30.0 mol

    Note that because the definition of molarity is mol/L, the product M × L gives mol, a unit of amount. Now, using the molar mass of C3H8O3, we convert mol to g:

    30.0 mol×62.08 g mol=1,860 g

    Thus, there are 1,860 g of C2H6O2 in the specified amount of engine coolant.

Note

Dimethylamine has a “fishy” odor. In fact, organic compounds called amines cause the odor of decaying fish. (For more information about amines, see Chapter 15 "Organic Acids and Bases and Some of Their Derivatives", Section 15.1 "Functional Groups of the Carboxylic Acids and Their Derivatives" and Section 15.11 "Amines: Structures and Names" through Section 15.13 "Amines as Bases".)

Skill-Building Exercise

  1. What volume of a 0.0902 M solution of formic acid (HCOOH) is needed to obtain 0.888 mol of HCOOH?

  2. Acetic acid (HC2H3O2) is the acid in vinegar. How many grams of HC2H3O2 are in 0.565 L of a 0.955 M solution?

Using Molarity in Stoichiometry Problems

Of all the ways of expressing concentration, molarity is the one most commonly used in stoichiometry problems because it is directly related to the mole unit. Consider the following chemical equation:

HCl(aq) + NaOH(s) → H2O(ℓ) + NaCl(aq)

Suppose we want to know how many liters of aqueous HCl solution will react with a given mass of NaOH. A typical approach to answering this question is as follows:

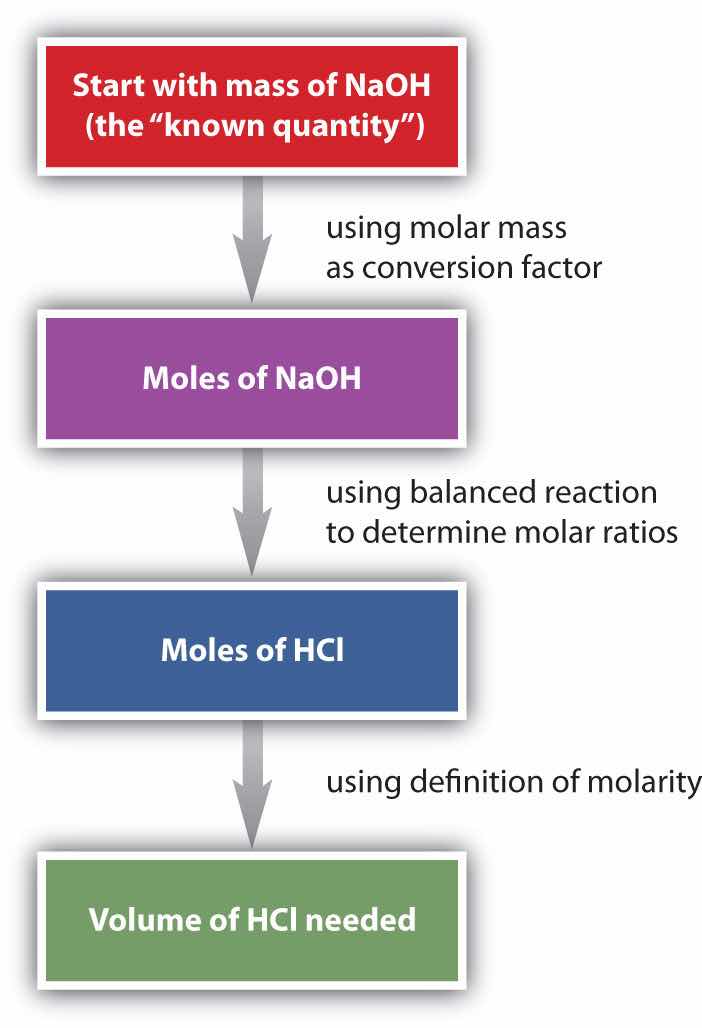
In itself, each step is a straightforward conversion. It is the combination of the steps that is a powerful quantitative tool for problem solving.

Example 8

How many milliliters of a 2.75 M HCl solution are needed to react with 185 g of NaOH? The balanced chemical equation for this reaction is as follows:

HCl(aq) + NaOH(s) → H2O(ℓ) + NaCl(aq)

Solution

We will follow the flowchart to answer this question. First, we convert the mass of NaOH to moles of NaOH using its molar mass, 40.00 g/mol:

185 g NaOH×1 mol NaOH40.00 g NaOH=4.63 mol NaOH

Using the balanced chemical equation, we see that there is a one-to-one ratio of moles of HCl to moles of NaOH. We use this to determine the number of moles of HCl needed to react with the given amount of NaOH:

4.63 mol NaOH×1 mol HCl1 mol NaOH=4.63 mol HCl

Finally, we use the definition of molarity to determine the volume of 2.75 M HCl needed:

2.75 M HCl=4.63 mol HClvolume of HCl solution volume of HCl=4.63 mol HCl2.75 M HCl=1.68 L×1,000 mL1 L=1,680 mL

We need 1,680 mL of 2.75 M HCl to react with the NaOH.

Skill-Building Exercise

  1. How many milliliters of a 1.04 M H2SO4 solution are needed to react with 98.5 g of Ca(OH)2? The balanced chemical equation for the reaction is as follows:

    H2SO4(aq) + Ca(OH)2(s) → 2H2O(ℓ) + CaSO4(aq)

The general steps for performing stoichiometry problems such as this are shown in Figure 9.3 "Diagram of Steps for Using Molarity in Stoichiometry Calculations". You may want to consult this figure when working with solutions in chemical reactions. The double arrows in Figure 9.3 "Diagram of Steps for Using Molarity in Stoichiometry Calculations" indicate that you can start at either end of the chart and, after a series of simple conversions, determine the quantity at the other end.

Figure 9.3 Diagram of Steps for Using Molarity in Stoichiometry Calculations

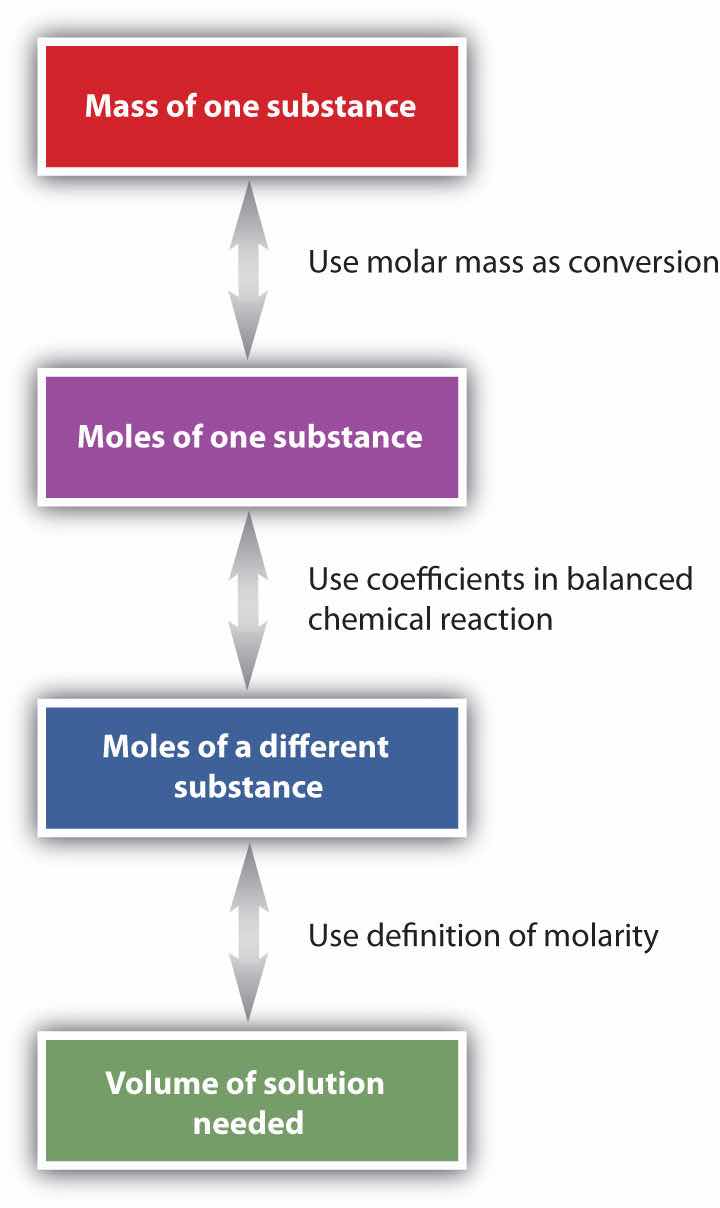
When using molarity in stoichiometry calculations, a specific sequence of steps usually leads you to the correct answer.

Many of the fluids found in our bodies are solutions. The solutes range from simple ionic compounds to complex proteins. Table 9.3 "Approximate Concentrations of Various Solutes in Some Solutions in the Body*" lists the typical concentrations of some of these solutes.

Table 9.3 Approximate Concentrations of Various Solutes in Some Solutions in the Body*

Solution Solute Concentration (M)
blood plasma Na+ 0.138
K+ 0.005
Ca2+ 0.004
Mg2+ 0.003
Cl− 0.110
HCO3− 0.030
stomach acid HCl 0.10
urine NaCl 0.15
PO43− 0.05
NH2CONH2 (urea) 0.30
*Note: Concentrations are approximate and can vary widely.

Looking Closer: The Dose Makes the Poison

Why is it that we can drink 1 qt of water when we are thirsty and not be harmed, but if we ingest 0.5 g of arsenic, we might die? There is an old saying: the dose makes the poison. This means that what may be dangerous in some amounts may not be dangerous in other amounts.

Take arsenic, for example. Some studies show that arsenic deprivation limits the growth of animals such as chickens, goats, and pigs, suggesting that arsenic is actually an essential trace element in the diet. Humans are constantly exposed to tiny amounts of arsenic from the environment, so studies of completely arsenic-free humans are not available; if arsenic is an essential trace mineral in human diets, it is probably required on the order of 50 ppb or less. A toxic dose of arsenic corresponds to about 7,000 ppb and higher, which is over 140 times the trace amount that may be required by the body. Thus, arsenic is not poisonous in and of itself. Rather, it is the amount that is dangerous: the dose makes the poison.

Similarly, as much as water is needed to keep us alive, too much of it is also risky to our health. Drinking too much water too fast can lead to a condition called water intoxication, which may be fatal. The danger in water intoxication is not that water itself becomes toxic. It is that the ingestion of too much water too fast dilutes sodium ions, potassium ions, and other salts in the bloodstream to concentrations that are not high enough to support brain, muscle, and heart functions. Military personnel, endurance athletes, and even desert hikers are susceptible to water intoxication if they drink water but do not replenish the salts lost in sweat. As this example shows, even the right substances in the wrong amounts can be dangerous!

Equivalents

Concentrations of ionic solutes are occasionally expressed in units called equivalents (Eq)One mole of charge (either positive or negative).. One equivalent equals 1 mol of positive or negative charge. Thus, 1 mol/L of Na+(aq) is also 1 Eq/L because sodium has a 1+ charge. A 1 mol/L solution of Ca2+(aq) ions has a concentration of 2 Eq/L because calcium has a 2+ charge. Dilute solutions may be expressed in milliequivalents (mEq)—for example, human blood plasma has a total concentration of about 150 mEq/L. (For more information about the ions present in blood plasma, see Chapter 3 "Ionic Bonding and Simple Ionic Compounds", Section 3.3 "Formulas for Ionic Compounds".)

Dilution

When solvent is added to dilute a solution, the volume of the solution changes, but the amount of solute does not change. Before dilution, the amount of solute was equal to its original concentration times its original volume:

amount in moles = (concentration × volume)initial

After dilution, the same amount of solute is equal to the final concentration times the final volume:

amount in moles = (concentration × volume)final

To determine a concentration or amount after a dilution, we can use the following equation:

(concentration × volume)initial = (concentration × volume)final

Any units of concentration and volume can be used, as long as both concentrations and both volumes have the same unit.

Example 9

A 125 mL sample of 0.900 M NaCl is diluted to 1,125 mL. What is the final concentration of the diluted solution?

Solution

Because the volume units are the same, and we are looking for the molarity of the final solution, we can use (concentration × volume)initial = (concentration × volume)final:

(0.900 M × 125 mL) = (concentration × 1,125 mL)

We solve by isolating the unknown concentration by itself on one side of the equation. Dividing by 1,125 mL gives

concentration = 0.900 M×125 mL1,125 mL=0.100 M

as the final concentration.

Skill-Building Exercise

  1. A nurse uses a syringe to inject 5.00 mL of 0.550 M heparin solution (heparin is an anticoagulant drug) into a 250 mL IV bag, for a final volume of 255 mL. What is the concentration of the resulting heparin solution?

Concept Review Exercises

  1. What are some of the units used to express concentration?

  2. Distinguish between the terms solubility and concentration.

Answers

  1. % m/m, % m/v, ppm, ppb, molarity, and Eq/L (answers will vary)

  2. Solubility is typically a limit to how much solute can dissolve in a given amount of solvent. Concentration is the quantitative amount of solute dissolved at any concentration in a solvent.

Key Takeaways

  • Various concentration units are used to express the amounts of solute in a solution.
  • Concentration units can be used as conversion factors in stoichiometry problems.
  • New concentrations can be easily calculated if a solution is diluted.

Exercises

  1. Define solubility. Do all solutes have the same solubility?

  2. Explain why the terms dilute or concentrated are of limited usefulness in describing the concentration of solutions.

  3. If the solubility of sodium chloride (NaCl) is 30.6 g/100 mL of H2O at a given temperature, how many grams of NaCl can be dissolved in 250.0 mL of H2O?

  4. If the solubility of glucose (C6H12O6) is 120.3 g/100 mL of H2O at a given temperature, how many grams of C6H12O6 can be dissolved in 75.0 mL of H2O?

  5. How many grams of sodium bicarbonate (NaHCO3) can a 25.0°C saturated solution have if 150.0 mL of H2O is used as the solvent?

  6. If 75.0 g of potassium bromide (KBr) are dissolved in 125 mL of H2O, is the solution saturated, unsaturated, or supersaturated?

  7. Calculate the mass/mass percent of a saturated solution of NaCl. Use the data from Table 9.2 "Solubilities of Various Solutes in Water at 25°C (Except as Noted)", assume that masses of the solute and the solvent are additive, and use the density of H2O (1.00 g/mL) as a conversion factor.

  8. Calculate the mass/mass percent of a saturated solution of MgCO3 Use the data from Table 9.2 "Solubilities of Various Solutes in Water at 25°C (Except as Noted)", assume that masses of the solute and the solvent are additive, and use the density of H2O (1.00 g/mL) as a conversion factor.

  9. Only 0.203 mL of C6H6 will dissolve in 100.000 mL of H2O. Assuming that the volumes are additive, find the volume/volume percent of a saturated solution of benzene in water.

  10. Only 35 mL of aniline (C6H5NH2) will dissolve in 1,000 mL of H2O. Assuming that the volumes are additive, find the volume/volume percent of a saturated solution of aniline in water.

  11. A solution of ethyl alcohol (C2H5OH) in water has a concentration of 20.56% v/v. What volume of C2H5OH is present in 255 mL of solution?

  12. What mass of KCl is present in 475 mL of a 1.09% m/v aqueous solution?

  13. The average human body contains 5,830 g of blood. What mass of arsenic is present in the body if the amount in blood is 0.55 ppm?

  14. The Occupational Safety and Health Administration has set a limit of 200 ppm as the maximum safe exposure level for carbon monoxide (CO). If an average breath has a mass of 1.286 g, what is the maximum mass of CO that can be inhaled at that maximum safe exposure level?

  15. Which concentration is greater—15 ppm or 1,500 ppb?

  16. Express the concentration 7,580 ppm in parts per billion.

  17. What is the molarity of 0.500 L of a potassium chromate solution containing 0.0650 mol of K2CrO4?

  18. What is the molarity of 4.50 L of a solution containing 0.206 mol of urea [(NH2)2CO]?

  19. What is the molarity of a 2.66 L aqueous solution containing 56.9 g of NaBr?

  20. If 3.08 g of Ca(OH)2 is dissolved in enough water to make 0.875 L of solution, what is the molarity of the Ca(OH)2?

  21. What mass of HCl is present in 825 mL of a 1.25 M solution?

  22. What mass of isopropyl alcohol (C3H8O) is dissolved in 2.050 L of a 4.45 M aqueous C3H8O solution?

  23. What volume of 0.345 M NaCl solution is needed to obtain 10.0 g of NaCl?

  24. How many milliliters of a 0.0015 M cocaine hydrochloride (C17H22ClNO4) solution is needed to obtain 0.010 g of the solute?

  25. Aqueous calcium chloride reacts with aqueous silver nitrate according to the following balanced chemical equation:

    CaCl2(aq) + 2AgNO3(aq) → 2AgCl(s) + Ca(NO3)2(aq)

    How many moles of AgCl(s) are made if 0.557 L of 0.235 M CaCl2 react with excess AgNO3? How many grams of AgCl are made?

  26. Sodium bicarbonate (NaHCO3) is used to react with acid spills. The reaction with sulfuric acid (H2SO4) is as follows:

    2NaHCO3(s) + H2SO4(aq) → Na2SO4(aq) + 2H2O(ℓ) + 2CO2(g)

    If 27.6 mL of a 6.25 M H2SO4 solution were spilled, how many moles of NaHCO3 would be needed to react with the acid? How many grams of NaHCO3 is this?

  27. The fermentation of glucose to make ethanol and carbon dioxide has the following overall chemical equation:

    C6H12O6(aq) → 2C2H5OH(aq) + 2CO2(g)

    If 1.00 L of a 0.567 M solution of C6H12O6 were completely fermented, what would be the resulting concentration of the C2H5OH solution? How many moles of CO2 would be formed? How many grams is this? If each mole of CO2 had a volume of 24.5 L, what volume of CO2 is produced?

  28. Aqueous sodium bisulfite gives off sulfur dioxide gas when heated:

    2NaHSO3(aq) → Na2SO3(aq) + H2O(ℓ) + SO2(g)

    If 567 mL of a 1.005 M NaHSO3 solution were heated until all the NaHSO3 had reacted, what would be the resulting concentration of the Na2SO3 solution? How many moles of SO2 would be formed? How many grams of SO2 would be formed? If each mole of SO2 had a volume of 25.78 L, what volume of SO2 would be produced?

  29. What is the concentration of a 1.0 M solution of K+(aq) ions in equivalents/liter?

  30. What is the concentration of a 1.0 M solution of SO42−(aq) ions in equivalents/liter?

  31. A solution having initial concentration of 0.445 M and initial volume of 45.0 mL is diluted to 100.0 mL. What is its final concentration?

  32. A 50.0 mL sample of saltwater that is 3.0% m/v is diluted to 950 mL. What is its final mass/volume percent?

Answers

  1. Solubility is the amount of a solute that can dissolve in a given amount of solute, typically 100 mL. The solubility of solutes varies widely.

  3. 76.5 g

  5. 12.6 g

  7. 26.5%

  9. 0.203%

  11. 52.4 mL

  13. 0.00321 g

  15. 15 ppm

  17. 0.130 M

  19. 0.208 M

  21. 37.6 g

  23. 0.496 L

  25. 0.262 mol; 37.5 g

  27. 1.13 M C2H5OH; 1.13 mol of CO2; 49.7 g of CO2; 27.7 L of CO2

  29. 1.0 Eq/L

  31. 0.200 M