Example: Test for Independence

The chi-square test for independence is used to determine the relationship between two variables of a sample. In this context, independence means that the two factors are not related. Typically in social science research, researchers are interested in finding factors which are related (e.g., education and income, occupation and prestige, age and voting behavior).

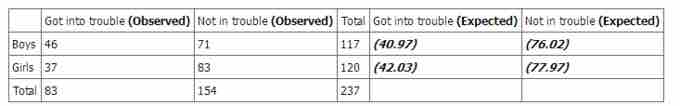
Suppose we want to know whether boys or girls get into trouble more often in school. Below is the table documenting the frequency of boys and girls who got into trouble in school.

Test for Independence

For our example, this table shows the tabulated results of the observed and expected frequencies.

To examine statistically whether boys got in trouble more often in school, we need to establish hypotheses for the question. The null hypothesis is that the two variables are independent. In this particular case, it is that the likelihood of getting in trouble is the same for boys and girls. The alternative hypothesis to be tested is that the likelihood of getting in trouble is not the same for boys and girls.

It is important to keep in mind that the chi-square test for independence only tests whether two variables are independent or not. It cannot address questions of which is greater or less. Using the chi-square test for independence, who gets into more trouble between boys and girls cannot be evaluated directly from the hypothesis.

As with the goodness of fit example seen previously, the key idea of the chi-square test for independence is a comparison of observed and expected values. In the case of tabular data, however, we usually do not know what the distribution should look like (as we did with tossing the coin). Rather, expected values are calculated based on the row and column totals from the table using the following equation:

expected value = (row total x column total) / total for table.

$E=\dfrac{\sigma_r \cdot \sigma_c}{\sigma_t}$

where $\sigma_r$ is the sum over that row, $\sigma_c$ is the sum over that column, and $\sigma_t$ is the sum over the entire table. The expected values (in parentheses, italics and bold) for each cell are also presented in the table above.

With the values in the table, the chi-square statistic can be calculated as follows:

$\begin{aligned}\chi^2 &= \dfrac{(46-40.97)^2}{40.97} + \dfrac{(37-42.03)^ d}{42.03} + d\frac{(71-76.03)^2}{76.03} +\dfrac{(83-77.97)^2}{77.97} \\ &= 1.87\end{aligned}$

In the chi-square test for independence, the degrees of freedom are found as follows:

$df=(r-1)(c-1)$

where $r$ is the number of rows in the table and $c$ is the number of columns in the table. Substituting in the proper values yields:

$df=(2-1)(2-1)=1$

Finally, the value calculated from the formula above is compared with values in the chi-square distribution table. The value returned from the table is $p<0.2$ ($20\%$). Therefore, the null hypothesis is not rejected. Hence, boys are not significantly more likely to get in trouble in school than girls.