Rate of Radioactive Decay

Decay Rate

The decay rate of a radioactive substance is characterized by the following constant quantities:

• The half-life (t_1/2) is the time taken for the activity of a given amount of a radioactive substance to decay to half of its initial value.
• The mean lifetime (τ, "tau") is the average lifetime of a radioactive particle before decay.
• The decay constant (λ, "lambda") is the inverse of the mean lifetime.

Although these are constants, they are associated with statistically random behavior of populations of atoms. Predictions using these constants are less accurate for small number of atoms.

There are also time-variable quantities to consider:

• Total activity (A) is number of decays per unit time of a radioactive sample.
• Number of particles (N) is the total number of particles in the sample.
• Specific activity (SA) number of decays per unit time per amount of substance of the sample at time set to zero (t = 0). "Amount of substance" can be the mass, volume, or moles of the initial sample.

Radioactivity is one very frequent example of exponential decay. The law of radioactive decay describes the statistical behavior of a large number of nuclides, rather than individual ones. In the following relation, the number of nuclides or nuclide population, N, is of course a natural number. Given a sample of a particular radioisotope, the number of decay events, −dN, expected to occur in a small interval of time, dt, is proportional to the number of atoms present N, that is:

$-\frac { dN }{ dt } \propto N$

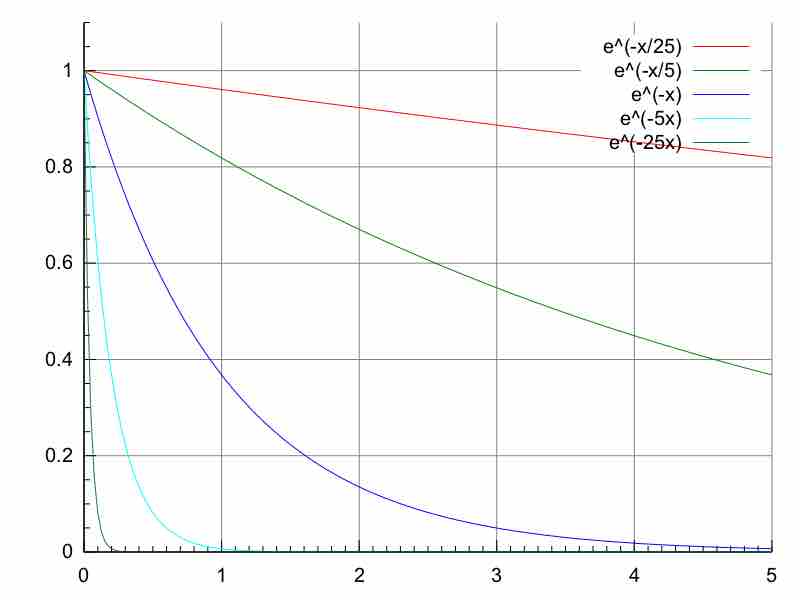
Exponential decay

A quantity undergoing exponential decay. Larger decay constants make the quantity vanish much more rapidly. This plot shows decay for decay constants of 25, 5, 1, 1/5, and 1/25 for x from 0 to 5.

Particular radionuclides decay at different rates, so each has its own decay constant, λ. The expected decay $\frac {-dN}{N}$ is proportional to an increment of time, dt. The constant $\lambda$ is put in place to make the two sides equal:

$-\frac { dN }{ N } =\quad \lambda dt$

The negative sign indicates that N decreases as time increases, as each decay event follows one after another. The solution to this first-order differential equation is the function:

$N={N}_{0}{e}^{-\lambda t}$

Here, N₀ is the value of N at time t = 0.

The SI unit of radioactive activity is the becquerel (Bq), in honor of the scientist Henri Becquerel. One Bq is defined as one transformation, decay, or disintegration per second. Since sensible sizes of radioactive material contain many atoms, a Bq is a tiny measure of activity; amounts giving activities on the order of GBq (gigabecquerel, 1 x 10⁹ decays per second) or TBq (terabecquerel, 1 x 10¹² decays per second) are commonly used.

Another unit of radioactivity is the curie, Ci, which was originally defined as the amount of radium emanation (radon-222) in equilibrium with one gram of pure radium, isotope Ra-226. At present, it is equal, by definition, to the activity of any radionuclide decaying with a disintegration rate of 3.7 × 10¹⁰ Bq, so that 1 curie (Ci) = 3.7 × 10¹⁰ Bq. The use of Ci is currently discouraged by the SI. Low activities are also measured in disintegrations per minute (dpm).

Example

Find the decay rate ($\lambda$) of element X, with a half-life of 2350 years.

To solve, we need to use our equation:

$N={N}_{0}{e}^{-\lambda t}$

Since we are dealing with the half-life we will use values for N and N_o that are equivalent to 0.5.

$5=10{e}^{-\lambda t}$

Now plug in the half-life for the time (t).

$5=10{e}^{-\lambda2350}$

Solve for $\lambda$

$0.5 = e^{-\lambda \times 2350} $

$ln\ 0.5 = -\lambda \times 2350$

$\lambda = 2.95\times 10^{-4} \ year^{-1}$