Integration is an important concept in mathematics and—together with its inverse, differentiation—is one of the two main operations in calculus.
Integration is connected with differentiation through the fundamental theorem of calculus: if
Area
To start off, consider the curve
We ask, "What is the area under the function
As a first approximation, look at the unit square given by the sides
Notice that we are taking a finite sum of many function values of
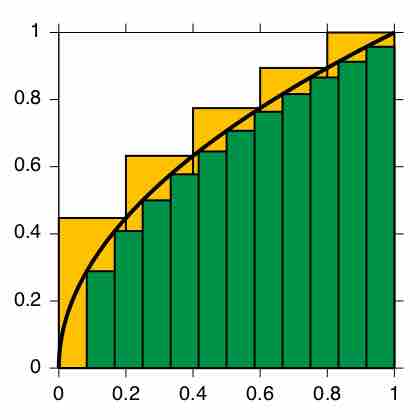
Integral Approximation
Approximations to integral of
Applying the fundamental theorem of calculus to the square root curve,
Distance (Finding arc length by Integrating)
If you know the velocity
However, you can also use integrals to calculate length—for example, the length of an arc described by a function
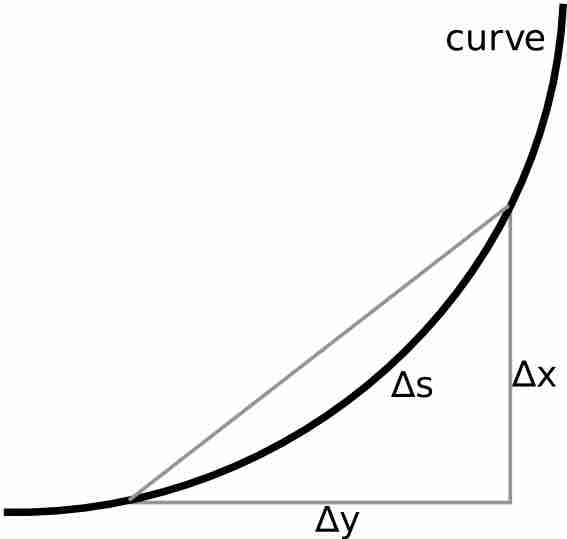
Calculating arc length
For a small piece of curve,
Equivalently, if a curve is defined parametrically by
then its arc length between
Example
For the following curve described by the parameter
the arc length integral for values of
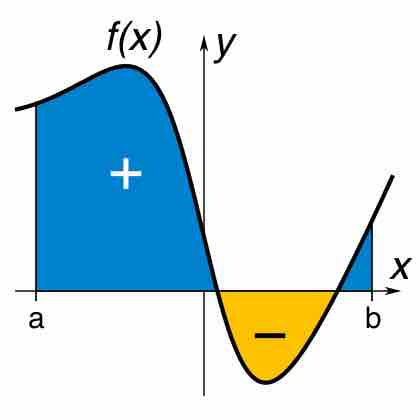
Definite Integral
A definite integral of a function can be represented as the signed area of the region bound by its graph.