Related Rates

One useful application of derivatives is as an aid in the calculation of related rates. What is a related rate? In differential calculus, related rates problems involve finding a rate at which a quantity changes by relating that quantity to other quantities whose rates of change are known. In most cases, the related rate that is being calculated is a derivative with respect to some value. We compute this derivative from a rate at which some other known quantity is changing. Given the rate at which something is changing, we are asked to find the rate at which a value related to the rate we are given is changing.

The rate of change is usually with respect to time. Because science and engineering often relate quantities to each other, the methods of related rates have broad applications in these fields. Because problems involve several variables, differentiation with respect to time or one of the other variables requires application of the chain rule.

Process for solving related rates problems:

1. Write out any relevant formulas and information.

2. Take the derivative of the primary equation with respect to time.

3. Solve for the desired variable.

4. Plug in known information and simplify.

See for a flowchart for solving related rates problems.

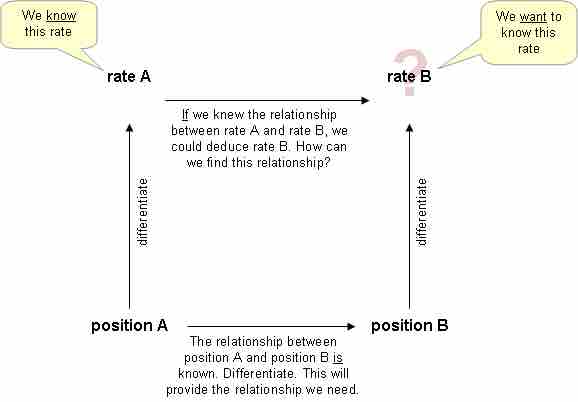
Flow Chart for Related Rate Problem Solving

Related rate problems cab be handled by taking a methodical approach.

Suppose you are given the following situation.

A spherical hot air balloon is being filled with air. The volume is changing at a rate of 2 cubic feet per minute. How is the radius changing with respect to time when the radius is equal to 2 feet?

The relevant formulas and pieces of information are the volume of the balloon, the rate of change of the volume, and the radius.

$\displaystyle{V = \frac{4}{3}\pi r^3}$

$V' = 2$

$r = 2$.

Take the derivative of both sides with respect to time:

$\displaystyle{V' = \frac{4}{3}(3)\pi r^2 r'}$

$\,\, \quad = {4}\pi r^2 r'$

Solve for $r$:

$\displaystyle{r ' = \frac{1}{4\pi r^2}V'}$

Plug in the known information:

$\displaystyle{r' = \frac {1}{16 \pi}2 = \frac {1}{8 \pi}}$ ft/min