Limits and Continuity

A study of limits and continuity in multivariable calculus yields many counter-intuitive results not demonstrated by single-variable functions . For example, there are scalar functions of two variables with points in their domain which give a particular limit when approached along any arbitrary line, yet give a different limit when approached along a parabola. For example, the function $f(x,y) = \frac{x^2y}{x^4+y^2}$ approaches zero along any line through the origin. However, when the origin is approached along a parabola $y = x^2$, it has a limit of $0.5$. Since taking different paths toward the same point yields different values for the limit, the limit does not exist.

Continuity

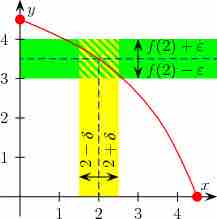
Continuity in single-variable function as shown is rather obvious. However, continuity in multivariable functions yields many counter-intuitive results.

Continuity in each argument does not imply multivariate continuity. For instance, in the case of a real-valued function with two real-valued parameters, $f(x,y)$, continuity of $f$ in $x$ for fixed $y$ and continuity of $f$ in $y$ for fixed $x$ does not imply continuity of $f$. As an example, consider

$f(x,y)= \begin{cases} \displaystyle{\frac{y}{x}}-y & \text{if } 1 \geq x > y \geq 0 \\ \displaystyle{\frac{x}{y}}-x & \text{if } 1 \geq y > x \geq 0 \\ 1-x & \text{if } x=y>0 \\ 0 & \text{else}. \end{cases}$

It is easy to check that all real-valued functions (with one real-valued argument) that are given by $f_y(x)= f(x,y)$ are continuous in $x$ (for any fixed $y$). Similarly, all $f_x$ are continuous as $f$ is symmetric with regards to $x$ and $y$. However, $f$ itself is not continuous as can be seen by considering the sequence $f \left(\frac{1}{n},\frac{1}{n} \right)$ (for natural $n$), which should converge to $\displaystyle{f (0,0) = 0}$ if $f$ is continuous. However, $\lim f \left(\frac{1}{n},\frac{1}{n} \right) = 1$.