Double Integrals Over General Regions

We studied how double integrals can be evaluated over a rectangular region. But there is no reason to limit the domain to a rectangular area. The integral domain can be of any general shape. In this atom, we will study how to formulate such an integral.

This method is applicable to any domain $D$ for which:

• the projection of $D$ onto either the $x$-axis or the $y$-axis is bounded by the two values, $a$ and $b$.
• any line perpendicular to this axis that passes between these two values intersects the domain in an interval whose endpoints are given by the graphs of two functions, $\alpha$ and $\beta$.

$x$-axis: If the domain $D$ is normal with respect to the $x$-axis, and $f:D \to R$ is a continuous function, then $\alpha(x)$  and $\beta(x)$ (defined on the interval $[a, b]$) are the two functions that determine $D$. It follows, then, that:

$\displaystyle{\iint_D f(x,y)\ dx\, dy = \int_a^b dx \int_{ \alpha (x)}^{ \beta (x)} f(x,y)\, dy}$

$y$-axis: If $D$ is normal with respect to the $y$-axis and $f:D \to R$ is a continuous function, then $\alpha(y)$ and $\beta(y)$ (defined on the interval $[a, b]$) are the two functions that determine $D$. It follows, then, that

$\displaystyle{\iint_D f(x,y)\ dx\, dy = \int_a^b dy \int_{\alpha (y)}^{ \beta (y)} f(x,y)\, dx}$

Example

Consider the following region:

$D = \{ (x,y) \in \mathbf{R}^2 \ : \ x \ge 0, y \le 1, y \ge x^2 \}$

Calculate $\iint_D (x+y) \, dx \, dy$. This domain is normal with respect to both the $x$- and $y$-axes. To apply the formulae, you must first find the functions that determine $D$ and the intervals over which these are defined. In this case the two functions are $\alpha (x) = x^2$ and $\beta (x) = 1$, while the interval is given by the intersections of the functions with $x=0$, so the interval is $[a,b] = [0,1]$ (normality has been chosen with respect to the $x$-axis for a better visual understanding).

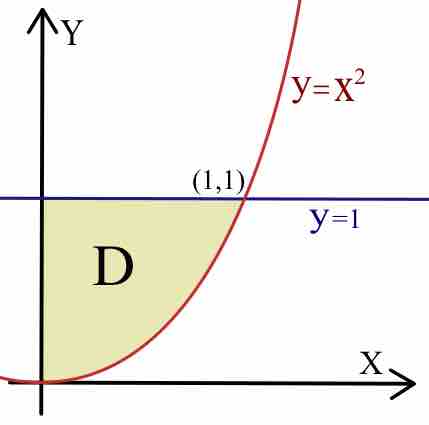
Double Integral

Double integral over the normal region $D$ shown in the example.

It is now possible to apply the formula:

$\begin{aligned}\iint_D (x+y) \, dx \, dy &= \int_0^1 dx \int_{x^2}^1 (x+y) \, dy \\ &= \int_0^1 dx \ \left[xy + \frac{y^2}{2} \right]^1_{x^2}\end{aligned}$

(At first the second integral is calculated considering $x$ as a constant). The remaining operations consist of applying the basic techniques of integration: 

$\begin{aligned}\int_0^1 \left[xy + \frac{y^2}{2}\right]^1_{x^2} \, dx &= \int_0^1 \left(x + \frac{1}{2} - x^3 - \frac{x^4}{2} \right) dx \\ &= \frac{13}{20}\end{aligned}$