Higher degree polynomial equations can be very difficult to solve. In some special situations, however, they can be made more manageable by reducing their exponents via substitution.  If a substitution can be made such that the higher order polynomial takes the form of a quadratic, any method for solving a quadratic equation can be applied.   

For example, if a quartic equation is biquadratic—that is, it includes no terms of an odd-degree— there is a quick way to find the zeroes of the quartic function by reducing it into a quadratic form.  Consider a quadratic function with no odd-degree terms which has the form:

$0=ax^4+bx^2+c$

If we let an arbitrary variable $p$ equal $x^2$, this can be reduced to an equation of a lower degree:

$0=ap^2+bp+c$

With substitution, we were able to reduce a higher order polynomial into a quadratic equation.  It can now be solved with any of a number of methods (via graphing, factoring, completing the square, or by using the quadratic formula).

Once values of $p$ are found, each positive value of the temporary variable $p$ can be used to find two values of $x$ such that:

$x=\sqrt p$

As with every square root, the root of $p$ will have two values, one positive and one negative.  It is important to realize that the same kind of substitution can be done for any equation in quadratic form, not just quartics. 

Example

As an example, consider the equation:

$0=x^4-12x^2+20$

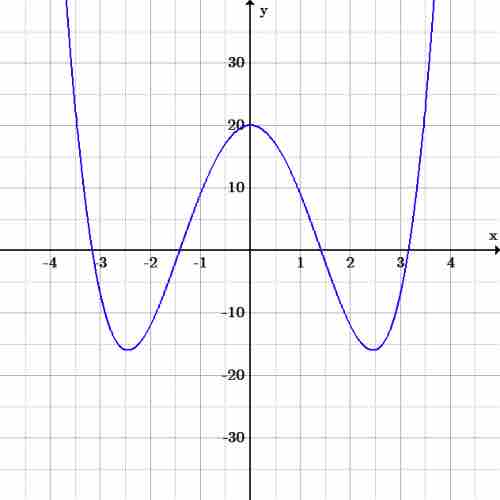
Quartic graph

Graph of the function $f(x) = x^4-12x^2+20$.

We can substitute the arbitrary variable $p$ in place of $x^2$:

$0=p^2-12p+20$

This equation is now solvable for $p$ using the quadratic formula:

$p=\dfrac {12 \pm \sqrt {(-12)^2-4\cdot 1\cdot 20}}{2\cdot 1}$

Simplifying this, we find $p$ equals 2 or 10.

Knowing that $p=x^2$, we can use each value of $p$ to solve for two values of $x$:

$x=\pm \sqrt 2$ and $x= \pm \sqrt 10$

A similar procedure can be used to solve higher-order equations. The requirement is that there are two terms of $x$ such that the ratio of the highest exponent of $x$ to the lower is $2:1$.