A hyperbola is one of the four conic sections. All hyperbolas share common features, and it is possible to determine the specifics of any hyperbola from the equation that defines it.

Standard Form

Diagram of a hyperbola

All hyperbolas share common features.

If the foci lie on the $x$-axis, the standard form of a hyperbola is:

$\displaystyle{\frac{(x-h)^2}{a^2} - \frac{(y-k)^2}{b^2} = 1}$

If the foci lie on the $y$-axis, the standard form is: 

$\displaystyle{\frac{(y-k)^2}{a^2} - \frac{(x-h)^2}{b^2} = 1}$

We will use the $x$-axis hyperbola to demonstrate how to determine the features of a hyperbola, so that $a$ is associated with $x$-coordinates and $b$ is associated with $y$-coordinates. For a $y$-axis hyperbola, the associations are reversed.

Center

The center has coordinates $(h,k)$.

Vertices

The vertices have coordinates $(h + a,k)$ and $(h-a,k)$. The line connecting the vertices is called the transverse axis.

Co-Vertices

The co-vertices correspond to $b$, the "minor semi-axis length", and have coordinates $(h,k+b)$ and $(h,k-b)$.

Asymptotes

The major and minor axes $a$ and $b$, as the vertices and co-vertices, describe a rectangle that shares the same center as the hyperbola, and has dimensions $2a \times 2b$. The asymptotes of the hyperbola are straight lines that are the diagonals of this rectangle. We can therefore use the corners of the rectangle to define the equation of these lines:

$\displaystyle{y = \pm \frac{a}{b}(x - h) + k}$

The rectangle itself is also useful for drawing the hyperbola graph by hand, as it contains the vertices. When drawing the hyperbola, draw the rectangle first. Then draw in the asymptotes as extended lines that are also the diagonals of the rectangle. Finally, draw the curve of the hyperbola by following the asymptote inwards, curving in to touch the vertex on the rectangle, and then following the other asymptote out. Repeat for the other branch.

Focal Points

The foci have coordinates $(h+c, k)$ and $(h-c,k)$. The value of $c$ is found with this relation: 

$c^2 = a^2 + b^2$

Rectangular Hyperbola

Rectangular hyperbolas, defined by 

$\left(x-h\right)\left(y-k\right) = m$

for some constant $m$, are much simpler to analyze than standard form hyperbolas.

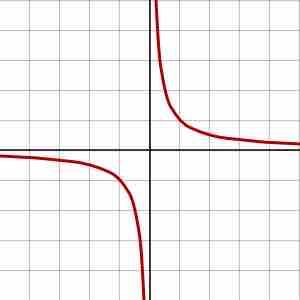
Rectangular hyperbola

This rectangular hyperbola has its center at the origin, and is also the graph of the function $\displaystyle{f(x) = \frac{1}{x}}$.

Center

The center of a rectangular hyperbola has coordinates $(h,k)$.

Vertices and Co-Vertices

The rectangular hyperbola is highly symmetric. Both its major and minor axis values are equal, so that $a = b = \sqrt{2m}$. The vertices have coordinates $(h+\sqrt{2m},k+\sqrt{2m})$ and $(h-\sqrt{2m},k-\sqrt{2m})$.

The co-vertices have coordinates $(h-\sqrt{2m},k+\sqrt{2m})$ and $(h+\sqrt{2m},k-\sqrt{2m})$.

Asymptotes

The asymptotes of a rectangular hyperbola are the $x$- and $y$-axes.

Focal Points

We can use $c^2 = a^2 + b^2$ as before. With $a = b = \sqrt{2m}$, we find that $c = \pm 2\sqrt{m}$. Therefore the focal points are located at $(h+2\sqrt{m},k+2\sqrt{m})$ and $(h-2\sqrt{m},k-2\sqrt{m})$.