Binomial Theorem
The binomial theorem is an algebraic method of expanding a binomial expression. Essentially, it demonstrates what happens when you multiply a binomial by itself (as many times as you want). For example, consider the expression
According to the theorem, it is possible to expand the power
For example, consider the following expansion:
Any coefficient
According to the binomial theorem, it is possible to expand any power of
where each value
A significant amount of time may be required to apply the binomial theorem and perform all of the calculations in the above formula, particularly for high values of
Pascal's Triangle
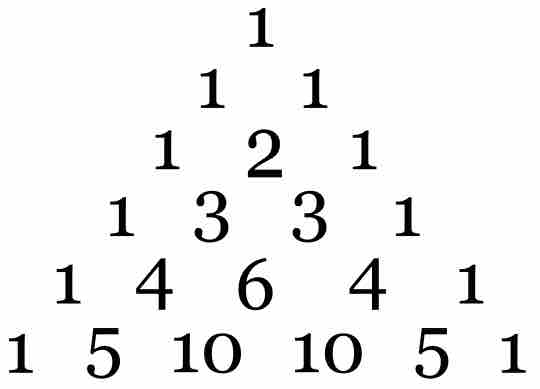
Pascal's triangle is an alternative way of determining the coefficients that arise in binomial expansions, using a diagram rather than algebraic methods. For a binomial expansion with a relatively small exponent, this can be a straightforward way to determine the coefficients.
In the diagram below, notice that each number in the triangle is the sum of the two directly above it. This pattern continues indefinitely.
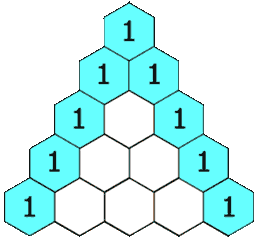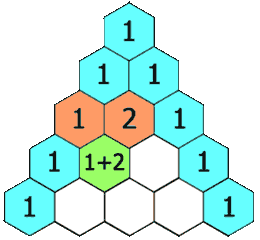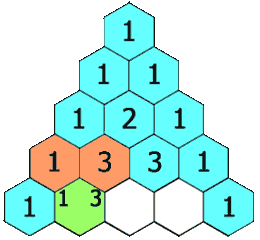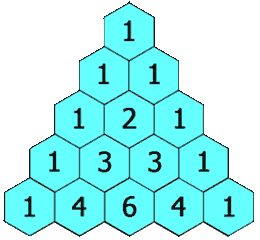
Pascal's Triangle
Each number in the triangle is the sum of the two directly above it.
The rows of Pascal's triangle are numbered, starting with row
To understand how this pattern applies to the binomial formula, consider the expansion:
Notice the coefficients are the numbers in row two of Pascal's triangle:
Where the coefficients
Notice that the entire right diagonal of Pascal's triangle corresponds to the coefficient of
Example: Find the expansion of $(x+y)^5$ using Pascal's triangle
Notice that
Pascal's Triangle
Pascal's triangle with 5 rows.
Recall that the binomial expansion of
It can be observed in the triangle that row