If the above player doesn't work, try this direct link.

If you're ever picking out a telescope to see your favorite planet, make sure you do the math first! Learn how to solve the logarithmic equation that will get you the size telescope you want.

Magnitude

I'm not going to lie; I'm kind of a nerd, and I got really into science fiction when I was just a little kid. Maybe it helps that I was named after Luke Skywalker, but, either way, starting in elementary school, I couldn't stop thinking about the Force, spaceships and our solar system. My favorite planet was Pluto (although, I suppose it's more of an ex-planet now), but either way I wanted to get a telescope so I could see it. My parents and I found a really cheap one at a store close to my house, but much to my dismay, when I got home and tried it out it wasn't nearly good enough.

As it turns out, the telescope I got could only see stars with an apparent magnitude of 8, but what I needed was one that could see stars with an apparent magnitude of 14. Too bad I wasn't a math teacher yet and didn't know the equation magnitude equals 5 times the log of diameter plus 2 (or M = 5 log D + 2). This is the equation that tells you what magnitude star you can see with a lens that has a diameter of D millimeters. If I had been a math teacher, I would have known that I could substitute 14 in for M and then solve the equation for D. The resulting answer would tell you how big your lens would need to be in order to see stars with an apparent magnitude of 14.

So, how big of a telescope did I actually need? Well, it's again our job to use inverse operations to get the D by itself in this equation. We need to start peeling the layers off of this equation one at a time in reverse order, starting with the outermost step in this equation, which is the + 2. I can undo addition with subtraction, and subtracting 2 from both sides gives me 12 = 5 log D. Now, the outermost layer is 5 times. I undo multiplication with division, dividing both sides by 5, and I get 2.4 = log D. Now, I need to undo a log, and this is where the new skill comes into play. We do know that the inverse operation to a log is an exponential, so I need to use an exponential to undo this log. I need to pick my exponential to be the same type of log that this is - meaning I need to be taking the same base log and exponential in order to undo them. This log is blank, which means there's an imaginary base 10 in there, so I need to do the exponential base 10 on both sides.

This is a little strange; we haven't actually done this before. A common mistake I see is people try to raise the log to the 10th power, but that's not exactly what we want. An exponential has the variable in the exponent, so the 10 shouldn't be the exponent; the log D should be the exponent. What I do is write 10 to the bottom left of the log, and that is my base, so I get 10^(log D). Whatever I do to one side, I have to do to the other side, so I have 10^(2.4) = 10^(log D). The 10^(log base 10) undoes, because the exponential and log are inverse operations, so I just get 10^(2.4) = D. If I plug 10^(2.4) into my calculator, I get ~251. That means that I needed a telescope that had a lens with a diameter of 251 millimeters. That's almost 10 inches, which is a pretty big telescope! Maybe it's for the best that I didn't know this equation, because if I would've asked my parents for something that big I would've been rejected for sure.

Product Property

We can use similar skills to solve even harder logarithmic equations, like log base 2(x) + log base 2(5) = 10. This is more difficult than our telescope example because there are two logs instead of one. This means we'll get a chance to practice our property skills as well as our solving skills all in one problem! Before I can solve this equation like we did before, I need to use the product property to combine the two logs into one. I picked the product property because I saw two logs separated by addition. When I see that, the product property tells me that I can condense them into one log where the two things on the inside are now multiplied. So, instead of log base 2(x) + log base 2(5), I combine them into one, multiply 5 and x and I get log2(5x) = 10.

Now that it is written as one log, I can continue to do this problem and solve it exactly like I did the last one. The 5 and the x are both on the inside of the log, so the next thing to undo, the outermost layer, is the log itself. We just learned that you undo logs with exponentials by exponentiating. This time, the base of my log is 2, which means I have to use the same base in my exponential, so I put in 2^(log base 2(5x)) = 2^10. That undoes the log on the left, I just get 5x, and on the right 2^10 = 1,024. I quickly undo multiplication with division, get the x by itself and find that x must be equal to 204.8.

Exponentiating

We can even solve logarithmic equations that have logs on both sides of the equals sign, like this: log base 3(4x) = log base 3(2x + 8). Because we're being asked to solve, the goal is still to figure out what x must equal for the equation to be true. That means undoing. Luckily, both logs have the same base, 3, and the logs are the outermost step on both sides of the equation. There's no plus 3 on the outside, or divided by 2 or times 4; on both sides, the log is the outermost thing. Another way of saying that is everything that is on either side of the equation is on the inside of the log. Because of this, we can simply exponentiate both sides with the same base, 3, and the logs undo on both sides. We are just left with a nice, easy linear equation, 4x = 2x + 8. Now it's simply a matter of undoing what we know with subtraction, then with division, to find that x = 4.

You will never come across a problem wherein you will need to exponentiate for logs with different bases

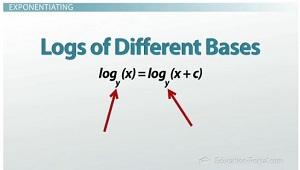Unlike exponential equations, I have never seen an example problem like this that has logs with different bases, so don't worry about having to change the base of the logs before exponentiating for problems like this. That will never be asked of you!

Lesson Summary

To review, we can undo logarithms by exponentiating. Just like we can multiply both sides of the equation by 2 to undo division, we can exponentiate both sides by 2, or by any number for that matter, turning x = y into b^x = b^y. If you are solving an equation with two logs on the same side of the equals sign, you must first use what you know about logarithmic properties to combine the logs into one before you can undo anything. Lastly, if you are solving an equation with logs on either side of the equals sign, as long as they have the same base, you can simply exponentiate to cancel both of them out.