Exponential Growth vs. Decay
After you watch the video and know the material, click HERE for the quiz.
How is it that it only takes four years for our computer to go from top-of-the-line to almost worthless? Well, it has something to do with what's called exponential decay, which we'll learn about here!
Exponential Growth
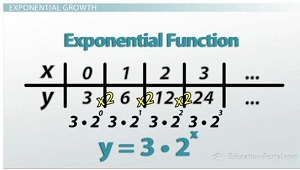 |
We know that an exponential function is any pattern of numbers that is continuously multiplied by something - for example 3, 6 (3x2), 12 (3x4), 24 (3x8). This is an exponential function because I start with a number 3 and continuously multiply by 2. We can represent this with an equation by saying that y=3x2^x, because the pattern starts at 3 and then is multiplied by 2 each step of the way. If you substitute in x values starting at 0 and remembering that 0 exponents equal 1, you'll find that we get this exact same pattern. This example is what is called exponential growth because the numbers are growing exponentially, but there is another type of exponential function whose entries get smaller instead of getting bigger, exponential decay.
Exponential Decay
Exponential decay still follows the same rule of repeated multiplication, only the number we multiply by has to be smaller than one. What this ends up looking like is something that appears to be repeated division. Let's take a look at an example that most of us can relate to, our computer becoming worthless way faster than we had expected.
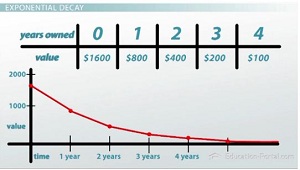 |
Say you buy a pretty nice new computer for $1,600, but after you've owned it for a year, it's already worth only 1/2 as much as it used to be. That makes it worth $800 after 1 year, and if this pattern continues it would be worth $400 after 2 years, $200 after 3, and only $100 after a short 4 years. The price of your computer is 'decaying' (or getting smaller) each year by a factor of 1/2. It kind of looks like division by 2, but remember that division by 2 is the same as multiplication by 1/2. That means that because your pattern begins at 1,600 and becomes repeatedly multiplied by 1/2, the exponential decay equation for this example would be y=1,600x1/2^x.
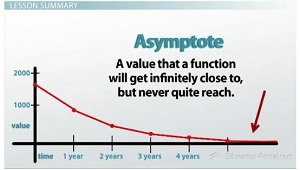
These exponential functions get really small, really fast. If we assume this pattern continues, your computer would only be worth $1.50 after ten years! But it's important to note that no matter how long you keep your computer, it will never be worth $0. It might seem like it, and it might be worth pretty close to zero, but multiplying by 1/2 over and over can only make a number that's really really small. Maybe you'll end up with 0.0000000000000000001, but it will always have that little 1 on the end. If I divide this by 2, which is smaller, now it's 0.00000000000000000005. There's still a number down there; no matter how many times I divide by two, it's still going to be a tiny bit bigger than zero. This means that on our graph our line is going to get closer and closer and closer to the x-axis but it'll never quite get there. This is a math concept that comes up pretty often and is called an asymptote. In this case we have an asymptote at y=0 because that is the line (which is basically the x-axis) that our graph will get infinitely close to but never quite touch.
 |
Growth vs. Decay
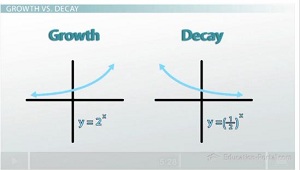
Now that we're familiar with both exponential growth and decay, it's important to take a look at their graphs and notice a very hopefully obvious similarity. Here I have y=2^x, which is growth because I multiply repeatedly by 2, which makes numbers bigger, and here I have y=1/2^x, which is decay because I have 1/2 being repeatedly multiplied, which makes things smaller. Looking at their graphs, you notice that they're almost the same except they've been flip-flopped or reflected around the y-axis. That means that the right-hand side of my decay graph is exactly the same as the left-hand side of my growth graph. This is true because I can plug negative values in for x in my growth graph, and when I plug in for example -1 negative exponents give me fractions, which means I have to take 1 and put it over 2^1, and that gives me 1/2, which is exactly what I do if I do 1/2^1 over here in my decay function. So the point at +1 in the decay graph is exactly the same as the point at -1 in the growth graph. That means that they both have an asymptote at y=0, it's just that the growth graph gets infinitely close to zero as you go into the negatives, and the decay graph gets infinitely close to zero as you go into the positives.
 |
Determining a Starting Value
Now that we're familiar with both exponential growth and exponential decay, we can begin to solve more complex examples, ones that, for example, ask us to determine the starting value of an exponential function when we are only given two random entries.
Let's say I told you that after owning my car for three years it was worth $7,168 and then three years later it was down to $3,670. If we assume that it was decreasing by the same percent each year, which means it's an exponential decay function, that means that I took $7,168 and multiplied it by something three times and ended up with $3,670. I can turn this into an equation simply by saying $7,168 times b times b times b (where b is my base) equals $3,670. Condensing the Bs as b^3 gives me 7168b^3=3670, and now I simply have to undo everything that's been done to the b to figure out what my base or multiplying factor is. I divide both sides by 7168, which leaves you with b^3 = 0.5119977…. I undo a third power with a third root and I find that b must be 0.79999999…, which we're going to round to 0.8. That means that the base of my exponential is 0.8, which means that every year I own my car it retains 80% of its value.
Now that I know that, I can simply work my way backwards to figure out how much it must have cost the very day I bought it. Now instead of multiplying by 0.8, to go backwards I'm going to divide. First I divide by 0.8, then I divide by 0.8 again, I divide by 0.8 one last time and it turns out that my car must have initially cost me $14,000.
Lesson Summary
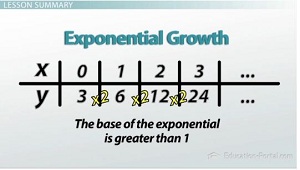 |
- Exponential functions are patterns that get continuously multiplied by some number.
- It's exponential growth when the base of our exponential is bigger than one, which means those numbers get bigger.
- It's exponential decay when the base of our exponential is in between 1 and 0 and those numbers get smaller.
- An asymptote is a value that a function will get infinitely close to, but never quite reach.