How to Factor Quadratic Equations: FOIL in Reverse
After you watch the video and know the material, click HERE for the quiz.
So, you know how to multiply binomials with the FOIL method, but can you do it backwards? That's exactly what factoring is, and it can be pretty tricky. Check out this lesson to learn a method that will allow you to factor quadratic trinomials with a leading coefficient of 1.
Third Grade Review
Probably the most fundamental concept of algebra is the use of a variable (like x) to represent any number we want. That means that a lot of the stuff we do in an algebra class is actually stuff you learn in elementary school; we just use variables instead of specific numbers. I've found that one of the best ways to teach algebra is to first review the skill with specific numbers and then apply that same skill to our new algebraic problem.
 |
What is 3 * 5? I think I need my calculator for this one. Ah! 15. Okay. Another third grade question, but this one might actually stump you because you'll need to remember some vocabulary: factor 15. So, the question really becomes, what does it mean to factor something? Turns out that it simply means to split that number up into what you can multiply to get it. So when I factor 15, I just turn it into 3 * 5. That means factoring is just doing multiplication in reverse. A little more vocab before we move on here. In the statement 3 * 5 = 15, 3 and 5 are called the factors of 15, while the answer to a multiplication problem is called the product.
 |
Multiplying Binomials
So, back to algebra class. Let's do the same set of problems that we just did - but with variables this time around. So, instead of 3 * 5, how about (x + 3)(x + 5)?
There are a lot of different ways to multiply binomials just like this, but the most well-known way is the FOIL method. FOIL is an acronym that helps us remember to multiply the first terms, the outer terms, inner terms and finally the last terms. Once we've done that, we can combine our like terms to get our answer, x^2 + 8x + 15.
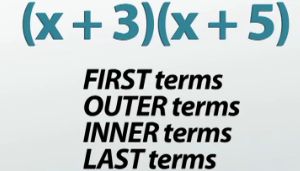 |
Following the same order of questions from the third grade review, the next thing I'll ask is this: factor x^2 + 8x + 15. We now know that this means to break up our number into the two things that I can multiply to get it (its two factors). Since we just did that, I know that the answer is going to be (x + 3)(x + 5), but what if we hadn't just done it? How would we know exactly how to break this trinomial up into its two separate binomials?
This question is what factoring quadratic expressions is all about, and it can be pretty tricky. But if we look closely at the example we just did, try to find some patterns and then generalize those patterns, we'll be able to come up with a method that will help you factor most quadratic trinomials you'll encounter.
Factoring Quadratic Expressions
Let's look closely at that last problem we just did. We said we could factor x^2 + 8x + 15 as (x + 3)(x + 5). What do the numbers from the trinomial (the product) have to do with the numbers from the two binomials (the factors)? Two things, actually!
3 + 5 gives us 8, which was the coefficient from the middle term from the trinomial. And 3 * 5 gives us 15, the constant on the end of the trinomial. This means that if you can find two numbers that add to the middle term of your trinomial and multiply to the constant on the end, those are going to be the two numbers in your factored expression.
Let's see if we can apply this idea to a different problem; maybe factor x^2 + 11x + 24. So, for our method to work, we need to find two numbers that add up to the middle coefficient (11) and that will also multiply to the constant on the end (24). Sometimes the answer is going to be obvious, but sometimes it will be hard to see. When it's hard to see right away, I like to write out all the different ways we can multiply to get the constant on the end and then see which one of those will add up to the middle. Let's do that here to practice. We'll always be able to do 1 times the number. In this case, (1 * 24). 24 is even, so 2 goes into it as well, so (2 x 12), I think 3, (3 x 8) and 4, (4 x 6), and that's it. I could do the other ones, (6 * 4), (0 * 3), but those are just the same just backwards, so those don't matter. So we have these four options - which ones add up to 11? Hey, 3 and 8!
That means that x^2 + 11x + 24 factors into (x + 3)(x + 8). If you'd like to check your answer, you can quickly multiply it out and make sure it ends up back where we started! If we do that here, sure enough, we're all good!
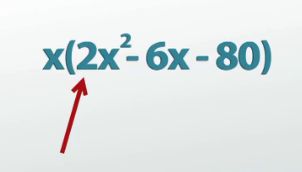 |
One last example: factor 2x^3 - 6x^2 - 80x. This one looks quite a bit different. x^3? We don't know how to do that. Sometimes, there are ways (maybe you'll learn those in a different class), but if you see that in this class, there's probably something tricky going on. Sure enough, looking at each term in our trinomial here, there's an x in it. Whether it's an x^3, an x^2 or just a plain old x, there's an x in each term, which means I can divide it out (or factor it out) of each term by doing the distributive property backwards.
When I pull that x out of each term, I can now write it in front and leave what I'm left with after I divide it out on the inside of the parentheses. So, I no longer have 2x^3, but I have 2x^2; instead of 6x^2, I have 6x; and instead of 80x, I just have plain old 80. But now there's another different thing going on. There's a 2 in front of my x^2. In a later lesson, we'll get to examples where you're going to have to deal with that 2, but it's also worth checking to see if I can do the same thing. Can I divide that 2 out of each term, just like I divided the xs out? Sure enough, again, we're going to be able to do that because 2, 6 and 80 are all even numbers and therefore divisible by 2.
 |
Doing the distributive property backward and factoring a 2 out of each, bringing the 2 in front and then putting what I'm left with in parentheses, gives me 2x(x^2 - 3x - 40). Now, even though I have a 2x out front, what's inside the parentheses is something we know how to do; it's a trinomial, it's got 1x^2 and I can try to use my pattern.
That pattern says I need two numbers that add up to -3 and multiply to -40. So we actually have some negatives this time, but that won't actually change too much. Again, if the two numbers aren't obvious to me right away, I can write out all the factors of 40, like so. But since we need to multiply to -40, one of the numbers is going to have to be positive and one's going to have to be negative. So now we're looking for a pair of numbers that have a difference of 3, not a sum. Which set of two can I subtract to get -3? It looks like 5 - 8 would do the trick. Because I'm doing a +5 minus an 8, the two numbers that are going to work are +5 and -8.
That means I factor the x^2 - 3x - 40 part as (x + 5)(x - 8), and the 2x is still hanging out in the front, so we get 2x(x + 5) (x - 8) as the answer.
The patterns we've talked about here are going to help you factor quadratic expressions that have a leading coefficient of 1. Like I mentioned before, sometimes we're going to have a 2 or 3 in front of the x ^2s. For those, you're going to have to use a different method. To find out about that method, you'll have to check out a future lesson.
Lesson Summary
When you are asked to factor something, it's your job to break that number up into its factors, the numbers that multiply to your original number. This means that factoring is basically multiplication in reverse.
If the quadratic expression you're factoring has only 1x^2 in front, you only need to find the pair of numbers that fit the following pattern. They have to add up to the middle coefficient, the number in front of the xs. That pair of numbers needs to multiply to the constant, the number on the end.