Practice Problems for Logarithmic Properties - Quiz
Choose your answer and write the correct one down. Then click HERE for the answers to this quiz.
NOTE: The transcript from the video is listed below the quiz for your reference.
1.
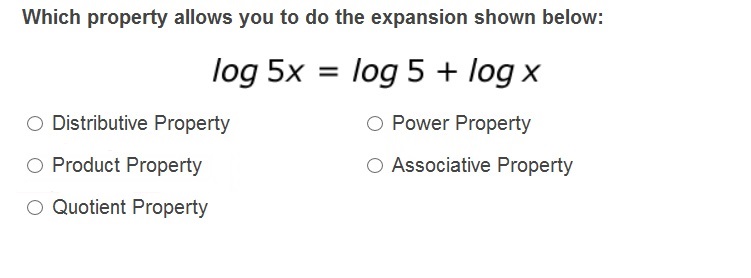
2.
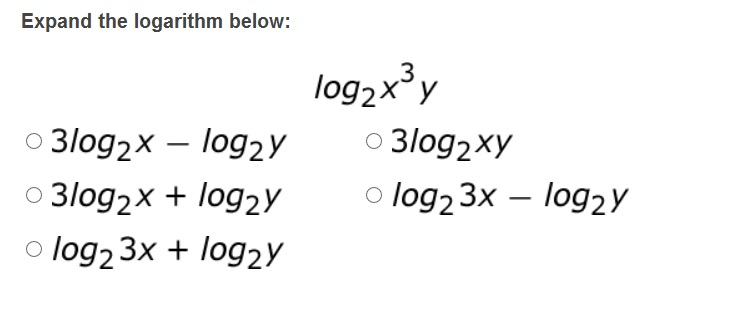
3.
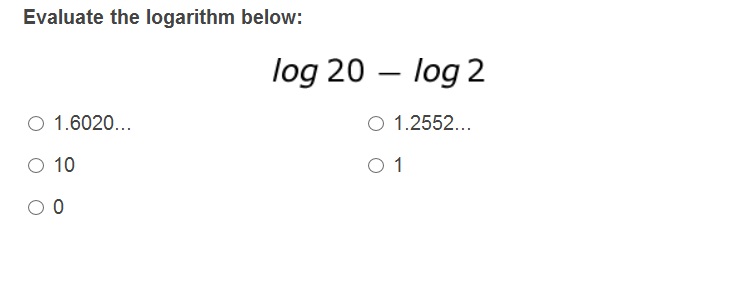
4.

5.

As you get further and further into mathematics, logarithms will appear more and more. It's the point of this lesson to get you used to dealing with them and able to know what to do when you see a whole line of paper full of them!
The Logarithmic Properties
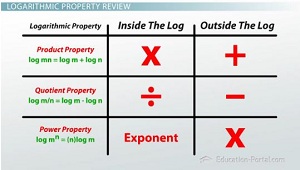 |
When we learned about the logarithmic properties, we were able to boil all the useful information from the three major properties down into this table. The product property told us that multiplication on the inside of a log can be turned into addition on the outside. The quotient property said that division on the inside could be turned into subtraction on the outside. The power property said that an exponent on the inside of the log could be turned into multiplication on the outside.
Expanding Logs
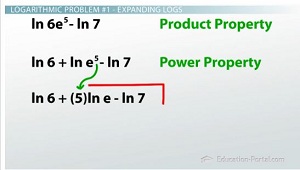
These properties come in handy when we're doing a problem and get to a step with a log that looks like this: ln(6e^5/7).
 |
Logs are confusing enough when they've only got one number on the inside, but ones like this can be daunting. Luckily, we have the properties to break this complex log into smaller, more manageable pieces. Because order of operations would tell us that dividing by 7 on the inside of this expression would be the last thing we'd do in this expression, it's going to be the first thing we're going to undo with the properties.
Since it's being divided by 7, we'll use the quotient property to start expanding this expression. It tells us that the division we see on the inside can be turned into subtraction on the outside. This means we get the natural log of the numerator minus the natural log of the denominator: ln(6e^5/7) = ln(6e^5) - ln(7).
Looking now at ln(7), I know I have nothing left to do with this because that's just the natural log of a single number. There are no other operations going on in the inside of that natural log. However, that first term ln(6e^5) still has stuff going on in there, so I still have work to do.
We can next use the product property to remove the most outside thing, the last thing order of operations would do, which is the 6 times e^5. Using that product property, we now end up with ln(6) + ln(e^5) for that first term. That product property just turned multiplication on the inside into addition on the outside. Now we have: ln(6e^5/7) = ln(6) + ln(e^5) - ln(7).
Lastly, we can use another property on the middle term. We can use the power property to change the exponent on the inside to multiplication on the outside. We now have our fully expanded expression: ln(6e^5/7) = ln(6) + 5ln(e) - ln(7).
This expanded expression might be a little bit easier to look at because I can now think about evaluating each term. I can evaluate ln(6) and ln(e) and so on to even get an estimate for this expression. Back in the beginning, I had no idea, since it was too complex. The fully expanded expression can help us get a better sense of what it is.
Condensing Logs
It's also nice to be able to do the opposite: condense a long list of logs into one single one. While it's nice to see each simple piece when we are evaluating it and trying to estimate it, if we have to write it over and over again, it's not fun writing out each step. We'd rather 'pack it up' into one log while we're using it and then 'un-pack' it when we actually need it.
Take this for example: 2(log(6) + log(x)) - log(12). Writing this over and over again on each line of my paper would get really annoying, really quickly. So let's use our properties and go backwards with this expression this time to condense it into one logarithm.
This time, we're going to do order of operations in a normal way. I first look inside my parentheses, and I see there's addition between logs. I want to condense this addition on the outside of the logs, which means using the product property. The product property says I can take addition on the outside and turn it into multiplication on the inside. Thus, I get this slightly smaller expression: 2(log(6x)) - log(12).
Next, I want to use the power property. The 2 in front of the log(6x) means multiplication on the outside, which our table says can be changed to an exponent on the inside, using the power property. Because the 6 and the x are both on the inside of the same log, the exponent is on both of them. I can then distribute that exponent to get log(36x^2) - log(12).
Lastly, I can use the quotient property to turn subtraction on the outside into division on the inside. We end up with log(36x^2/12). That can actually be simplified to be log(3x^2). Now, we have our fully condensed expression. Not only did condensing this log make it easier to write, but it also made some of the numbers cancel out!
 |
Evaluating Logs
Finally, we can even use the properties to help us evaluate some logarithms as well. Take this example: log base 3 of the square root of 27 minus log base 5 of the square root of 5.
While it is true that these logs can be evaluated without calculators, it isn't very clear how we would do that by looking at this problem right now. Let's try 'un-packing' the logs a little bit. Maybe if we break them into smaller pieces, we'll have a better idea of what these things equal.
We first have to remember that square roots written in radical form can be switched to rational exponent form. This basically means that the square root of a number is the same thing as that number to the ½ power. Remembering this allows us to rewrite our first expression as log base 3 of 27 to the ½ power minus the log base 5 of 5 to the ½ power.
This already looks nicer because I know what to do with exponents on logs. I can use the power property to bring the exponents down in front of the logs and have it be multiplication. Now, when I see log base 3 of 27 without the square root or the power, I can think what does log 3 of 27 equal? Well, 3^3 = 27, so log base 3 of 27 is just 3. If I look over at log base 5 of 5, what exponent turns 5 into 5? Well, that would be a power of 1, which means the log base 5 of 5 is just 1. Now, I have an expression that I can just multiply and subtract to get out: 1/2(3) - 1/2(1) = 1.5 - .5 = 1.
Lesson Summary
 |
Using the properties to expand logarithms can be helpful when we're trying to evaluate the log or simply when it looks to complicated to do anything with it. However, condensing them back into a single log can make it much easier to write down and also will sometimes help cancel out some values