Using this lesson, you can get practice evaluating logarithms, as well as learn some of the shortcuts behind writing and estimating them. You can also learn how to use your calculator to evaluate logarithms, and learn about a concept called the change of base theory.

Quick Review of Logarithmic Form

So in our last lesson, we learned that logarithms are the inverse operation of exponentials, and they give us a way to solve exponential equations. When we were trying to figure out how far into the future it will be before there are 200 billion cell phones, we were stuck with the equation 2^x=34,359,738,368. That's where the log came in, because the answer to our question, 'what exponent turns 2 into 34, 359,738,368?' is exactly what log2(34,359,738,368) is. We decided that the log2 of 34,359,738,368 was 35, because 2^35=34,359,738,368. What you see are two different ways of writing the exact same thing; I could write this equation in logarithmic form, which is logb(y)=x, or I could write it in exponential form, which is b^x=y. And both of these forms are just two different ways of saying the same thing.

Both forms are two different ways of saying the same thing.

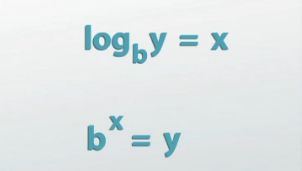Now, a lot of students get freaked out when they see a log, because it's new, and they're not used to it, and think that it means to multiply log with 5, or to raise 5 to the 25th power… but neither of these are actually true. The little 5 beneath the log is a 'subscript' and goes with the log. Every log is going to have one of these, and it tells us which type of log we are doing.

Problem #1

In this example, we are taking the log base 5 of 25 - so we're taking the log5 of this number. Therefore, we are being asked, 5 to what power equals 25?. The answer is, of course, 2. So the answer to our log is simply 2, because 5^2=25.

Problem #2

Let's try another one, log3(27)=? The answer is the exponent that will turn the base of the log into the number we are taking the log of. Therefore, the answer is 3, because 3^3=27. Now, writing out the exponential form for these problems can be a way of proving your answer, but it's not the answer itself. The answer is just the number we get, and the exponential form is a way of saying why that answer is the answer it is, or a way of checking your work.

Problem #3

What exponent turns 9 into 1?

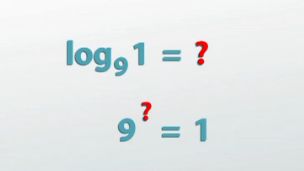Another example: How about log9(1)=? What exponent turns 9 into 1? Well, you have to remember the 0 exponent property - remember that anything to the 0 power gives you 1, so 0 is the exponent that turns 9 into 1, so the answer to this one is just 0.

Problem #4

Negative exponents give us fractions.

Or, finally, what about log2(1/8)=? For this one you're going to have to remember the negative exponent property. Negative exponents give us fractions, and because I have to turn 2 into 8, that is the 3rd power, because 2*2*2=8, so 2^-3=1/8, and therefore the answer to log2(1/8) is, simply, -3.

Log Base 10 and Natural Log

These logs are used most often.

So as you can see, there are all types of logs. log2, log8, log55, logPi… anything we want. But there are two logs that are used the most often: log10 and loge. Now, just like we use pi to mean 3.14159 and on and on, we use the letter e to mean 2.718281 and on and on. This one is called the natural base. It won't really become useful until calculus, so for now, we're just going to know that it exists and that it's about 2.7.

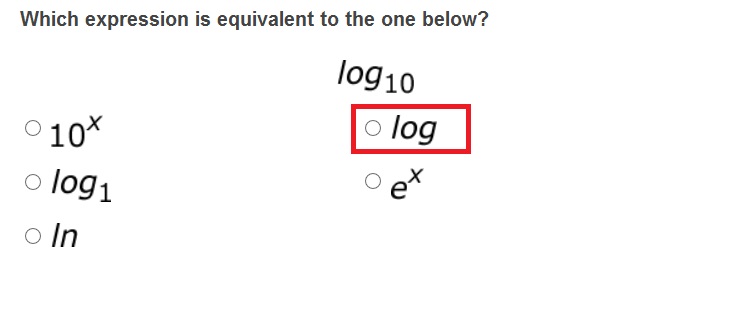
So, because these two logs are used so much, mathematicians came up with a shorthand way of writing them. Log10 became just plain log, and loge became the natural log, abbreviated ln. So if you see a problem that says log100, that really means log10(100), and if you see a problem that says ln5, that really just means loge(5).

Abbreviations for these two logs

Change of Base Formula

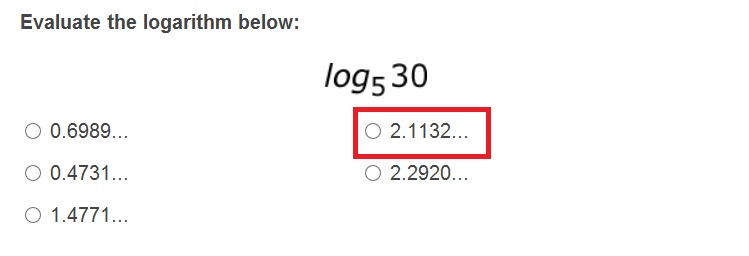
One last thing. Sometime we'll be asked to evaluate a log that doesn't have a whole number answer. Say log2(5). Well, since 2^2=4, and 2^3=8, and I'm being asked 2 to what is 5, I'm not really sure. It's somewhere in between 2 and 3, but it's probably closer to 2 - I don't know, maybe 2.1? Well, we don't really know, so we'd like to be able to use our calculator for this. But, most calculators only have log10 (log) and loge (ln) buttons on them, so how can we do log2?

For circumstances like this, we can use what's called the change of base formula. The change of base formula tells you that logb(a)=logz(a)/logz(b), which basically means we can change the log to a different base - we can pick what base to change it to and just divide the log of a with the log of b. So, we can evaluate log2(5) by changing the base from 2 to a button that's on my calculator - maybe 10. That allows us to say that log2(5) is equal to log10(5)/log10(2) - I can use the buttons on my calculator to find that it's actually about 2.3. That wasn't too far off!

Lesson Summary

To review: logarithmic form (logb(y)=x) and exponential form (b^x=y) are just two ways of writing the same thing. We can use that knowledge to evaluate logarithms by thinking, 'what is the exponent that would turn the base of the log into the number we're taking the log of?' If the log does not have a whole number solution, then we can use the change of base formula to estimate it with our calculator. The two log buttons that are on most calculators are the log10, which is just the log button with no number on it (log), and the loge, which is the button that just says ln.