Math 101: College Algebra

There are a few mistakes that are easy to make when multiplying binomials with FOIL and also a few ways to complicate problems like this, so why not make sure you're brushed up on your skills? You'll also learn a shortcut and how to use the area method to multiply even bigger polynomials.

Multiplying Binomials

Kind of like learning your times tables, multiplying binomials is one of those skills that can start out slow but with some practice become really fast and easy. This lesson is here to help you move from slow and unsure to fast and confident!

Because there are so many different ways to multiply two binomials, we'll do each problem in this lesson two ways. I won't explain both ways word-for-word in each problem, but we'll have both ways going on the screen at once so you can pick which of them you like more and also see how they're basically the same thing. Feel free to pause the lesson when I introduce a problem and give it a shot on your own before you watch me do it. That way you can either focus on the specific mistake you make or quickly skip through the problem if you got it right!

Example #1

Let's start with a pretty basic one.

Multiply (x + 6)(x - 6)

We'll use the FOIL method on the left side of the screen and the area method on the right. Remember that both are simply procedures that help you remember the four mini-multiplication problems that these examples will break down into. FOIL uses an acronym to help you remember which things to multiply, while the area method uses a chart to do the same thing. FOIL is a little bit quicker but also easier to make mistakes with, while the area method takes a little bit more time because you need to draw out the chart, but it's much better at preventing silly mistakes.

Multiply using the foil method.

Because the numbers aren't too bad on this one, I'll go ahead and use FOIL. That means I begin by multiplying the First terms, x and x, to get x^2.

Then we do the Outer terms, x and -6, to get -6x.

The Inner terms, 6 and x, give us 6x.

And finally we have the Last terms, 6 and -6, leaving us with a -36 on the end.

x^2 - 6x + 6x - 36

We're already basically done, but we'd like to simplify our answer by combining like terms. I've got two groups of xs here, and when we do -6x + 6x, they will actually cancel each other out, leaving our answer as simply: x^2 - 36

Now, there is actually a shortcut to answering problems like this where you are multiplying two conjugates together. Two binomials are conjugates if they have the same two terms but opposite signs on the second one like (a + b) and (a - b). Any time we multiply two conjugates together, the two middle terms will drop out, just like we saw, giving us the shortcut:

(a + b)(a - b) = a^2 - b^2

Example #2

Let's move on to another example:

Multiply (2x + 5)^2

I bring this example up for one important reason: it's good to learn that this problem should be done like the other ones in this video, even though it doesn't look like it. If you're not aware of this, it can be very easy to make this simple mistake:

2x^2 + 5^2

Distributing the exponent like this is not allowed! Here's the reason. Squaring something means multiplying it by itself. That means taking 2x + 5 and multiplying it by 2x + 5. Aha! Two binomials! If you make the mistake of distributing the exponent, you are only doing the first and last steps of FOIL and skipping the middle two - not good!

Multiply using the area method.

But now that we know how it should be done, let's do it the right way really quick. I'll use the area method for this one just to mix it up a little bit. Because we are multiplying two binomials, we draw our chart with two segments on each side, making four boxes on the inside. I now label each side with one of the binomials, each term getting its own segment of the side, like so.

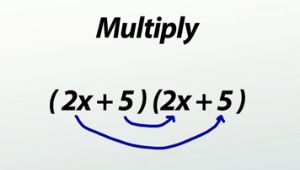	2x	5
2x
5

Now we just look above and across to decide what to multiply for each box. Doing so gives us this:

	2x	5
2x	4x^2	10x
5	10x	25

4x^2 + 10x + 10x + 25

and now we can combine like terms to end up with our answer:

4x^2 + 20x + 25

Example #3

Our last example will stray away from the title a little bit. You might call it an extra credit question - a preview into a future concept. The reason I do this is to show you that the skills we're mastering now can help you do more than just multiply binomials.

Take (x - 1)(x^2 + 5x + 3) for example. These aren't two binomials - this is a binomial times a trinomial. While the FOIL acronym no longer quite applies, it's still the same idea and the area model still works great.

Example of a binomial times a trinomial

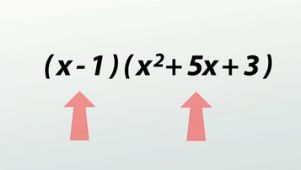This time, though, instead of making our chart have two segments on each side, we'll make a rectangular chart with two segments on one side and three on the other. The (x - 1) goes on the side with two segments, while the (x^2 + 5x + 3) goes on the side with three. And we're good to go!

	x^2	5x	3
x
-1

We simply look above and across to decide what to multiply for each box, fill the entire chart in, and combine like terms. Just like before!

	x^2	5x	3
x	x^3	5x^2	3x
-1	-x^2	-5x	-3

x^3 + 5x^2 - x^2 + 3x - 5x - 3 =

x^3 + 4x^2 - 2x - 3

Area Method and Other Polynomials

Need to multiply two trinomials? Make your chart three by three! A polynomial with five terms multiplied by one with six? A five by six chart! The area method will help you multiply any two polynomials together no matter what size they are. Hopefully you're starting now to feel like a polynomial pro.

Lesson Summary

Let's review. Conjugates are binomials that have the same terms, only the sign has been changed on the second one. When you multiply them together, you end up with the square of the first term minus the square of the second one: (a + b)(a - b) = a^2 - b^2.

When squaring a binomial, remember not to distribute the square to the two terms and instead rewrite another binomial next to the first one and FOIL it out: (a + b)^2 = (a + b)(a + b).

The area model can be used to multiply any two polynomials together, not just binomials. Simply make your chart have dimensions equal to the number of terms in each polynomial.