Math 101: College Algebra

Once you get familiar with any new operation, the next step in any algebra class is to learn how to solve equations with that operation in them. Absolute values are no different. Solve absolute value equations here!

Review of Absolute Values

Alright, so you're a pro at taking absolute values. |10| = ? 10! |-10| = ? Still 10! Negative numbers turn positive again because all an absolute value cares about is how 'large' the number is or what the magnitude is, not what sign it is. You even know that absolute values can be thought about as how far away that number is away from zero. But all that has just been to get you ready for this: solving absolute value equations.

Now this is a really common theme in algebra classes; simply learning a new skill isn't usually good enough and you're almost always going to end up having to learn how to apply that skill in order to solve an equation.

Solving Basic Equations with Absolute Values

X can be positive 15 or negative 15.

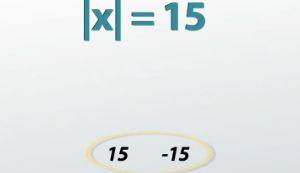So what are we talking about here? How about solve the |x| = 15. Well, any time we are asked to solve, it's our job to find a value for the variable that makes the equation true. So what number can I substitute in for x that will make the absolute value of it 15? Or you could think about this as what number is 15 units away from zero. 15 seems like the obvious choice, but we can't forget that -15 is also 15 units away from zero, which means that we actually get two answers here. x could be 15 or -15. This makes sense, right? If I just plug 15 into the absolute value, I just get 15 back out. Or if I plug -15 into the absolute value, I, again, just get 15 back out.

Absolute value equations give us two answers.

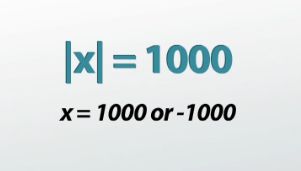This brings up a really important point: absolute value equations give us two answers. |x| = 20? x is 20 or -20. |x| = 1,000? x is 1,000 or -1,000. |x| = -5,000? x equals 5,000 or negative - wait! Absolute values can't be negative numbers, so no matter what I plug in for x, there's no way I'm going to get -5,000. So anytime an absolute value is set equal to a negative number, there is no solution.

Splitting an Equation into Two

Let's take the difficulty up a bit now. What if it asks you to solve |x-5| = 20? Now it can be really tempting to simply undo the subtraction with addition because they're inverse operations. So we add 5 to both sides and we get |x| = 25 and then we say, 'hey, two answers! 25 and -25 and we're done.' But you can't forget that absolute value bars kind of act like parentheses, which means you have to do everything on the inside before you take the absolute value. So when you first add 5 to both sides like that, you're kind of breaking that rule, which means doing that is a big no-no. You can't do that.

So if we take a step back and look at the big picture and say, 'okay, we're going to do some stuff to our x here, and after all that's done, we take the absolute value and get 20.' It's kind of like we can just cover up what's on the inside of the absolute value with our finger and say, 'I don't really care what's on the inside there; I take an absolute value when I get 20, which means that whatever is on the inside there must be equal to 20 or whatever is on the inside there must be equal to -20.'

When splitting an equation into two separate ones, do not switch the sign within the bars.

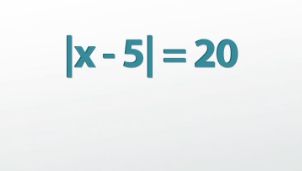After you split it up into two equations, then you can substitute back in what's on the inside of the bars by taking your finger away and saying, 'oh yeah, there's an x-5 there.' So x-5 = 20 or x-5 = -20, and those are the correct two equations we should end up with.

Now we can undo the subtraction with addition. Adding 5 to both sides gives us our two answers that x = 25 or x = -15. When you're solving equations, you can always check your answers by substituting them back into the original equation. I can do that here just to make sure I did everything okay. If I plug in 25, 25-5 is 20 and |20| stays the same and I get 20, so it checks out. I put in -15, -15-5 is -20. Now I take the absolute value, it turns positive again and it checks out as well so we know we got the right answer.

Just to remind us of another common misconception; when splitting up the equation into two separate ones, do not change it into x-5 = 20 or x+5 = 20. Again, absolute value bars do not change the operations going on inside of them, they only change the end result to be a positive number. So we still have to do the -5 and then after we do that, we change the number back. So changing it into two equations where one is a minus and one is a plus is another thing you've got to be careful not to do.

Lesson Summary

To review, absolute value equations give us two answers. Absolute values that equal negative numbers have no solution because absolute values are always positive. Absolute value equations with operations on the inside must be split up first before solving for the variable.