The capital Greek letter sigma might be most recognizable as a common symbol in a fraternity or sorority, but it's used for some pretty cool math tricks too. In this lesson, learn how to use sigma for things like determining how many seats are in Michigan Stadium.

Understanding a Number Series

So now that we know what a sequence is, we can actually start putting them to use. The most basic thing we can do with a sequence is to take all its entries, and add them up. When you do this, you turn something like 5, 6, 7, 8, which would be a sequence, into 5 + 6 + 7 + 8, which is now a series.

The most basic thing you can do with a sequence of numbers is to add them up.

So instead of simply being able to say things like, 'The 34th row in Michigan Stadium has 167 seats,' we can instead say things like, 'There are 3,434 seats in the first 34 rows of one section of Michigan Stadium.'

Calculating the number of stadium seats

Series Notation Using Sigma

I got that number simply by doing 35, because there were 35 seats in the first row, plus 39, because there are 4 more in each row, plus 43, plus 47, all the way out to plus 163, plus 167, because that's how many seats there would be in the 34th row. If you add all those numbers up, you get 3,434.

The thing is, I didn't sit down with my calculator and put in 35 + 39 + 43 all the way to 167, because that would've taken me a long time. Surprise, surprise, there's a shortcut. Now, we're going to learn a shortcut for how to actually add those numbers up in a later lesson. In this lesson, we're simply going to learn the way of writing that series out without having to use this '…' (35 + 39 + 43 + … + 167).

This symbol implies that we are adding up the terms of a sequence.

This is where the Greek letter sigma comes in. Anytime you see this letter in math, it's implying that we'll be taking a series, which means that we're just adding up the terms of a sequence.

What goes underneath the letter will tell you where we start the sequence. So, it tells you the starting term, n = something. The number on the top is where we end the series. The only thing missing is the rule, and that goes directly to the right of the sigma. What we have after we fill all those things in is either called sigma notation or summation notation.

Sigma tells us the starting term.

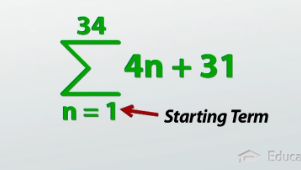Therefore, if I was going to express this series that tells us how many seats are in the first 34 rows of Michigan Stadium, I would draw my Greek letter sigma in. I would put n = 1 underneath is, because we're starting with the first row. I would put a 34 on top of it, because we're adding through row 34. And, I would put 4n + 31 next to the sigma. That's my rule because it goes up by 4 seats each time we add a row and if there was a 0 row, it would have 31 seats.

Building a Series Formula

So, let's quickly look at another random example. Let's say that we're asked to express the series 5 + 10 + 20 +40 + 80 + 160 + 320 + … using summation notation.

The first thing we should try to figure out is the rule for this series. I know that this is a geometric series because each term is multiplied by 2 to find the next one. 5 * 2 = 10, 10 * 2 = 20, and so forth.

I know that any geometric sequence has the rule a_n = a_1 * r^(n - 1). a_1 in this case is 5, because this is where my sequence begins. r is 2, because I'm multiplying by 2 each time. So, the rule for the nth term is 5(2)^(n - 1). And we can take this rule and slide it in directly to the right of this sigma.

Now, I simply need to label the beginning and ending value of the series. We start with the first term, so I put n = 1 on the bottom. Because there is a '...' at the end of our series here, that means it goes on forever. So, there's actually no ending point, so we write an infinity above the sigma to indicate that this is an infinite series.

Breaking Down a Series Formula

It's also good to be able to read something in sigma notation and understand what it's asking you to do. Say that you're asked to evaluate the following sum. I notice that underneath the sigma, I have n = 3, which means the first term in my series, the first term in the sequence that I'm going to be adding up, is the third term, a_3.

Breaking down a series formula

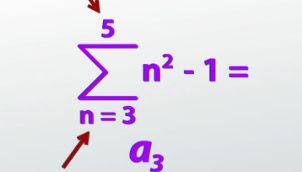The top is 5, which means I'm going to stop after I get to the fifth term. All I have to do is a_3 + a_4 + a_5.

I know that the rule is n^2 - 1. So, a_3 is just 3^2 - 1. a_4 is 4^2 - 1. a_5 is 5^2 - 1. I evaluate that out and 9 - 1 = 8, 4^2 - 1 = 15, 5^2 - 1 = 24. I add those numbers up: 8 + 15 + 24. And, it turns out that this sum would be equal to 47.

Lesson Summary

To review, a series is what you get when you add up the entries of a sequence. Summation notation, also known as sigma notation, is just a shorthand way of writing out a long list of terms that are being added together.

The rule for the sequence goes directly to the right of the letter sigma. The first term in the series to be added goes directly below the letter. The final term in the series is above it.