Math 101: College Algebra

Just because you now know what a linear equation is doesn't mean that you are a master! This video will help you learn how to apply your knowledge of linear equations in more abstract algebraic ways.

What is an Abstract Equation?

In this lesson, we will do some problems that have to do with linear equations that are a little more abstract. Now, when I say abstract, I mean that they probably won't have very many numbers in them and there usually won't be a specific procedure you can follow to end up with the result they're looking for. These problems are usually a little bit harder and they're also a little bit harder to teach, but I'm hoping that these four examples we go through will give you an idea of what I'm talking about.

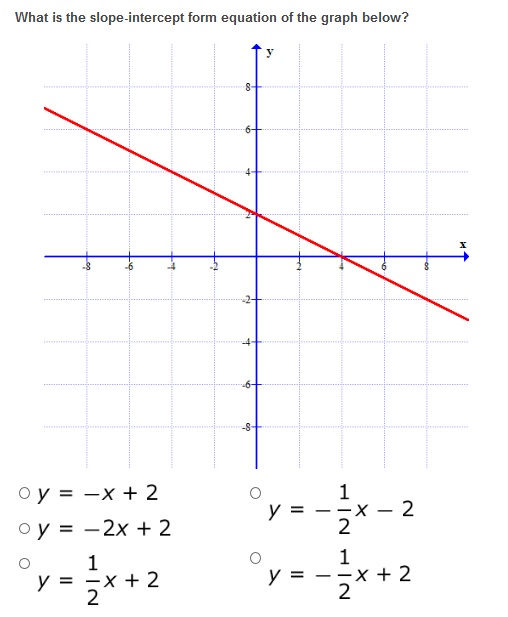
Finding the Equation of a Graph

We'll start with a question that shows you a graph and asks you to write the rule for that graph. Looking at the graph, we can tell that it's a linear equation because it's a straight line and we know that any linear equation can be written as y = mx+b, where the m is the slope and the b is the y-intercept. So it's essentially our job to figure out those two things from the graph; what is the slope and what is the y-intercept?

It will often times be easiest to find the y-intercept first, but there's no particular order you need to do these things in. We'll go ahead and do the y-intercept first. We know that the y-intercept is the point where the graph crosses the y-axis. It's also the beginning point. In this case, the graph crosses the y-axis right here, which have the coordinates (0,3) because it's where x is zero and y is 3. This means that we already know the y-intercept for this graph, and it's 3. So we already have half the equation done. The only other thing left to find is the slope.

Abstract linear equations have few numbers, and there will not always be a specific procedure to follow to get to the end result.

Now there's a lot of different words and phrases for the slope; often 'rise over run' is used or 'change in y over change in x', often times represented with the little Delta symbols. But all that's meaning is that the slope is how much the line goes up and down divided by how much the line goes left and right. So we can pick two points - any two points on this graph - and simply connect them with a straight up and down line and a straight left and right line and count to see how much it's going up and down and left and right.

So maybe we'll pick this point and the y-intercept. I draw a straight up and down line and a straight left and right line to get there and we simply count. I started up here at 5 and I went down 2, and then I started at -3 and went over 3 to the right. This means that my slope, or my rise over run, or my change in y and change in x - just the amount I went up and down, which was -2 over the amount I went left and right - was positive 3. I find that my slope is -2/3. This means the equation of my line is y = -2/3x+3.

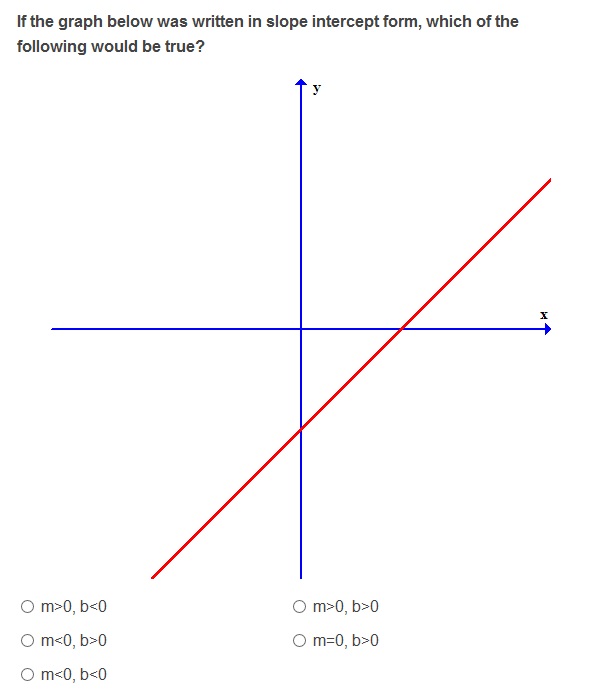
Identifying Graphs Based on Appearance

This next question is a really good example of a more abstract question. It simply tells us that if we have a line that's written in y = mx+b form, and my m value is greater than zero (therefore it's positive) and my b value is less than zero (which makes it negative), which one of these graphs must my line be?

So we're not going to plug in any numbers and simply do a bunch of operations and eventually end up with an answer (what I might call 'plug and chug'). This question simply requires you to have an understanding of what m and b are at a conceptual level for you to answer it.

Identifying graphs with the greatest slopes

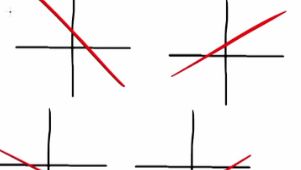So we need to know that m is the slope, and the slope is the rise over the run again, and all those different things we mentioned, which means that as it goes to the right, it also goes up. So positive slopes go up from left to right; if you're trying to walk up it from left to right, you'd have to walk up the line. So we know that because my slope is positive, it has to go up from left to right, so we can rule out these two examples because they are both going down. These two examples would have slopes that are negative, so I know it must be one of these two.

Which brings us to our second clue that b is negative. So we have to remember that b is our y-intercept. It's the beginning value. It's the point where the line crosses the y-axis. And if b is negative, that means it crosses the y-axis below the x-axis. It crosses it down at the bottom of the graph somewhere. This means that because this graph has a y-intercept above the x-axis, in the positive part of the y-axis, this can't be it, which brings us to our answer right here. It has a positive slope, it's going up from left to right and it begins on the negative part of the y-axis.

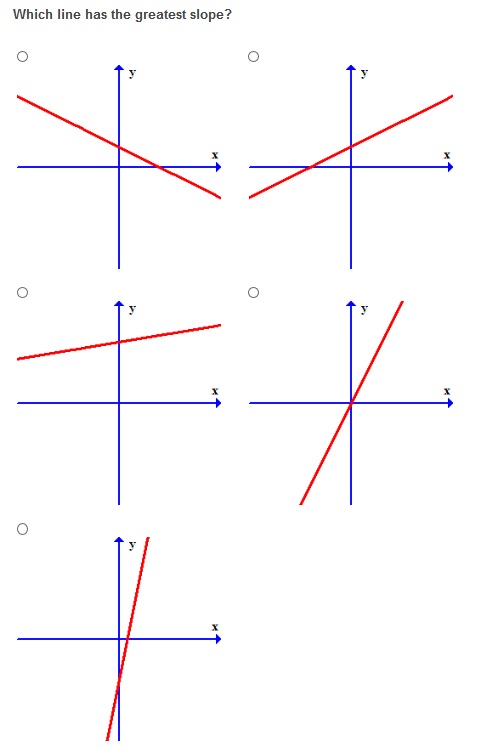
Finding the Greatest Slope

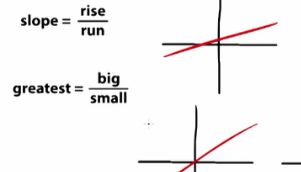
Our third example, again has no numbers, and simply asks us which of the four graphs shown has the greatest slope. Again, we simply need to know a conceptual understanding of what slope is - rise over run, change in y over change in x, how much it goes up and down over how much it goes left and right.

In order for my slope to be a big number, because it's a fraction, that means I have to have a big number on the top of the fraction and a small number on the bottom. This means it has to be going up and down a lot and only left and right a little bit. So we're looking for a line that goes up and down a lot and left and right a little bit.

I look through my options and I think, 'which line is going up and down the most?' And pretty quickly it stands out that this is our winner.

Another way of thinking about this is simply to have another representation of slope. Instead of using all these math terms and fractions, we can also think of slope as how steep a line is. The steeper the line, the greater the slope. So another way of asking this question is 'which line is the steepest?' In other words, which line, if you were trying to walk up it, would be the hardest to walk up? Which line would be the toughest mountain to climb? That makes it pretty clear that, again, this is our winner.

A slope is rise over run.

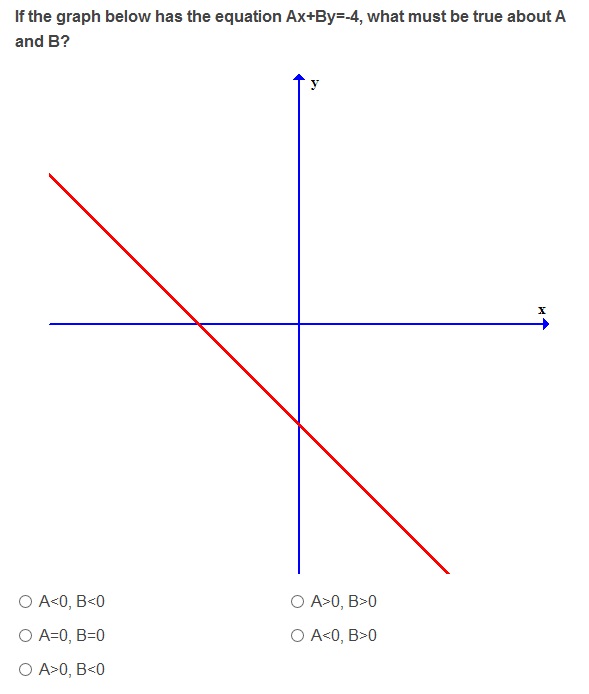
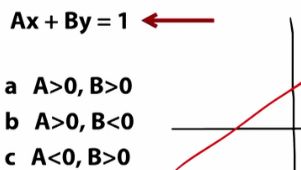
Solving Using Standard Form

This last problem shows us the graph and tells us that the standard form equation of this graph is Ax+By = 1. It then asks us which of the following must be true about A and B. So again, there aren't many numbers in this question, and it really requires you to have a fundamental understanding of what a standard form equation is and what A and B can really tell us.

What we're going to need to know for this problem has to do with the intercepts, specifically the y-intercept and the x-intercept. The thing about these intercepts that we really need to notice is that this y-intercept is positive and this x-intercept is negative, and we can use that fact to answer our question.

Now, we also need to know that at the y-intercept, my x value is zero, which means if I'm solving for the y-intercept, I can plug in or substitute zero for x and then undo the B in front of the y by dividing both sides by B, which will give me my y-intercept. This means that when I get my y-intercept by dividing positive 1 by B, I end up with a positive number, which means that 1/B has to be positive, which means that 1 and B have to be the same sign, both either positive or negative. Since 1 is positive, B has to be positive, which means that I know that my answer is not this, or this.

Example of a standard form equation

Again, to quickly review that (I said a lot of things pretty quickly), I know that my y-intercept is positive, which means that when I solve for the y-intercept by dividing by B, I have to get a positive number. In order for 1/B to be positive, they have to be the same sign, which means B has to be the same sign as 1; 1 is positive to B is positive.

I do the exact same thing for the x-intercept, except I know that I get a negative number. This time, I plug in zero for y, I divide both sides of the equation by A, and because I get a negative number, I know that 1 and A have to be opposite signs. This means that in this case, A is negative, so it's not either of these ones, which means my answer has to be C.

Lesson Summary

The only thing to say to review is that abstract algebraic examples can be tough. They often don't have very many numbers, more just variables and letters, and they require that you really have a fundamental, conceptual understanding of what's going on. There isn't just one procedure you can learn to solve them.

But hopefully these examples gave you a better idea of what I'm talking about and they'll help you do better on problems like this in the future.