Paired Difference Test
In statistics, a paired difference test is a type of location test used when comparing two sets of measurements to assess whether their population means differ. A paired difference test uses additional information about the sample that is not present in an ordinary unpaired testing situation, either to increase the statistical power or to reduce the effects of confounders.
Paired-Samples $t$ -Test
Paired samples
A typical example of the repeated measures t-test would be where subjects are tested prior to a treatment, say for high blood pressure, and the same subjects are tested again after treatment with a blood-pressure lowering medication . By comparing the same patient's numbers before and after treatment, we are effectively using each patient as their own control. That way the correct rejection of the null hypothesis (here: of no difference made by the treatment) can become much more likely, with statistical power increasing simply because the random between-patient variation has now been eliminated.
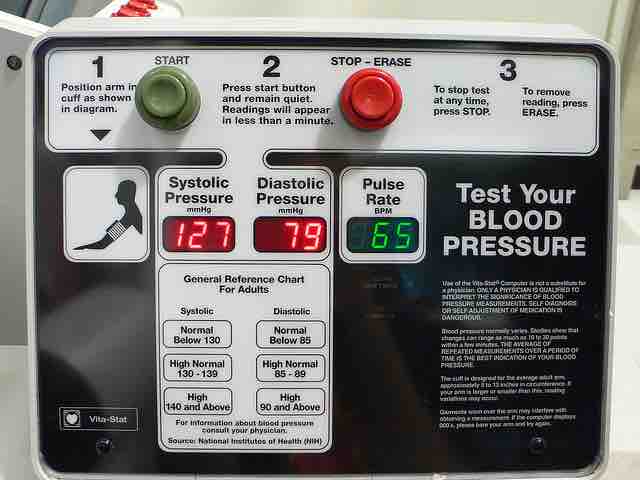
Blood Pressure Treatment
A typical example of a repeated measures
Note, however, that an increase of statistical power comes at a price: more tests are required, each subject having to be tested twice. Because half of the sample now depends on the other half, the paired version of Student's
A paired-samples
Paired-samples