If the mean (
The area above the
Standardization
Unfortunately, in most cases in which the normal distribution plays a role, the mean is not 0 and the standard deviation is not 1. Luckily, one can transform any normal distribution with a certain mean
Therefore, a
A key point is that calculating
Example
Assuming that the height of women in the US is normally distributed with a mean of 64 inches and a standard deviation of 2.5 inches, find the following:
- The probability that a randomly selected woman is taller than 70.4 inches (5 foot 10.4 inches).
- The probability that a randomly selected woman is between 60.3 and 65 inches tall.
Part one: Since the height of women follows a normal distribution but not a standard normal, we first need to standardize. Since
Therefore, the probability
The next step requires that we use what is known as the
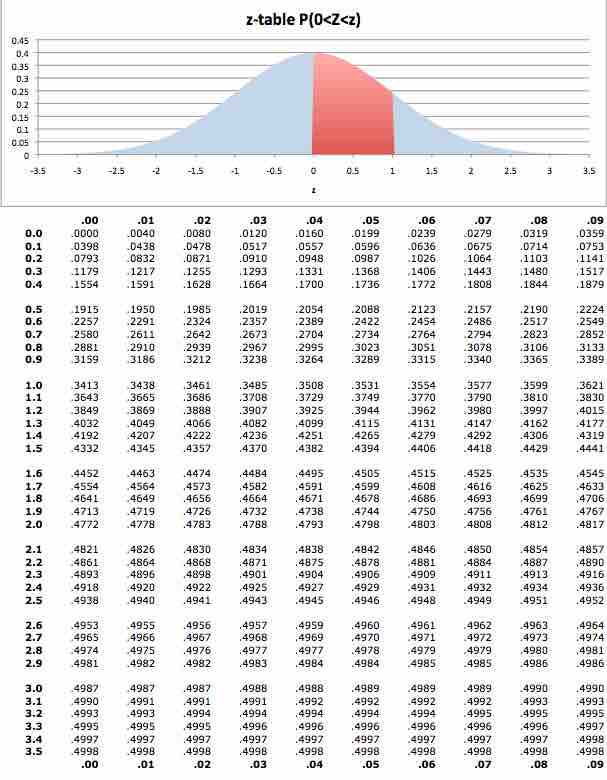
$z$ -table
The
From the table, we learn that:
Part two: For the second problem we have two values of
Notice that the first value is negative, which means that it is below the mean. Therefore: