Simply by using our intuition, we can begin to see the interrelatedness of rotational quantities like θ (angle of rotation), ω (angular velocity) and α (angular acceleration). For example, if a motorcycle wheel has a large angular acceleration for a fairly long time, it ends up spinning rapidly and rotating through many revolutions. The wheel's rotational motion is analogous to the fact that the motorcycle's large translational acceleration produces a large final velocity, and the distance traveled will also be large.
Kinematic Equations
Kinematics is the description of motion. We have already studied kinematic equations governing linear motion under constant acceleration:
Similarly, the kinematics of rotational motion describes the relationships among rotation angle, angular velocity, angular acceleration, and time. Let us start by finding an equation relating ω, α, and t. To determine this equation, we use the corresponding equation for linear motion:
As in linear kinematics where we assumed a is constant, here we assume that angular acceleration α is a constant, and can use the relation:
By using the relationships a=rα, v=rω, and x=rθ, we derive all the other kinematic equations for rotational motion under constant acceleration:
The equations given above can be used to solve any rotational or translational kinematics problem in which a and α are constant. shows the relationship between some of the quantities discussed in this atom.
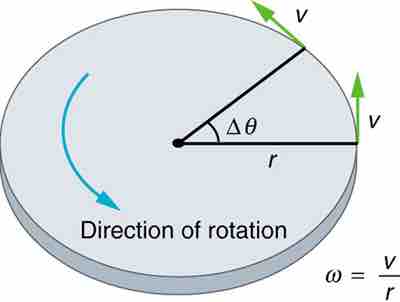
Linear and Angular
This figure shows uniform circular motion and some of its defined quantities.