In quantum mechanics, a wave function is a probability amplitude describing the quantum state of a particle and how it behaves. Typically, its values are complex numbers. For a single particle, it is a function of space and time. The most common symbols for a wave function are ψ(x) or Ψ(x) (lowercase or uppercase psi, respectively), when the wave function is given as a function of position x. Although ψ is a complex number, |ψ|2 is a real number and corresponds to the probability density of finding a particle in a given place at a given time, if the particle's position is measured.
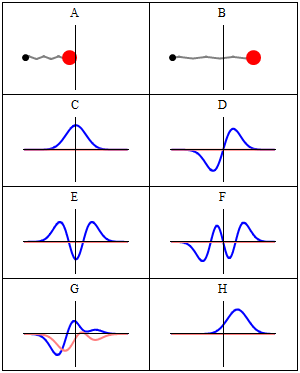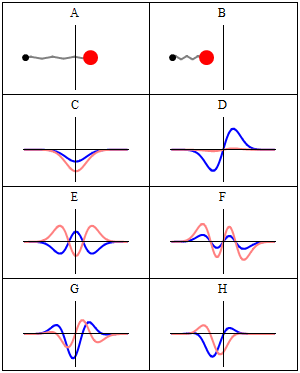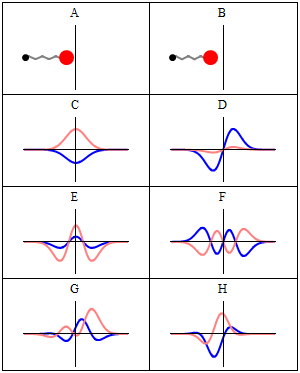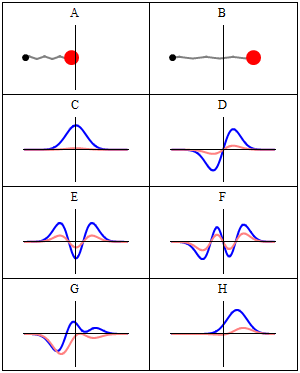
Trajectories of a Harmonic Oscillator
This figure shows some trajectories of a harmonic oscillator (a ball attached to a spring) in classical mechanics (A-B) and quantum mechanics (C-H). In quantum mechanics (C-H), the ball has a wave function, which is shown with its real part in blue and its imaginary part in red. The trajectories C-F are examples of standing waves, or "stationary states. " Each standing-wave frequency is proportional to a possible energy level of the oscillator. This "energy quantization" does not occur in classical physics, where the oscillator can have any energy.
The laws of quantum mechanics (the Schrödinger equation) describe how the wave function evolves over time. The wave function behaves qualitatively like other waves, such as water waves or waves on a string, because the Schrödinger equation is mathematically a type of wave equation. This explains the name "wave function" and gives rise to wave-particle duality.
The wave function must satisfy the following constraints for the calculations and physical interpretation to make sense:
- It must everywhere be finite.
- It must everywhere be a continuous function and continuously differentiable.
- It must everywhere satisfy the relevant normalization condition so that the particle (or system of particles) exists somewhere with 100-percent certainty.
If these requirements are not met, it's not possible to interpret the wave function as a probability amplitude. This is because the values of the wave function and its first order derivatives may not be finite and definite (having exactly one value), which means that the probabilities can be infinite and have multiple values at any one position and time, which is nonsense. Furthermore, when we use the wave function to calculate an observation of the quantum system without meeting these requirements, there will not be finite or definite values to use (in this case the observation can take a number of values and can be infinite). This is not a possible occurrence in a real-world experiment. Therefore, a wave function is meaningful only if these conditions are satisfied.