Energy is needed to generate a magnetic field both to work against the electric field that a changing magnetic field creates and to change the magnetization of any material within the magnetic field. For non-dispersive materials this same energy is released when the magnetic field is destroyed. Therefore, this energy can be modeled as being "stored" in the magnetic field .
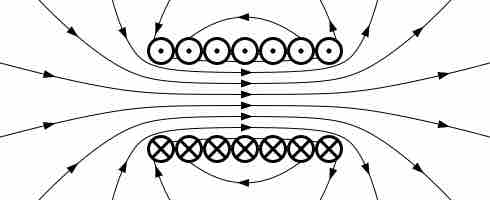
Magnetic Field Created By A Solenoid
Magnetic field created by a solenoid (cross-sectional view) described using field lines. Energy is "stored" in the magnetic field.
Energy Stored in a Magnetic Field
For linear, non-dispersive, materials (such that B = μH where μ, called the permeability, is frequency-independent), the energy density is:
Energy density is the amount of energy stored in a given system or region of space per unit volume. If there are no magnetic materials around, μ can be replaced by μ0. The above equation cannot be used for nonlinear materials, though; a more general expression (given below) must be used.
In general, the incremental amount of work per unit volume δW needed to cause a small change of magnetic field δB is:
Once the relationship between H and B is known this equation is used to determine the work needed to reach a given magnetic state. For hysteretic materials such as ferromagnets and superconductors, the work needed also depends on how the magnetic field is created. For linear non-dispersive materials, though, the general equation leads directly to the simpler energy density equation given above.
Energy Stored in the Field of a Solenoid
The energy stored by an inductor is equal to the amount of work required to establish the current through the inductor, and therefore the magnetic field. This is given by:
Proof: Power that should be supplied to an inductor with inductance L to run current I through it it given as
Therefore