Equipotential lines depict one-dimensional regions in which the electric potential created by one or more nearby charges has a constant value. This means that if a charge is at any point on a given equipotential line, no work will be required to move it from one point to another on that same line.
Equipotential lines may be straight, curved, or irregularly shaped, depending on the orientation of charges that give rise to them. Since they are located radially around a charged body, they are perpendicular to electric field lines, which extend radially from the center of a charged body.
A Single Point Charge
For a single, isolated point charge, the formula for potential (V) is functionally dependent upon charge (Q) and inversely dependent upon radial distance from the charge (r):
The radial dependence means that at any point a certain distance from the point charge, potential will be the same. Therefore, equipotential lines for a single point charge are circular, with the point charge at the center .
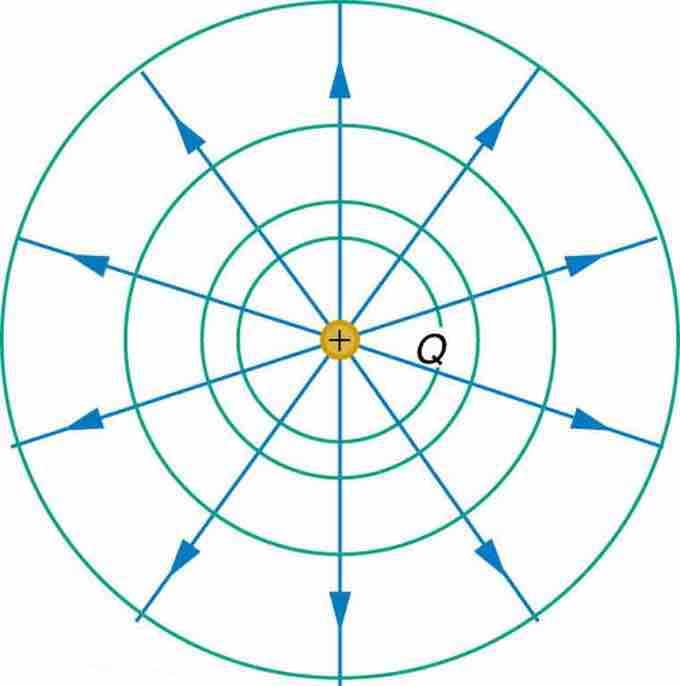
Equipotential Lines
An isolated point charge Q with its electric field lines (blue) and equipotential lines (green)
Multiple Point Charges
When multiple, discrete charges interact, their fields overlap. The combining of fields also results in a combining of potential, which results in the skewing of equipotential lines in areas that are close enough to both charges to "feel" the effects of both fields .
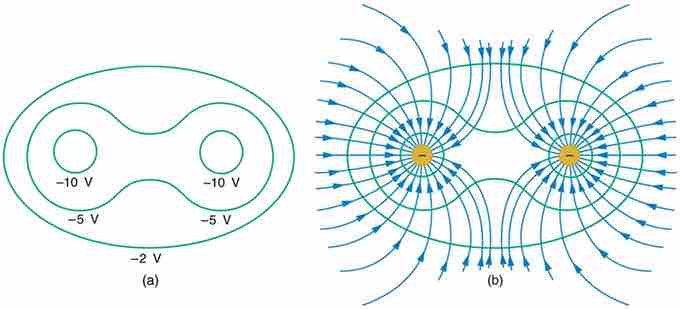
Equipotential lines with multiple charges
Equipotential lines become irregularly shaped when there are multiple charges vicinal to one another. At a point between the charges, a test charge may "feel" the effects of both charges.
Continuous Charge
If charges are distributed across two conductor plates in static equilibrium, in which charges are continuous and distributed in a straight line, the equipotential lines will be approximately straight . This is because the continuity of charges results in a continuity of action upon any point a certain distance from either plate.
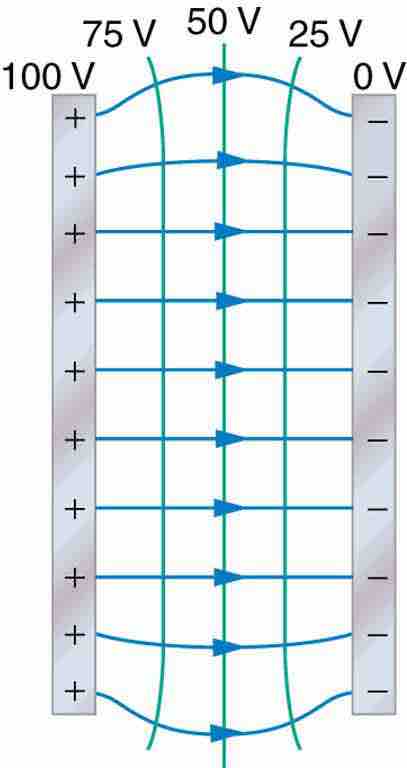
Equipotential Lines Between Two Plates
When charges are lined up and continuous on conducting plates, equipotential lines are straight between them. The only exception is a curving of the lines near the edges of the conductor plates.
This continuity is broken towards the ends of the plates, however, which causes curvature in these areas. This curvature is known as "edge effects. "