Calculating Values for Different Durations of Compounding Periods

Finding the Effective Annual Rate (EAR) accounts for compounding during the year, and is easily adjusted to different period durations.

Learning Objective

Calculate the present and future value of something that has different compounding periods

Key Points

The units of the period (e.g. one year) must be the same as the units in the interest rate (e.g. 7% per year).
When interest compounds more than once a year, the effective interest rate (EAR) is different from the nominal interest rate.
The equation in skips the step of solving for EAR, and is directly usable to find the present or future value of a sum.

Terms

Future Value
The value of an asset at a specific date. It measures the nominal future sum of money that a given sum of money is "worth" at a specified time in the future, assuming a certain interest rate, or more generally, rate of return, it is the present value multiplied by the accumulation function.
present value
Also known as present discounted value, is the value on a given date of a payment or series of payments made at other times. If the payments are in the future, they are discounted to reflect the time value of money and other factors such as investment risk. If they are in the past, their value is correspondingly enhanced to reflect that those payments have been (or could have been) earning interest in the intervening time. Present value calculations are widely used in business and economics to provide a means to compare cash flows at different times on a meaningful "like to like" basis.

Full Text

Sometimes, the units of the number of periods does not match the units in the interest rate. For example, the interest rate could be 12% compounded monthly, but one period is one year. Since the units have to be consistent to find the PV or FV, you could change one period to one month. But suppose you want to convert the interest rate into an annual rate. Since interest generally compounds, it is not as simple as multiplying 1% by 12 (1% compounded each month). This atom will discuss how to handle different compounding periods.

Effective Annual Rate

The effective annual rate (EAR) is a measurement of how much interest actually accrues per year if it compounds more than once per year. The EAR can be found through the formula in where i is the nominal interest rate and n is the number of times the interest compounds per year (for continuous compounding, see ). Once the EAR is solved, that becomes the interest rate that is used in any of the capitalization or discounting formulas.

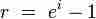
EAR with Continuous Compounding

The effective rate when interest compounds continuously.

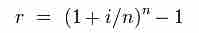
Calculating the effective annual rate

The effective annual rate for interest that compounds more than once per year.

For example, if there is 8% interest that compounds quarterly, you plug .08 in for i and 4 in for n. That calculates an EAR of .0824 or 8.24%. You can think of it as 2% interest accruing every quarter, but since the interest compounds, the amount of interest that actually accrues is slightly more than 8%. If you wanted to find the FV of a sum of money, you would have to use 8.24% not 8%.

Solving for Present and Future Values with Different Compounding Periods

Solving for the EAR and then using that number as the effective interest rate in present and future value (PV/FV) calculations is demonstrated here. Luckily, it's possible to incorporate compounding periods into the standard time-value of money formula. The equation in is the same as the formulas we have used before, except with different notation. In this equation, A(t) corresponds to FV, A₀ corresponds to Present Value, r is the nominal interest rate, n is the number of compounding periods per year, and t is the number of years.

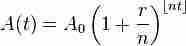
FV Periodic Compounding

Finding the FV (A(t)) given the PV (Ao), nominal interest rate (r), number of compounding periods per year (n), and number of years (t).

The equation follows the same logic as the standard formula. r/n is simply the nominal interest per compounding period, and nt represents the total number of compounding periods.

Solving for n

The last tricky part of using these formulas is figuring out how many periods there are. If PV, FV, and the interest rate are known, solving for the number of periods can be tricky because n is in the exponent. It makes solving for n manually messy. shows an easy way to solve for n. Remember that the units are important: the units on n must be consistent with the units of the interest rate (i).

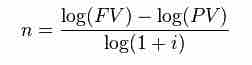
Solving for n

This formula allows you to figure out how many periods are needed to achieve a certain future value, given a present value and an interest rate.

[ edit ]

Prev Concept

Calculating Perpetuities

Comparing Interest Rates

Next Concept