To determine the optimal quantity of a public good, it is necessary to first determine the demand for it. Demand for public goods is represented through price-quantity schedules, which show the price someone is willing to pay for the extra unit of each possible quantity. Unlike the market demand curve for private goods, where individual demand curves are summed horizontally, individual demand curves for public goods are summed vertically to get the market demand curve. As a result, the market demand curve for public goods gives the price society is willing to pay for a given quantity. It is equal to the marginal benefit curve. Due to the law of diminishing marginal utility, the demand curve is downward sloping.
Often, the government supplies the public good. The supply curve for a public good is equal to its marginal cost curve. Because of the law of diminishing returns, the marginal cost increases as the quantity of the good produced increases. The supply curve therefore has an upward slope.
As already noted, the demand curve is equal to the marginal benefit curve, while the supply curve is equal to the marginal cost curve. The optimal quantity of the public good occurs where MB (society's marginal benefit) equals MC (provider's marginal cost), or where the two curves intersect . When MB = MC, resources have been allocated efficiently.
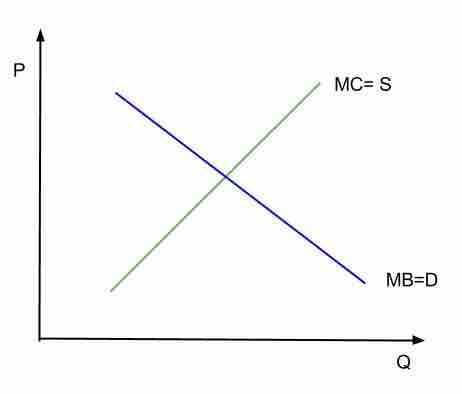
Optimal Quantity of a Public Good
The optimal quantity of public good occurs where MB = MC.
The public good provider uses cost-benefit analysis to decide whether to provide a particular good by comparing marginal costs and marginal benefits. Cost-benefit analysis can also help the provider decide the extent to which a project should be pursued. Output activity should be increased as long as the marginal benefit exceeds the marginal cost. An activity should not be pursued when the marginal benefit is less than the marginal cost. An activity should be stopped at the point where MB equals MC. This is the MC=MB rule, by which the provider of the public good can determine which plan, will give society maximum net benefit.