Derivatives of Exponential Functions

The importance of the exponential function in mathematics and the sciences stems mainly from properties of its derivative. In particular:

$\dfrac{d}{dx}e^{x} = e^{x}$

That is to say, $e^x$ is its own derivative. 

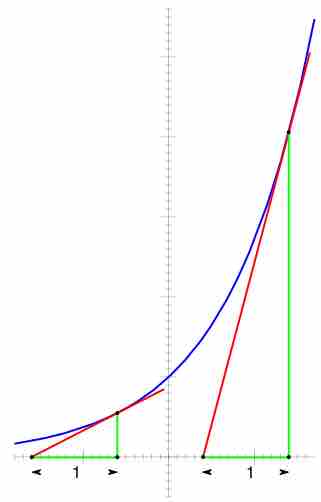
Graph of an Exponential Function

Graph of the exponential function illustrating that its derivative is equal to the value of the function. From any point $P$ on the curve (blue), let a tangent line (red), and a vertical line (green) with height $h$ be drawn, forming a right triangle with a base $b$ on the $x$-axis. Since the slope of the red tangent line (the derivative) at $P$ is equal to the ratio of the triangle's height to the triangle's base (rise over run), and the derivative is equal to the value of the function, $h$ must be equal to the ratio of $h$ to $b$. Therefore, the base $b$ must always be $1$.

Functions of the form $ce^x$ for constant $c$ are the only functions with this property. 

Other ways of saying this same thing include: 

• The slope of the graph at any point is the height of the function at that point.
• The rate of increase of the function at $x$ is equal to the value of the function at $x$.
• The function solves the differential equation $y' = y $.
• $e^x$ is a fixed point of derivative as a functional.

If a variable's growth or decay rate is proportional to its size—as is the case in unlimited population growth, continuously compounded interest, or radioactive decay—then the variable can be written as a constant times an exponential function of time. Explicitly for any real constant $k$, a function $f: R→R$ satisfies $f′ = kf $ if and only if $f(x) = ce^{kx}$ for some constant $c$.

Furthermore, for any differentiable function $f(x)$, we find, by the chain rule:

$\displaystyle{\frac{d}{dx}e^{f(x)} = f'(x)e^{f(x)}}$