A surface of revolution is a surface in Euclidean space created by rotating a curve around a straight line in its plane, known as the axis . Examples of surfaces generated by a straight line are cylindrical and conical surfaces when the line is co-planar with the axis, as well as hyperboloids of one sheet when the line is skew to the axis. A circle that is rotated about a diameter generates a sphere, and if the circle is rotated about a co-planar axis other than the diameter it generates a torus.
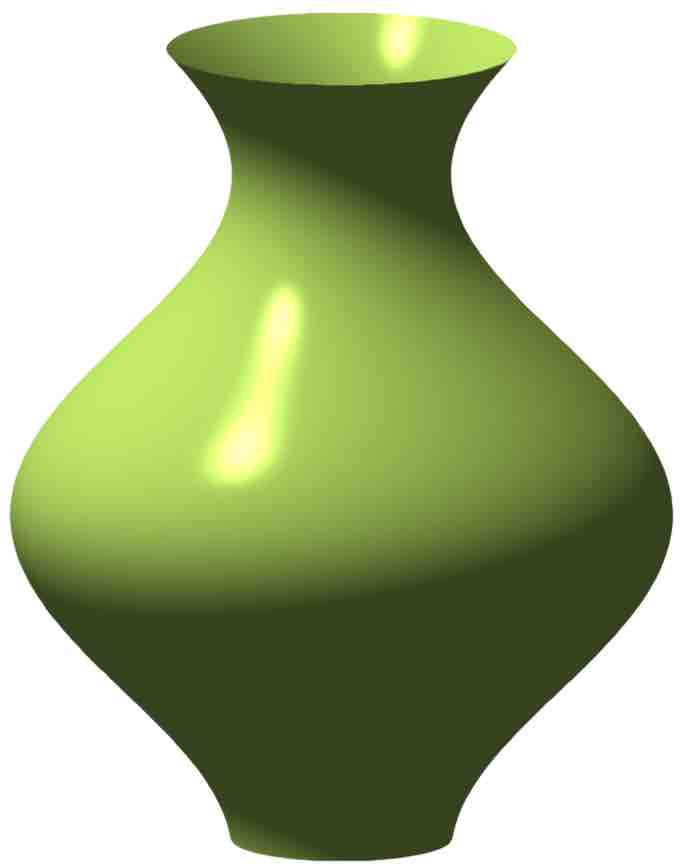
Surface of Revolution
A portion of the curve
If the curve is described by the parametric functions
provided that
If the curve is described by the function
for revolution around the
for revolution around the
Example
The spherical surface with a radius