Sequences

A sequence is an ordered list of objects (or events). Like a set, it contains members (also called elements, or terms). The number of ordered elements (possibly infinite) is called the length of the sequence. Unlike a set, order matters in a sequence, and exactly the same elements can appear multiple times at different positions in the sequence. Most precisely, a sequence can be defined as a function whose domain is a countable, totally ordered set, such as the natural numbers.

Examples: $(M, A, R, Y)$ is a different sequence from $(A, R, M, Y)$. Also, the sequence $(1, 1, 2, 3, 5, 8)$, which contains the number $1$ at two different positions, is a valid sequence. Sequences can be finite, as in this example, or infinite, such as the sequence of all even positive integers $(2, 4, 6, \cdots)$. Finite sequences are sometimes known as strings or words, and infinite sequences as streams. The empty sequence $( \quad )$ is included in most notions of sequence, but may be excluded depending on the context.

Indexing

The terms of a sequence are commonly denoted by a single variable, say $a_n$, where the index $n$ indicates the $n$th element of the sequence. Indexing notation is used to refer to a sequence in the abstract. It is also a natural notation for sequences whose elements are related to the index $n$ (the element's position) in a simple way. For instance, the sequence of the first 10 square numbers could be written as:

 $(a_1,a_2, \cdots ,a_{10}), \quad a_k = k^2$

This represents the sequence $(1,4,9, \cdots, 100)$.

Sequences can be indexed beginning and ending from any integer. The infinity symbol, $\infty$, is often used as the superscript to represent the sequence that includes all integer $k$-values starting with a certain one. The sequence of all positive squares is then denoted as:

$\displaystyle{(a_k)_{k=1}^\infty, \quad a_k = k^2}$.

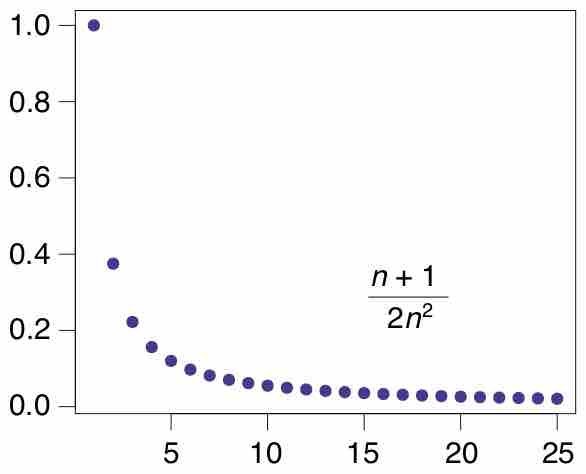
A Convergent Sequence

The plot of a convergent sequence ($a_n$) is shown in blue. Visually, we can see that the sequence is converging to the limit of $0$ as $n$ increases.

Specifying a Sequence by Recursion

Sequences whose elements are related to the previous elements in a straightforward way are often specified using recursion. This is in contrast to the specification of sequence elements in terms of their position. To specify a sequence by recursion requires a rule to construct each consecutive element in terms of the ones before it. In addition, enough initial elements must be specified so that new elements of the sequence can be specified by the rule.

Example

The Fibonacci sequence can be defined using a recursive rule along with two initial elements. The rule is that each element is the sum of the previous two elements, and the first two elements are $0$ and $1$: $a_n = a_{n-1} + a_{n-2}$ and $a_0 = 0, \, a_1=1$. The first ten terms of this sequence are ($0,1,1,2,3,5,8,13,21,34$).