Let
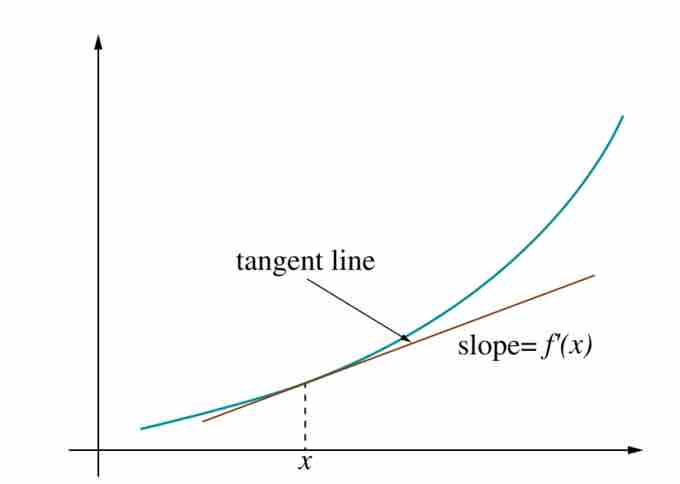
Derivative As Slope
The slope of tangent line shown represents the value of the derivative of the curved function at the point
Sometimes
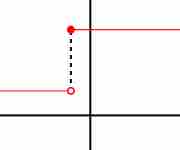
Discontinuous Function
At the point where the function makes a jump, the derivative of the function does not exist.
Using this idea, differentiation becomes a function of functions: The derivative is an operator whose domain is the set of all functions that have derivatives at every point of their domain and whose range is a set of functions. If we denote this operator by
For comparison, consider the doubling function
The operator
Because the output of
which is f(x)f(x). This output function can then be evaluated to get f(1)=2f(1) = 2,