There are several limits of special interest involving trigonometric functions.
1. $\displaystyle{\lim_{x \to 0} \frac{\sin x}{x} = 1}$
This limit can be proven with the squeeze theorem.
For
Dividing everything by
which reduces to:
Taking the limit of the right-hand side:
The squeeze theorem tells us that:
Equivalently:
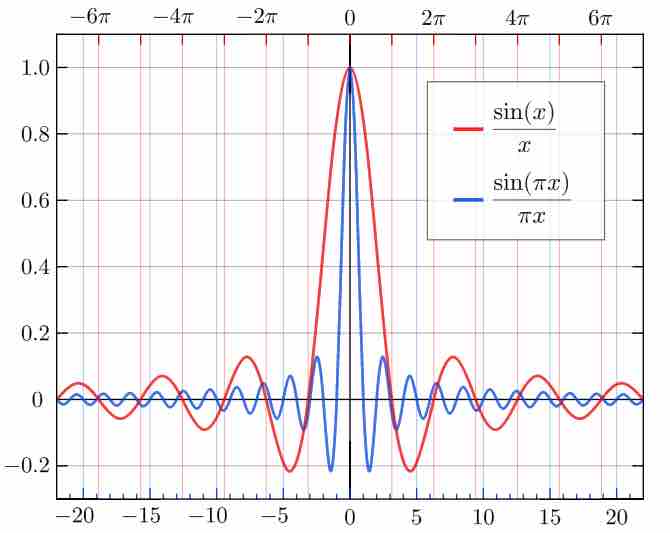
Sinc Function
The normalized sinc (blue, higher frequency) and unnormalized sinc function (red, lower frequency) shown on the same scale.
2. $\displaystyle{\lim_{x \to 0} \frac{1 - \cos x}{x} = 0}$
This equation can be proven with the first limit and the trigonometric identity
We start with:
Multiplying the numerator and denominator by
Using the algebraic limit theorem,
Therefore:
3. $\lim_{x \to \infty} x \sin \left(\frac{c}{x}\right) = c$
This relation can be proven by substituting