Limits involving infinity can be formally defined using a slight variation of the
Infinite Limit
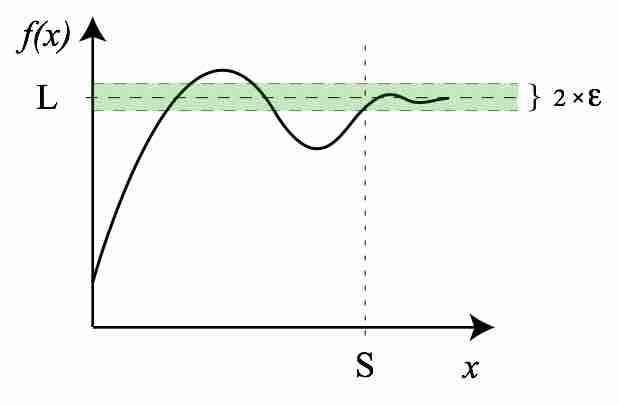
For any arbitrarily small
Similarly, the limit of
For a rational function
- If the degree of
$p$ is greater than the degree of$q$ , then the limit is positive or negative infinity depending on the signs of the leading coefficients; - If the degree of
$p$ and$q$ are equal, the limit is the leading coefficient of$p$ divided by the leading coefficient of$q$ ; - If the degree of
$p$ is less than the degree of$q$ , the limit is$0$ .
If the limit at infinity exists, it represents a horizontal asymptote at