The method of graphing a function to determine general properties can be used to solve financial problems.Given the algebraic equation for a quadratic function, one can calculate any point on the function, including critical values like minimum/maximum and x- and y-intercepts.

These calculations can be more tedious than is necessary, however. A graph contains all the above critical points and more, and acts as a clear and concise representation of a function. If one needs to determine several values on a quadratic function, glancing at a graph is quicker than calculating several points.

Example

Consider the function: 

$F(x)=-\frac {x^2}{10}+50x-750$

Suppose this models a profit function $f(x)$ in dollars that a company earns as a function of $x$ number of products of a given type that are sold, and is valid for values of $x$ greater than or equal to $0$ and less than or equal to $500$.

If a financier wanted to find the number of sales required to break even, the maximum possible loss (and the number of sales required for this loss), and the maximum profit (and the number of sales required for this profit), they could simply reference a graph instead of calculating it out algebraically.

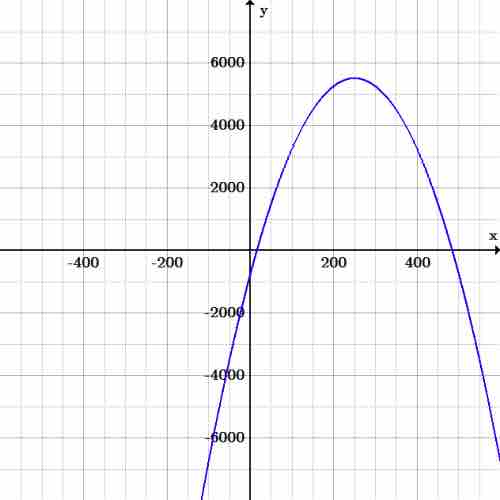
Financial example

Graph of the equation $F(x) = -\frac{x^2}{10} + 50x - 750$, where the x-axis is number of sales, and the y-axis is the monetary return

By inspection, we can find that the maximum loss is $750 (the y-intercept), which is lost at both $0$ and $500$ sales. Maximum profit is $5500 (the vertex), which is achieved at $250$ sales. The break-even points are between $15$ and $16$ sales, and between $484$ and $485$ sales.